looking for answers for part e(i) and e(ii)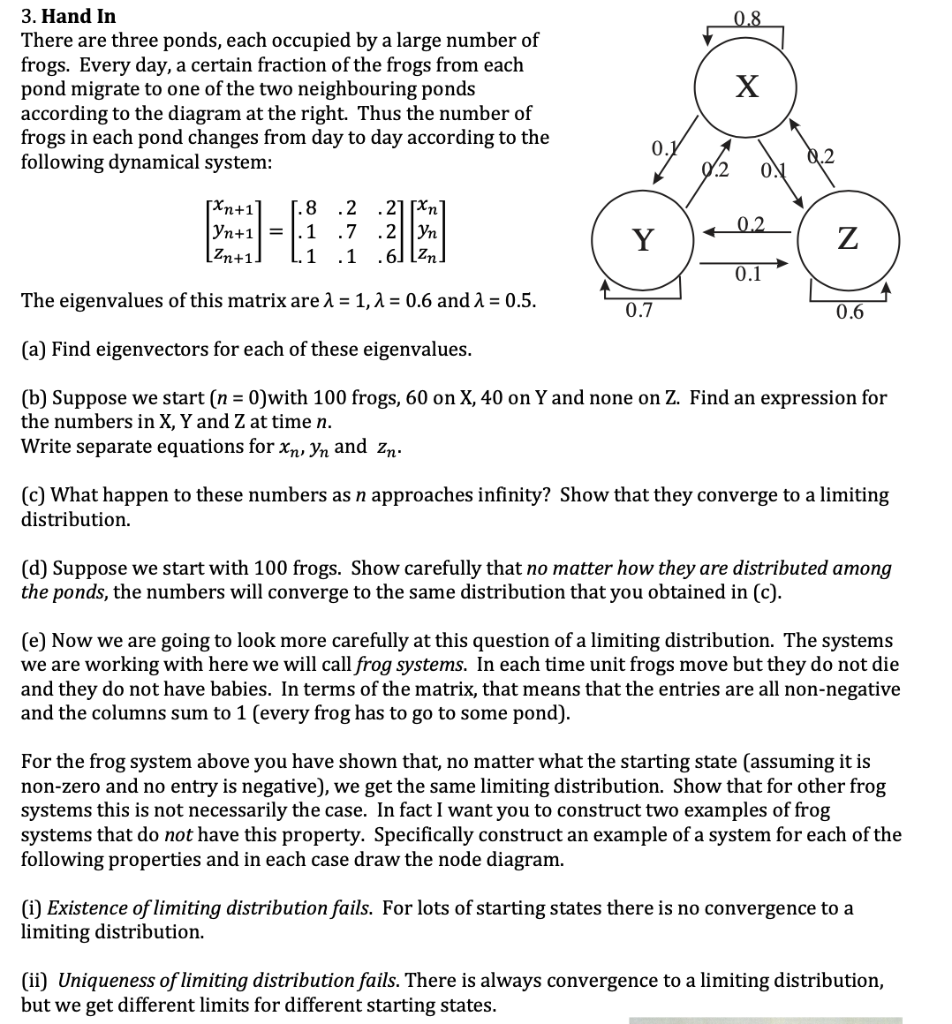

0.8 3. Hand In There are three ponds, each occupied by a large number of frogs. Every day, a certain fraction of the frogs from each pond migrate to one of the two neighbouring ponds according to the diagram at the right. Thus the number of frogs in each pond changes from day to day according to the following dynamical system: X 2.2 [Xn+1 Yn+1 = Zn+1 1.8 1 1 2 .2] [Xn .7.2|yn .1 .6[Zn] 0.2 Y Z 0.1 The eigenvalues of this matrix are 1 = 1, 1 = 0.6 and 1 = 0.5. 0.7 0.6 (a) Find eigenvectors for each of these eigenvalues. (b) Suppose we start (n = 0)with 100 frogs, 60 on X, 40 on Y and none on Z. Find an expression for the numbers in X, Y and Z at time n. Write separate equations for Xn, Yn and 2n- (c) What happen to these numbers as n approaches infinity? Show that they converge to a limiting distribution. (d) Suppose we start with 100 frogs. Show carefully that no matter how they are distributed among the ponds, the numbers will converge to the same distribution that you obtained in (c). (e) Now we are going to look more carefully at this question of a limiting distribution. The systems we are working with here we will call frog systems. In each time unit frogs move but they do not die and they do not have babies. In terms of the matrix, that means that the entries are all non-negative and the columns sum to 1 (every frog has to go to some pond). For the frog system above you have shown that, no matter what the starting state (assuming it is non-zero and no entry is negative), we get the same limiting distribution. Show that for other frog systems this is not necessarily the case. In fact I want you to construct two examples of frog systems that do not have this property. Specifically construct an example of a system for each of the following properties and in each case draw the node diagram. (i) Existence of limiting distribution fails. For lots of starting states there is no convergence to a limiting distribution. (ii) Uniqueness of limiting distribution fails. There is always convergence to a limiting distribution, but we get different limits for different starting states