Many likes guaranteed if the answer is correct and readable. Do not copy from previous answers PLEASE. We post here because the previous one is blatantly incorrect.

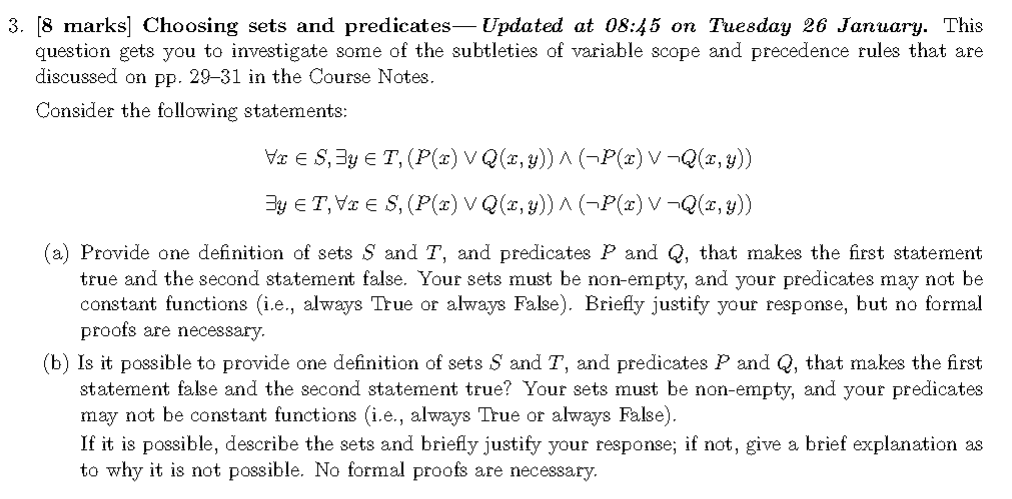
3. [8 marks] Choosing sets and predicates Updated at 08:45 on Tuesday 26 January. This question gets you to investigate some of the subtleties of variable scope and precedence rules that are discussed on pp. 2931 in the Course Notes. Consider the following statements: VE E S, BY E T,(P(2) VQ(x,y))^(-P(I) V-Q(x,y)) BY ET, VI E S, (P2) VQ(2,y))^(-P(2) V-Q(x, y)) (a) Provide one definition of sets S and T, and predicates P and Q, that makes the first statement true and the second statement false. Your sets must be non-empty, and your predicates may not be constant functions (i.e., always True or always False). Briefly justify your response, but no formal proofs are necessary. (b) Is it possible to provide one definition of sets S and T, and predicates P and Q, that makes the first statement false and the second statement true? Your sets must be non-empty, and your predicates may not be constant functions (i.e., always True or always False). If it is possible, describe the sets and briefly justify your response; if not, give a brief explanation as to why it is not possible. No formal proofs are necessary. 3. [8 marks] Choosing sets and predicates Updated at 08:45 on Tuesday 26 January. This question gets you to investigate some of the subtleties of variable scope and precedence rules that are discussed on pp. 2931 in the Course Notes. Consider the following statements: VE E S, BY E T,(P(2) VQ(x,y))^(-P(I) V-Q(x,y)) BY ET, VI E S, (P2) VQ(2,y))^(-P(2) V-Q(x, y)) (a) Provide one definition of sets S and T, and predicates P and Q, that makes the first statement true and the second statement false. Your sets must be non-empty, and your predicates may not be constant functions (i.e., always True or always False). Briefly justify your response, but no formal proofs are necessary. (b) Is it possible to provide one definition of sets S and T, and predicates P and Q, that makes the first statement false and the second statement true? Your sets must be non-empty, and your predicates may not be constant functions (i.e., always True or always False). If it is possible, describe the sets and briefly justify your response; if not, give a brief explanation as to why it is not possible. No formal proofs are necessary