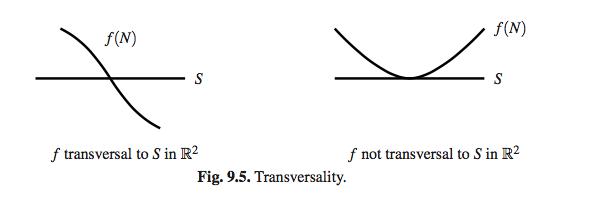
map f: NM is said to be transversal to a submanifold SCM (Figure 9.5) if for every Pf-(S), f(TN)+Tf(p)S=Tf(p)M. (9.4) (If A and B are subspaces of a vector space, their sum A+B is the subspace consisting of all a+b with a A and b B. The sum need not be a direct sum.) The goal of this exercise is to prove the transversality theorem: if a C map f: NM is transversal to a regular submanifold S of codimension k in M, then f-1 (S) is a regular submanifold of codimension k in N. When S consists of a single point c, transversality of f to S simply means that f-1 (c) is a regular level set. Thus the transversality theorem is a generalization of the regular level set theorem. It is especially useful in giving conditions under which the intersection of two submanifolds is a submanifold. Let p f(S) and (U,x1,...,x") be an adapted chart centered at f(p) for M relative to S such that UnS=Z(xm-k+1, ,x"), the zero set of the functions x-k+1 g: UR to be the map 8 = (xm-k+1 (a) Show that f(U) n(S) = (go f)(0). xm. Define (b) Show that f(U)nf-1 (S) is a regular level set of the function gof: f-1 (U) Rk. (c) Prove the transversality theorem. Activate Go to Setti f(N) * f transversal to S in R S Fig. 9.5. Transversality. f(N) S f not transversal to S in R