Math Analysis and Approaches

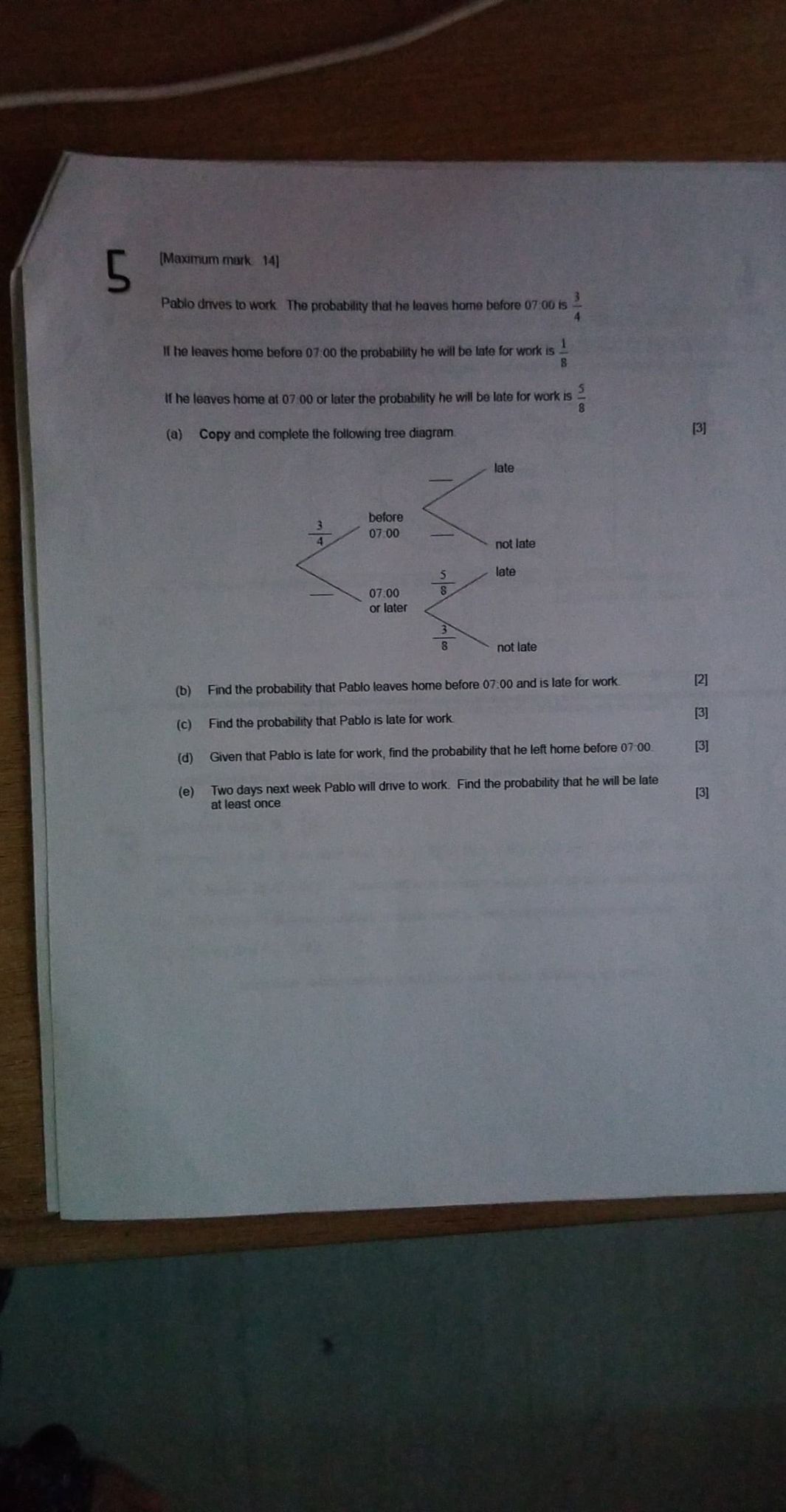
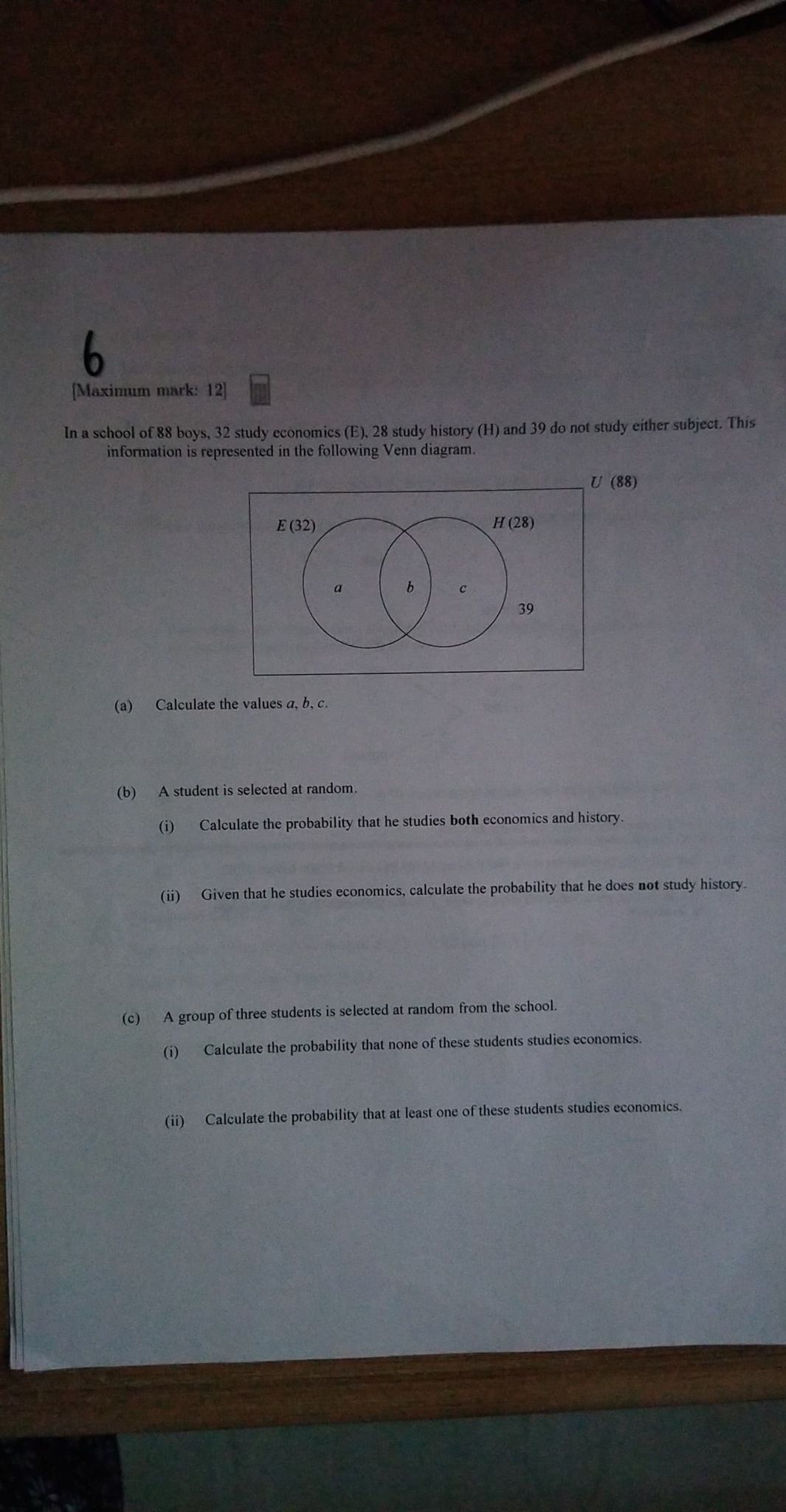
[Maximum mark: 6) In a class, 40 students take chemistry only, 30 take physics only, 20 take both chemistry and physics, and 60 take neither (a) Find the probability that a student takes physics given that the student takes chemistry. (b) Find the probability that a student takes physics given that the student does not take chemistry. (c) State whether the events "taking chemistry" and "taking physics" are mutually exclusive, independent, or neither. Justify your answer. [Maximum mark: 6] Two events A and B are such that P(A) = 0.25 and P(A U B) = 0.7. Find P(B), given that A and B are: (a) mutually exclusive; 121 (b) independent.[Maximum mark: 6) A lego storage box is left with 4 blue and 7 red bricks. Max takes a brick from the box at at random. random and attaches it to his new castle. He then chooses another brick from the same box (n) Complete the following tree diagram. 13 blue blue red blue red red (b) Calculate the probability that both bricks chosen by Max are of the same color. (3) [Maximum mark: 6] OO Two events A and B are such that P(A'n B) = P(An B') = 0.3 and P(An B) = 0.2. (a) Calculate P( A'n B'). 121 (b) Calculate P( B'). 2 (c) Hence find P(A' | B'). 12* * *BONUS* * * Maximum mark: 7 Isabella studies at the University of Chicago and has relatives in Detroit and New York City. Each time she visits her relatives, she either drives or flies. When travelling to Detroit, the probability she drives is -, and when travelling to New York City, the probability she flies is Given that the probability she drives when visiting her relatives is 5 12' . find the probability that for a particular trip, she: (a) travels to Detroit, giving your answer as a fraction; (b) is on her way to Detroit, given that she is flying. 3(Maximum mark: 6) The events A and B are independent such that P(B) = 3P(A) and P(AUB) = 0.68. Find P(B) [Maximum mark: 4] (a) On the Venn diagram below, shade in the region A' UB'. E Two events A and B are such that P(A' U B') = 0.85 and P(B') = 0.6. (b) Find P(A ( B)[Maximum mark 14] UT Pablo drives to work The probability that he leaves home before 07:00 is Il he leaves home before 07:00 the probability he will be late for work is _ If he leaves home at 07 00 or later the probability he will be late for work is (a) Copy and complete the following tree diagram. [3] late before 07 00 not late late 07:00 or later not late (b) Find the probability that Pablo leaves home before 07:00 and is late for work [2] (c) Find the probability that Pablo is late for work [3] (d) Given that Pablo is late for work, find the probability that he left home before 07:00 [3] (e) Two days next week Pablo will drive to work. Find the probability that he will be late at least once [3][Maximum mark: 12) In a school of 88 boys, 32 study economics (E), 28 study history (H) and 39 do not study either subject. This information is represented in the following Venn diagram. U (88) E (32) H (28) 39 (a) Calculate the values a, b, c. (b) A student is selected at random. (i) Calculate the probability that he studies both economics and history. (ii) Given that he studies economics, calculate the probability that he does not study history. (c) A group of three students is selected at random from the school. (i) Calculate the probability that none of these students studies economics. (ii) Calculate the probability that at least one of these students studies economics