Need help with part C of this question. Thanks

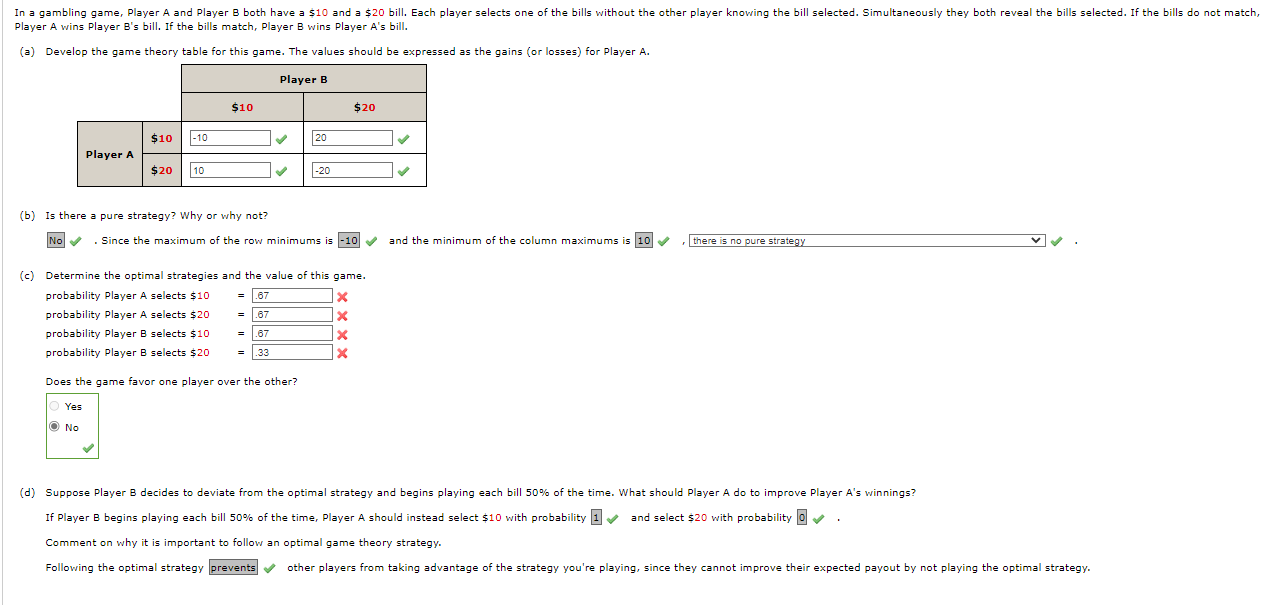
'layer A wins Player B's bill. If the bills match, Player B wins Player A's bill. (a) Develop the game theory table for this game. The values should be expressed as the gains (or losses) for Player A. (b) Is there a pure strategy? Why or why not? . Since the maximum of the row minimums is and the minimum of the column maximums is (c) Determine the optimal strategies and the value of this game. probabilityPlayerAselects$10probabilityPlayerAselects$20probabilityPlayerBselects$10probabilityPlayerBselects$20==== Does the game favor one player over the other? \begin{tabular}{|c|} \hline Yes \\ \hline No \\ \hline \end{tabular} If Player B begins playing each bill 50% of the time, Player A should instead select $10 with probability 1 and select $20 with probability 0 . Comment on why it is important to follow an optimal game theory strategy. Following the optimal strategy other players from taking advantage of the strategy you're playing, since they cannot improve their expected payout by not playing the optimal strategy. 'layer A wins Player B's bill. If the bills match, Player B wins Player A's bill. (a) Develop the game theory table for this game. The values should be expressed as the gains (or losses) for Player A. (b) Is there a pure strategy? Why or why not? . Since the maximum of the row minimums is and the minimum of the column maximums is (c) Determine the optimal strategies and the value of this game. probabilityPlayerAselects$10probabilityPlayerAselects$20probabilityPlayerBselects$10probabilityPlayerBselects$20==== Does the game favor one player over the other? \begin{tabular}{|c|} \hline Yes \\ \hline No \\ \hline \end{tabular} If Player B begins playing each bill 50% of the time, Player A should instead select $10 with probability 1 and select $20 with probability 0 . Comment on why it is important to follow an optimal game theory strategy. Following the optimal strategy other players from taking advantage of the strategy you're playing, since they cannot improve their expected payout by not playing the optimal strategy