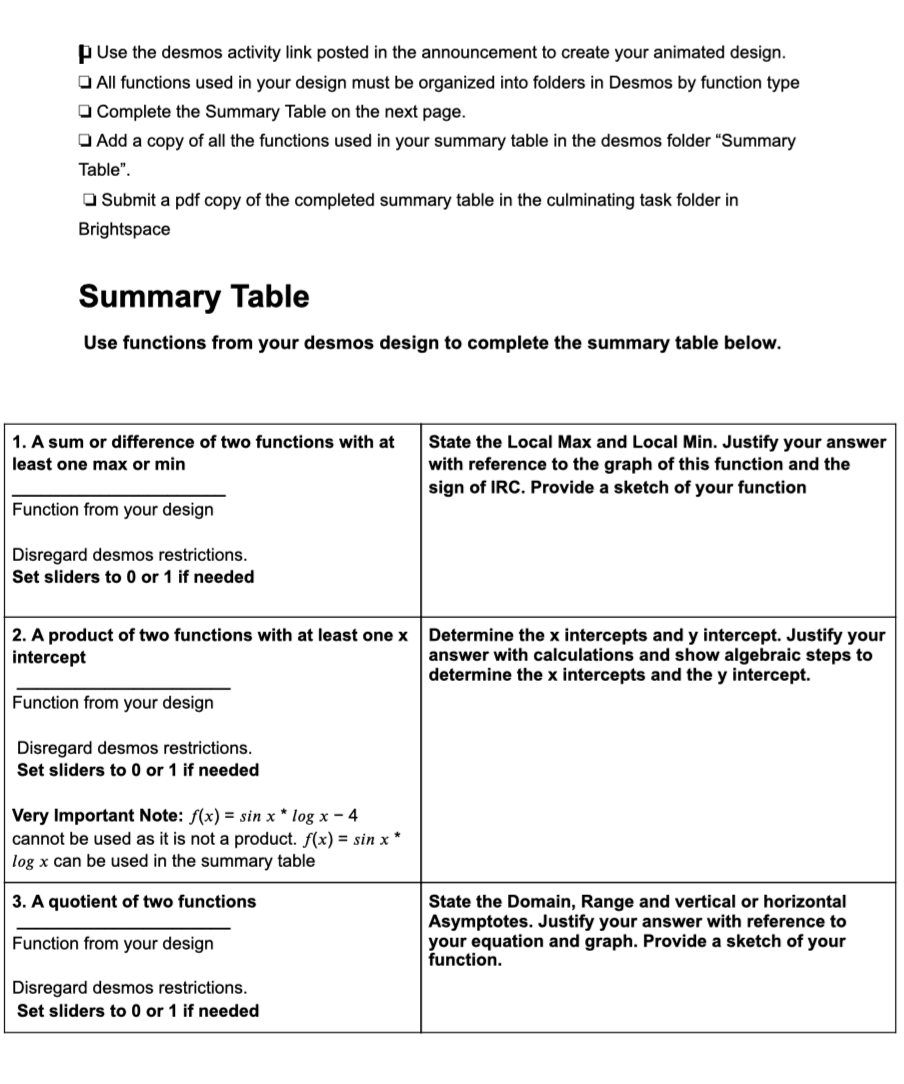
NO MARKS ARE GIVEN IF YOUR SOLUTION INCLUDES: e, In, differentiation or integration
In this assignment, you will use your knowledge of advanced functions and the graphing calculator desmos.com to create an animated design that Is inspired by your hobbies. You will also analyze some of the functions used and complete a summary table. The animated design must meet the following criteria: D It is unique and your own work El It must have a minimum of 10 functions CI It Includes at least one of each of the functions below: A. Polynomial Function(degree 3 or higher) Exponential Function . Logarithmic Function . Trigonometric Function Rational Function A sum or difference function with at least one local maximum or minimum. The two functions added must be from two different categories A. B, C. D. E (eg. a trig a. a rational) G. A product function with at least one x intercept. The two functions multiplied must be from two different categories A. B. c. D. E (eg. a trig s a rational) H. A quotient function. The two functions that are divided must be from two different categories A, 3.6, D. E (99. a trig & a rational) I. A composite function. The inner and outer function must be from two different categories from the categories above A. B, C. D. E (eg. trig 8: a rational) J. No other functions allowed. This means no linear, quadratic. absolute value functions allowed or functions that were not covered In this course. You must not use functlons that simplify linear or quadratic functions. :nrnoorn Your task Is: D Watch the vidaee posted for support with your design and using deernoa.com (30 whites] - - - Creating a Design using Deemoe . : a an. - Mil-hating a design using sliders - '. . - Shading the design using Inequalities l 'u. - Sliders In restrictions p Use the desrnos activity link posted In the announcement to create your animated design. :I All functions used in your design must be organized into folders in Desmos by function type It Complete the Summary Table on the next page. D Add a copy of all the functions used in your summary table in the desmos folder \"Summary Table". El Submit a pdf copy ofthe completed summary table In the culminating task folder In Brightspace Summary Table Use functions from your desmos design to complete the summary table below. 1. A sum or difference of two functions with at State the Local Max and Local Min. Justify your answer least one max or min with reference to the graph of this function and the sign of me. Provide a sketch of your function Function from your design Disregard dosrnos restrictions. Set sliders to ti or 1 If needed 2. A product of two functions with at least one 1: Determine the x Intercepts and y Intercept Justify your "neg-rum answer with calculations and show algebraic steps to determlne the x intercepts and the y Intercept. Function from your design Disregard desmos restrictions. Set sliders to 0 or 1 if needed Very Important Note: x) = sir: x * log at - 4 cannot be used as it is not a product. x) = sin in: ' log 2: can be used in the summary table 3. A quotient of two functions State the Domain. Range and vertical or horlzontal Asymptotes. Justify your answer with reference to Function from your design your equation and graph. Provide a sketch of your functlon. Disregard desmos restrictions. Set sliders to I] or 1 if needed