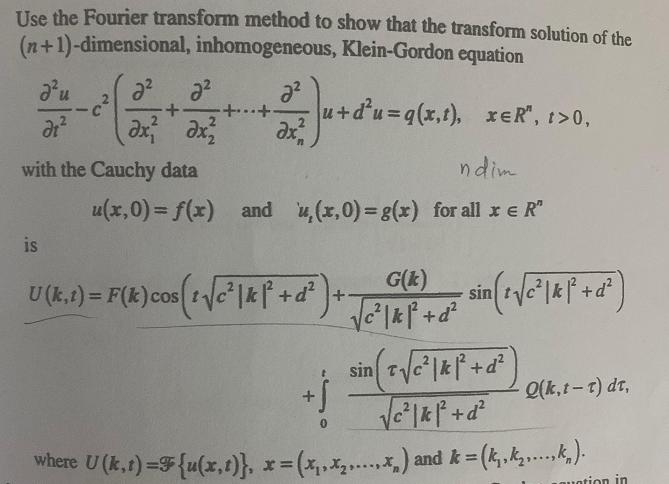
Use the Fourier transform method to show that the transform solution of the (n+1)-dimensional, inhomogeneous, Klein-Gordon equation u+d'u=q(x,t), reR", 1>0, ax with the Cauchy data ndim u(x,0) = f(x) and ,(x,0)= g(x) for all r e R" is U(k.1) = F(k)cos tye"|& f+d + G(k) %3D Q(k, t-t) dr, where U (k,t) =F{u(x,t)}, *3= (x,,x.) and k = %3D uotion in