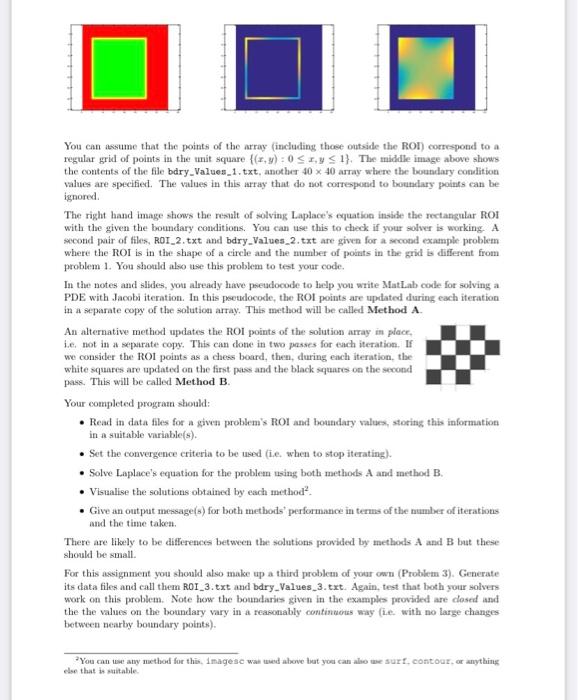
Objective To gain practical experience in writing code that computes a numerical solution of a PDE. Introduction In two dimensions, Laplace's Equation can be written or + y = 0. for (x,y) where is the Region of Interest (ROI) inside which we want to solve the equation. In short form it can be written Au = 0 or = 0. Laplace's Equation needs a boundary condition to be well-posed. A Dirichlet boundary condition is sufficient, i.e, one that specifies the value of u at all points on 22. In other the words, the value of ur,y) needs to be given for all points (x,y) that are on the boundary of the ROI 1. For this coursework task, you need to write code that uses a numerical scheme to estimate the solution for Laplace's Equation for a ROI in a discrete grid of points. You will be given files that contain the following information Which grid points are on the boundary of the ROI and which are inside. The values of the function at the boundary points. In other words, the files describe the ROI and the boundary condition. Your code should read these files and then use iterative Jacobi methods to estimate the solution of Laplace's Equation inside the ROI Instructions You will be given two examples of problems to solve (i.e. the ROI information and boundary values for each). You should check that your code works with each one. When you are satisfied that your code is working, you will make up your own ROI and boundary values and test that your code still works. The data files for the example problems can be downloaded from KEATS. These include ROI_1.txt and bdry Values 1.txt for the first example problem. Each file contains an array of data. The left hand image below illustrates the contents of the file ROI_1.txt which describes a rectangular ROL. This is in the form of a 40 x 40 array with values of +1 for points inside the ROI (green in the diagram), 0 for points on the boundary (yellow), and -1 for points outside the boundary (red) The arrays are displayed wing the images You can assume that the points of the array (including those outside the ROI) correspond to a regular grid of points in the unit square {x,y): 0 SL epsilon return U Objective To gain practical experience in writing code that computes a numerical solution of a PDE. Introduction In two dimensions, Laplace's Equation can be written or + y = 0. for (x,y) where is the Region of Interest (ROI) inside which we want to solve the equation. In short form it can be written Au = 0 or = 0. Laplace's Equation needs a boundary condition to be well-posed. A Dirichlet boundary condition is sufficient, i.e, one that specifies the value of u at all points on 22. In other the words, the value of ur,y) needs to be given for all points (x,y) that are on the boundary of the ROI 1. For this coursework task, you need to write code that uses a numerical scheme to estimate the solution for Laplace's Equation for a ROI in a discrete grid of points. You will be given files that contain the following information Which grid points are on the boundary of the ROI and which are inside. The values of the function at the boundary points. In other words, the files describe the ROI and the boundary condition. Your code should read these files and then use iterative Jacobi methods to estimate the solution of Laplace's Equation inside the ROI Instructions You will be given two examples of problems to solve (i.e. the ROI information and boundary values for each). You should check that your code works with each one. When you are satisfied that your code is working, you will make up your own ROI and boundary values and test that your code still works. The data files for the example problems can be downloaded from KEATS. These include ROI_1.txt and bdry Values 1.txt for the first example problem. Each file contains an array of data. The left hand image below illustrates the contents of the file ROI_1.txt which describes a rectangular ROL. This is in the form of a 40 x 40 array with values of +1 for points inside the ROI (green in the diagram), 0 for points on the boundary (yellow), and -1 for points outside the boundary (red) The arrays are displayed wing the images You can assume that the points of the array (including those outside the ROI) correspond to a regular grid of points in the unit square {x,y): 0 SL epsilon return U