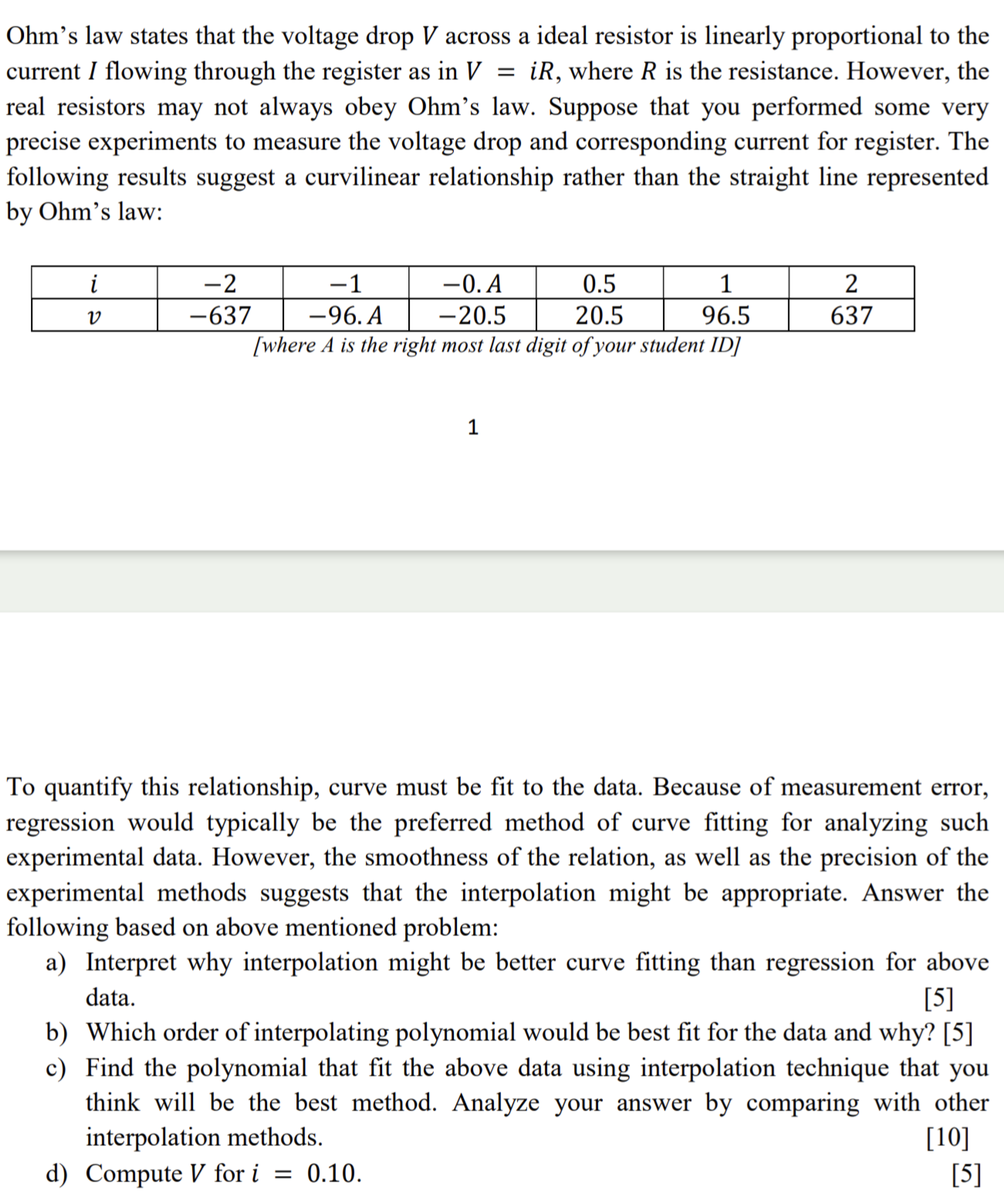
Ohm's law states that the voltage drop $V$ across a ideal resistor is linearly proportional to the

current I flowing through the register as in $V=iR,$ where $R$ is the resistance. However, the

real resistors may not always obey Ohm's law. Suppose that you performed some very

precise experiments to measure the voltage drop and corresponding current for register. The

following results suggest a curvilinear relationship rather than the straight line represented

by Ohm's law:

$[$where A is the right most last digit of your student ID$]$

To quantify this relationship, curve must be fit to the data. Because of measurement error,

regression would typically be the preferred method of curve fitting for analyzing such

experimental data. However, the smoothness of the relation, as well as the precision of the

experimental methods suggests that the interpolation might be appropriate. Answer the

following based on above mentioned problem:

a$)$ Interpret why interpolation might be better curve fitting than regression for above

data.

b$)$ Which order of interpolating polynomial would be best fit for the data and why? $[5]$

c$)$ Find the polynomial that fit the above data using interpolation technique that you

think will be the best method. Analyze your answer by comparing with other

interpolation methods.

d$)$ Compute $V$ for $i=0\mathrm{.}10.$ Ohm's law states that the voltage drop V across an ideal resistor is linearly proportional to the current i flowing through the resister as in V$=$i R$,$ where R is the resistance. However, real resistors may not always obey Ohm's law. Suppose that you performed some very precise experiments to measure the voltage drop and corresponding current for a resistor. The following results suggest a curvilinear relationship rather than the straight line represented by Ohm's law: To quantify this relationship, a curve must be fit to the data. Because of measurement error, regression would typically be the preferred method of curve fitting for analyzing such experimental data. However, the smoothness of the relationship, as well as the precision of the experimental methods, suggests that interpolation might be appropriate. Use a fifth$-$order interpolating polynomial to fit the given data. $($Round the final answer to two decimal places.$)$ The interpolating polynomial V$=$