ONLY CORRECT ANSWERS please
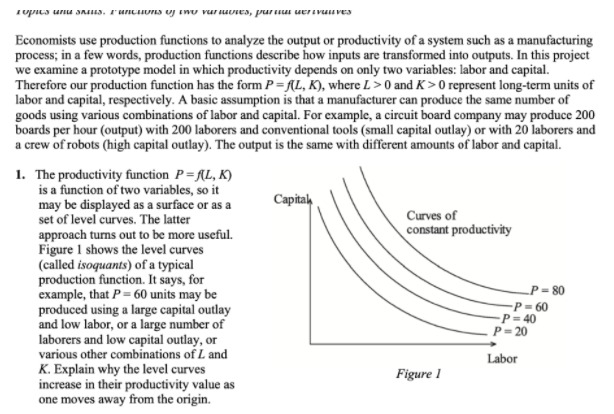
Consider the 5olow-5wan model with no population growth and no technological progress. bet aggregate consumption be denoted by C; and aggregate output be denoted by. Over time. aggregate investment in physical capital is nanced by savings where total savings at time t is equal to the savings rate. .5, times aggregate output. Aggregate output is produced through use of physical capital. Kt- and labour. Nt- The aggregate production function, which exhibits constant returns to scale and diminishing marginal products with respect to individual inputs. is given by Y; = PHI}, M) with Ni = N. Let Ct denote consumpon-per-capita. kt denote capital-per- capita. and yt = fg) denote output-per-capita. Assume that a steady state exists in per-capita variables with the steady state capital stock following a function 1:13} with the property that dial 3:. I]. In words, assume that the steady state capital stock is higher when the savings rate is higher. 4i Construct the expression relating consumption-per-capita to output-per-capita land implicitly to the capital-per-capital. By taking the derivative of this expression with respect to the savings rate. show that. in the steady state. increasing the savings rate has two opposing forces on steady state consumption-per-capita. [25 marks} With reference to the opposing forces that you found in your answer for Question #4, explain when and why undersaving or oversaving occurs in the steady state. 2. Explain why the level curves of a realistic production function have negative slope. (Consider the meaning of a level curve that has positive slope). 3. The level curves of the production function indicate how much a decrease in labor must be replaced by an increase in capital to maintain the same output. Suppose a company operates on a level curve P = ((L, K) = c at certain values of (L, K). Use differentials to show that if L and K are changed by small amounts AL and AK, respectively, the corresponding change in P is AP = fLAL + fx AK, where fi and fx are the partial derivatives of f evaluated at (L, X). 4. Now suppose the company wishes to change _ and A and maintain the same output (that is, remain on the AK same level curve). Setting AP =0 in Step 3, show that the changes in L and K must satisfy ALEconomists use production functions to analyze the output or productivity of a system such as a manufacturing process; in a few words, production functions describe how inputs are transformed into outputs. In this project we examine a prototype model in which productivity depends on only two variables: labor and capital. Therefore our production function has the form P=AL, K), where [ > 0 and K > 0 represent long-term units of labor and capital, respectively. A basic assumption is that a manufacturer can produce the same number of goods using various combinations of labor and capital. For example, a circuit board company may produce 200 boards per hour (output) with 200 laborers and conventional tools (small capital outlay) or with 20 laborers and a crew of robots (high capital outlay). The output is the same with different amounts of labor and capital. 1. The productivity function P=AL, K) is a function of two variables, so it may be displayed as a surface or as a Capital set of level curves. The latter Curves of approach turns out to be more useful. constant productivity Figure I shows the level curves (called isoquants) of a typical production function. It says, for example, that P = 60 units may be P = 80 produced using a large capital outlay P = 60 and low labor, or a large number of - P = 40 P = 20 laborers and low capital outlay, or various other combinations of L and Labor K. Explain why the level curves Figure I increase in their productivity value as one moves away from the origin