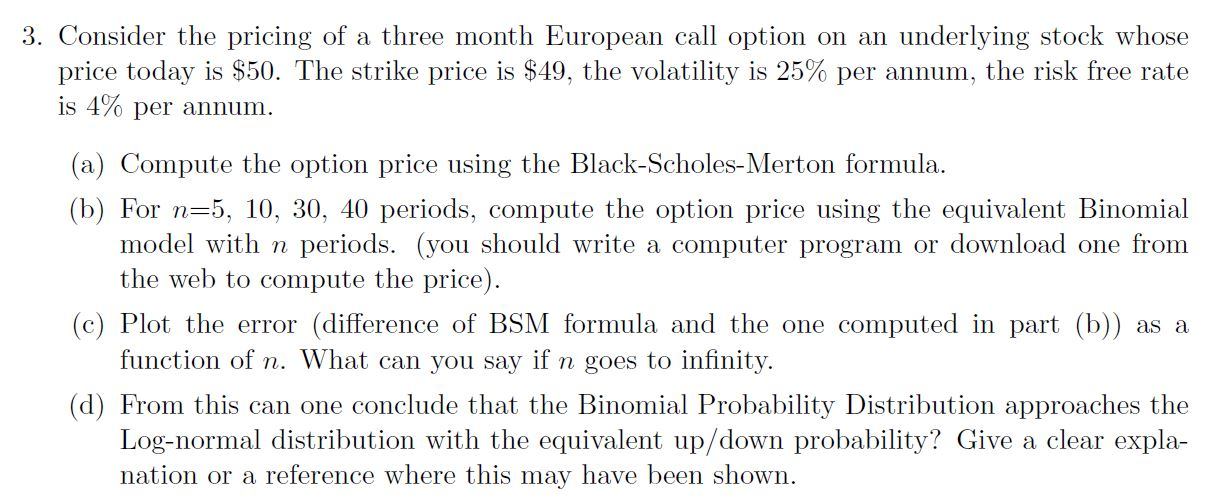
Only need answers to (c) and (d)

(c) Plot the error (difference of BSM formula and the one computed in part (b)) as a function of n. What can you say if n goes to infinity.

(d) From this can one conclude that the Binomial Probability Distribution approaches the Log-normal distribution with the equivalent up/down probability? Give a clear expla- nation or a reference where this may have been shown.

3. Consider the pricing of a three month European call option on an underlying stock whose price today is $50. The strike price is $49, the volatility is 25% per annum, the risk free rate is 4% per annum. (a) Compute the option price using the Black-Scholes-Merton formula. (b) For n=5, 10, 30, 40 periods, compute the option price using the equivalent Binomial model with n periods. (you should write a computer program or download one from the web to compute the price). (c) Plot the error (difference of BSM formula and the one computed in part (b)) as a function of n. What can you say if n goes to infinity. From this can one conclude that the Binomial Probability Distribution approaches the Log-normal distribution with the equivalent up/down probability? Give a clear expla- nation or a reference where this may have been shown. 3. Consider the pricing of a three month European call option on an underlying stock whose price today is $50. The strike price is $49, the volatility is 25% per annum, the risk free rate is 4% per annum. (a) Compute the option price using the Black-Scholes-Merton formula. (b) For n=5, 10, 30, 40 periods, compute the option price using the equivalent Binomial model with n periods. (you should write a computer program or download one from the web to compute the price). (c) Plot the error (difference of BSM formula and the one computed in part (b)) as a function of n. What can you say if n goes to infinity. From this can one conclude that the Binomial Probability Distribution approaches the Log-normal distribution with the equivalent up/down probability? Give a clear expla- nation or a reference where this may have been shown