P6.10
Scuba divers carry ballast weights to have neutral buoyancy. At that condition,
the buoyancy force on the diver exactly balances weight, and there is no
tendency either to float toward the surface or to sink. In freshwater, a certain
diver carries 10 lb of lead alloy ballast of density 1.17 104
kg/m3
. During
an excursion in seawater, the diver must carry 50% more ballast to remain
neutrally buoyant. How much does this diver weigh?
P6.11
Examine the transition between the laminar and turbulent flows of water by
sketching the stream of water that exits from a faucet (without an aerator) or
hose (without a nozzle). You can control the velocity of the stream by adjusting
the water valve.
(a) Make sketches of the flow for four different fluid speeds: two below and
two above the laminar-turbulent transition point.
(b) Estimate the velocity of the water by determining the time Dt required
to fill a container of known volume, such as a beverage container. By
measuring the diameter of the stream with a ruler, calculate the average
speed of the water from Equations (6.9) and (6.10).
(c) For each speed, calculate the Reynolds number.
(d) Indicate the value of the Reynolds number where turbulence begins.
P6.12
Water flows through a 5 cm diameter pipe at the average velocity of 1.25 m/s.
(a) In the dimensions of L/s, what is the volumetric flow rate?
(b) If the diameter of the pipe is reduced by 20% at a constriction, by what
percentage does the water's velocity change?
P6.13
In a pipeline that connects a production oilfield to a tanker terminal, oil having
density 1.85 slug/ft3
and viscosity 6 10?3 slug/(ft s) flows through a 48 in.
diameter pipeline at 6 mph. What is the Reynolds number? Is the flow laminar
or turbulent
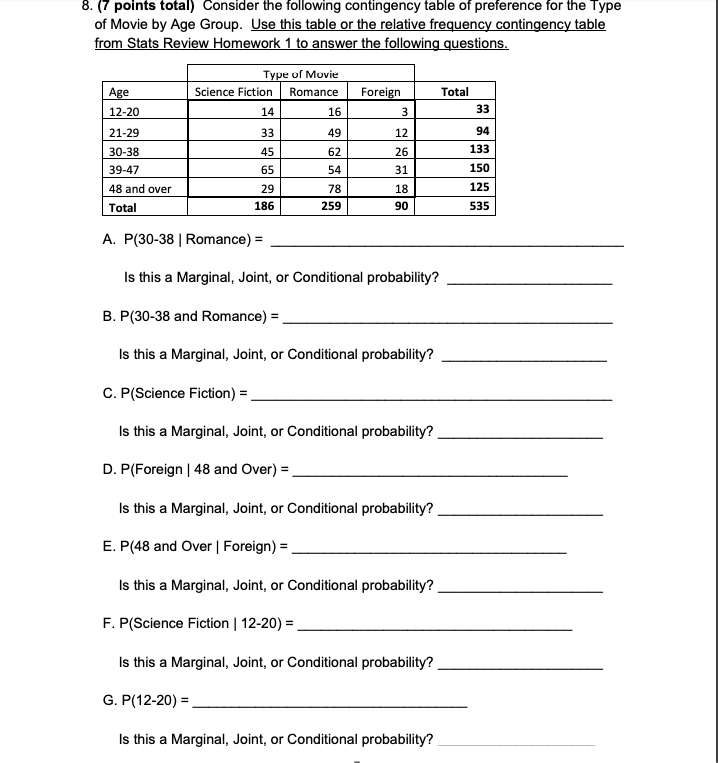
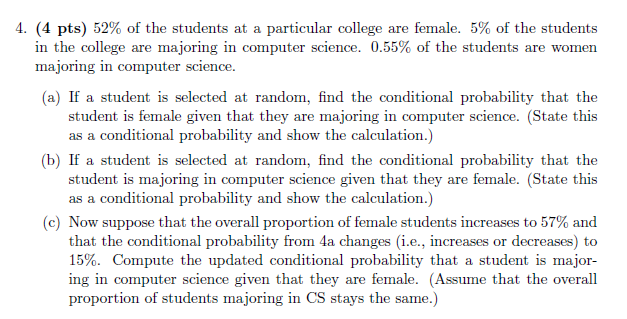
8. (7 points total) Consider the following contingency table of preference for the Type of Movie by Age Group. Use this table or the relative frequency contingency table from Stats Review Homework 1 to answer the following questions. Type of Movie Age Science Fiction Romance Foreign Total 12-20 14 16 3 33 21-29 33 49 12 94 30-38 45 62 26 133 39-47 65 54 31 150 48 and over 29 78 18 125 Total 186 259 90 535 A. P(30-38 | Romance) = Is this a Marginal, Joint, or Conditional probability? B. P(30-38 and Romance) = Is this a Marginal, Joint, or Conditional probability? C. P(Science Fiction) = Is this a Marginal, Joint, or Conditional probability? D. P(Foreign | 48 and Over) = Is this a Marginal, Joint, or Conditional probability? E. P(48 and Over | Foreign) = Is this a Marginal, Joint, or Conditional probability? F. P(Science Fiction | 12-20) = Is this a Marginal, Joint, or Conditional probability? G. P(12-20) = Is this a Marginal, Joint, or Conditional probability?4.57 The following data relate to the proportions in a population of drivers. A = Defensive driver training last year B = Accident in current year 4.55 Suppose that P(A) = .68, P(B) = .55, and P(AB) = .35. Find (a) The conditional probability that B occurs given that A occurs. (b) The conditional probability that B does not occur given that A oc- curs. (c) The conditional probability that B occurs given that A does not oc- cur. 4.56 Suppose that P(A) = .4, P(B) = .5 and the probability that either A or B occurs is .7. Find (a) The conditional probability that A occurs given that B occurs. (b) The conditional probability that B occurs given that A does not oc- cur.Answer the following questions about conditional probability. Complete parts (a) and (b) below. a. What is conditional probability? Select the correct answer below. IVA. The conditional probability of an event is the probability that one event occurs, under the assumption that another event occurs. B. The conditional probability of an event is the probability that both events occur. C. The conditional probability of an event is the probability that either event occurs, but not both. D. The conditional probability of an event is the probability that either event occurs, divided by the probability of the given event. b. Which event is the "given event"? Select the correct answer below. 0 A. The given event is the event that is conditional upon the event that is assumed to have occurred. The outcomes of the given event are restricted by the assumed event, and the probability that the given event occurs is necessary to calculate the conditional probability. 0 B. The iven event is the event that is assumed to have occurred. The given event can restrict the outcomes available to the other event, and the probability that the 9 given event occurs is usually necessary to calculate the conditional probability. 0 C. The given event is the event that all individual events occur. That is, for events A, B, C, and so on, the given event is the event (A & B & C & - - - ). O D. The given event is the event with probability 1. The given event must be certain to occur. vi. {4 pts) 52% of the students at a particular college are female. 5% of the students in the college are majoring in computer science. {1.55%. of the students are women majoring in computer science. [a] If a student is selected at random, nd the conditional probability that the student is female given that they are majoring in computer science. {State this as a conditional probability and show the calculation.) {b} If a student is selected at random, nd the conditional probability that the student is majoring in computer science given that they are female. {State this as a conditional probability and show the calculation.) [c] Now suppose that the overall proportion of female students increases to 57% and that the conditional probability from dia changes [i.e., increases or decreases]: to 15%. Compute the updated conditional probability that a student is major ing in computer science given that they are female. {Assmne that the overall proportion of students majoring in CS stays the same.)