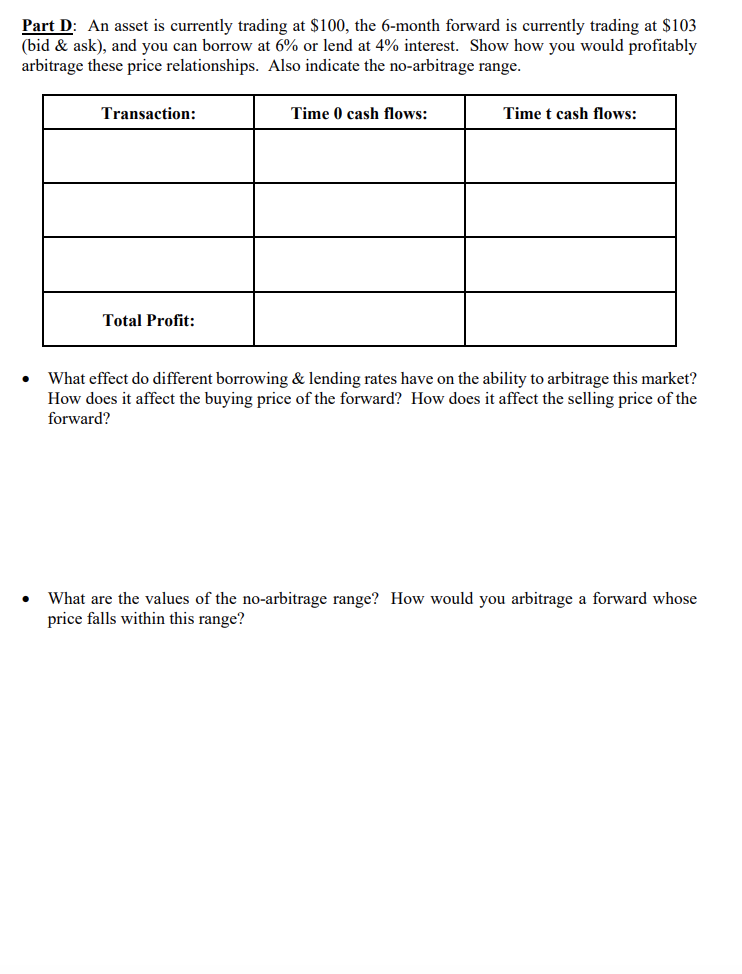
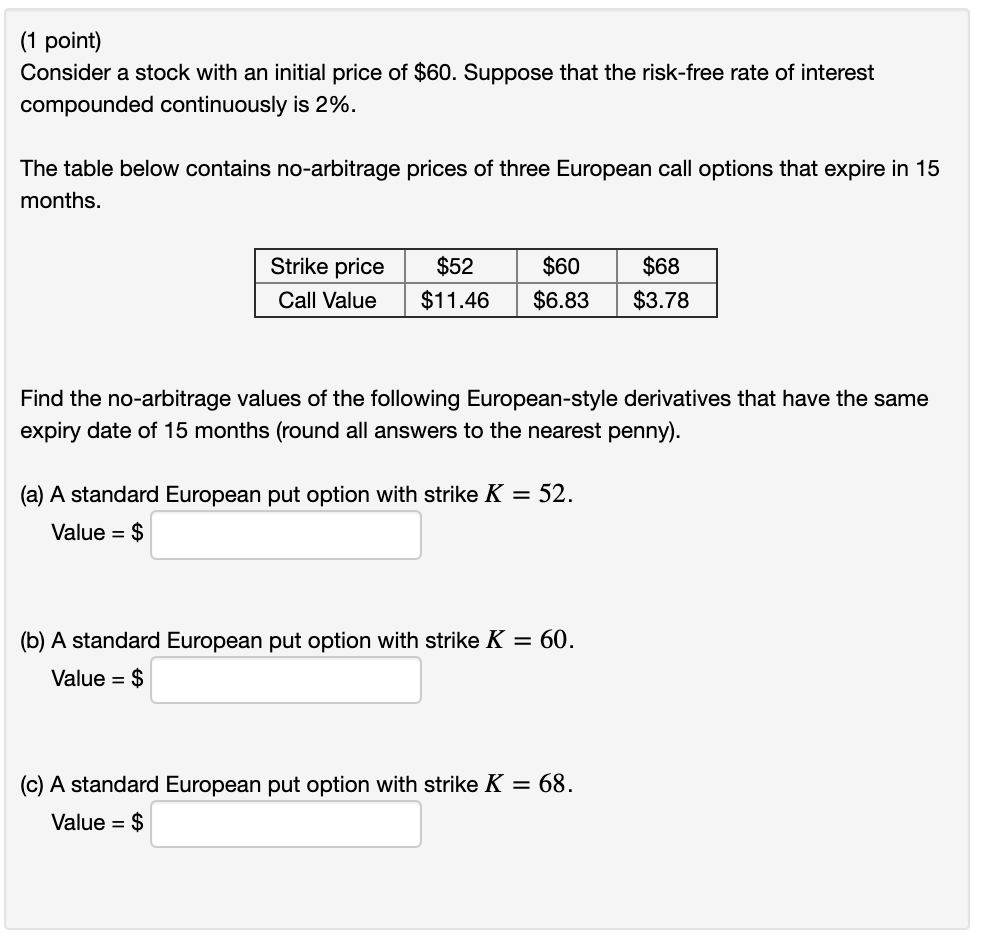
Part D: An asset is currently trading at $100, the 6-month forward is currently trading at $103 (bid & ask), and you can borrow at 6% or lend at 4% interest. Show how you would profitably arbitrage these price relationships. Also indicate the no-arbitrage range. Transaction: Total Profit: Time 0 cash flows: Time t cash flows: . What effect do different borrowing & lending rates have on the ability to arbitrage this market? How does it affect the buying price of the forward? How does it affect the selling price of the forward? What are the values of the no-arbitrage range? How would you arbitrage a forward whose price falls within this range? (1 point) Consider a stock with an initial price of $60. Suppose that the risk-free rate of interest compounded continuously is 2%. The table below contains no-arbitrage prices of three European call options that expire in 15 months. Strike price $52 Call Value $11.46 $60 $6.83 $68 $3.78 Find the no-arbitrage values of the following European-style derivatives that have the same expiry date of 15 months (round all answers to the nearest penny). (a) A standard European put option with strike K = 52. Value = $ (b) A standard European put option with strike K = 60. Value = $ (c) A standard European put option with strike K = 68. Value = $ Derivatives & Risk Management - Options Market Pricing Models) Be able to explain and apply the binomial options pricing model (BOPM): o This applies to both the arbitrage pricing version (i.e., the 5 steps version) and the risk neutral pricing version. o What are the probabilities of the up and down state-of-nature (S.O.N.) for any given option? How does this relate to the expected moneyness of an option? o What is the "economic story" behind the BOPM. Explain the logic and how it leads to an equilibrium price that can be enforced with the Law of One Price (i.e., how the call premium can be arbitrage enforced). There are lots of parts to this story, but remember that the foundation of this model is the concept of a replicating portfolio (aka the "partially covered call"). o Explain why the no-arbitrage condition must exist for a call. In other words, explain what it means for a call to be over-priced or under-priced relative to the BOPM. EVEN PARTIAL ANSWERS APPRECIATED if unable to provide an entire answer