Question
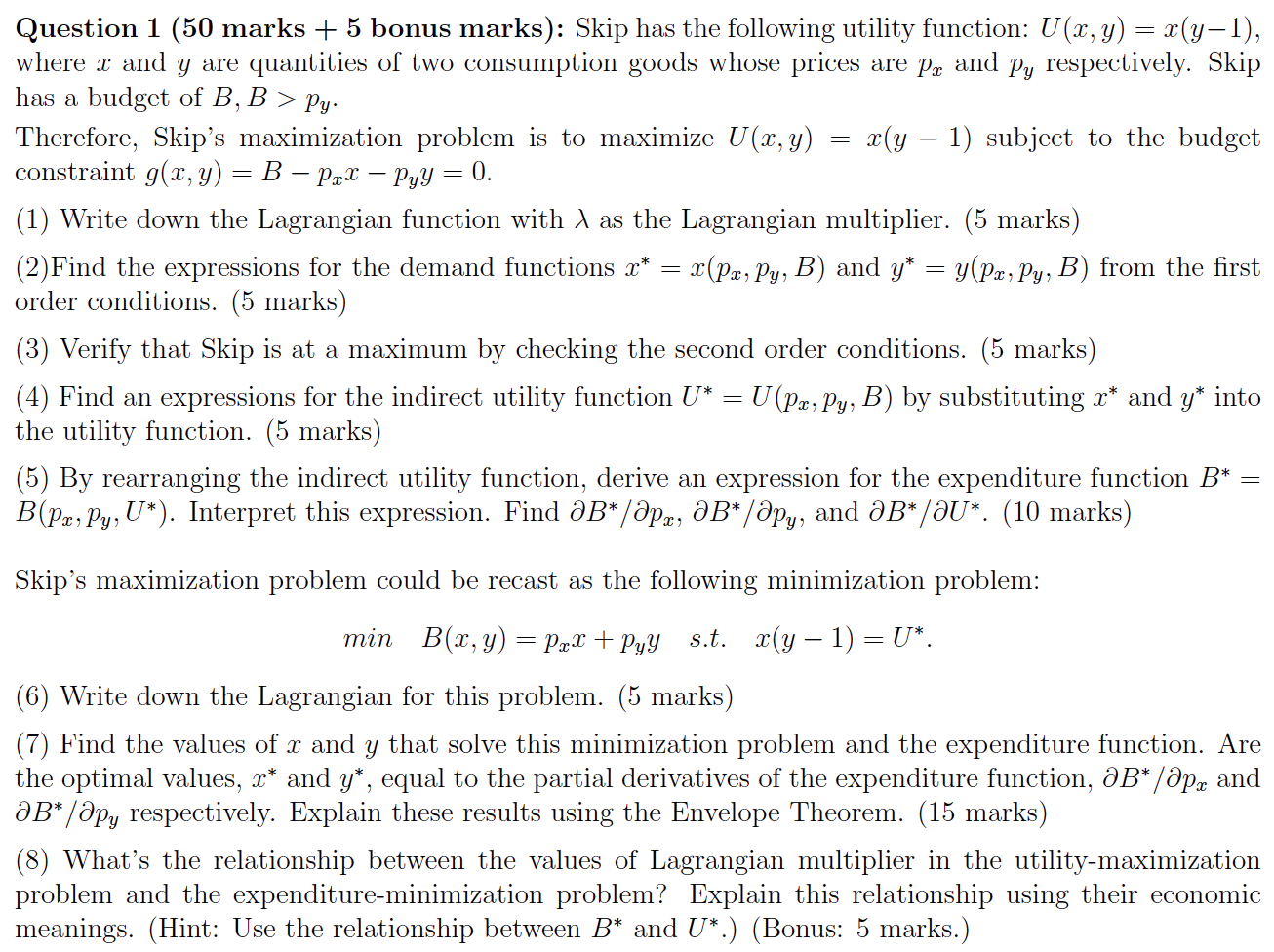
Please answer questions #5-8. Question 1 (50 marks + 5 bonus marks): Skip has the following utility function: U(x; y) = x(y1), where x and
Please answer questions #5-8.
Question 1 (50 marks + 5 bonus marks): Skip has the following utility function: U(x; y) = x(y1),
where x and y are quantities of two consumption goods whose prices are px and py respectively. Skip
has a budget of B; B > py.
Therefore, Skip's maximization problem is to maximize U(x; y) = x(y 1) subject to the budget
constraint g(x; y) = B pxx pyy = 0:
(5) By rearranging the indirect utility function, derive an expression for the expenditure function B =
B(px; py; U). Interpret this expression. Find @B=@px, @B=@py, and @B=@U. (10 marks)
Skip's maximization problem could be recast as the following minimization problem:
min B(x; y) = pxx + pyy s:t: x(y 1) = U:
(6) Write down the Lagrangian for this problem. (5 marks)
(7) Find the values of x and y that solve this minimization problem and the expenditure function. Are
the optimal values, x and y, equal to the partial derivatives of the expenditure function, @B=@px and
@B=@py respectively. Explain these results using the Envelope Theorem. (15 marks)
(8) What's the relationship between the values of Lagrangian multiplier in the utility-maximization
problem and the expenditure-minimization problem? Explain this relationship using their economic
meanings. (Hint: Use the relationship between B and U.) (Bonus: 5 marks.)
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


