Please assist me

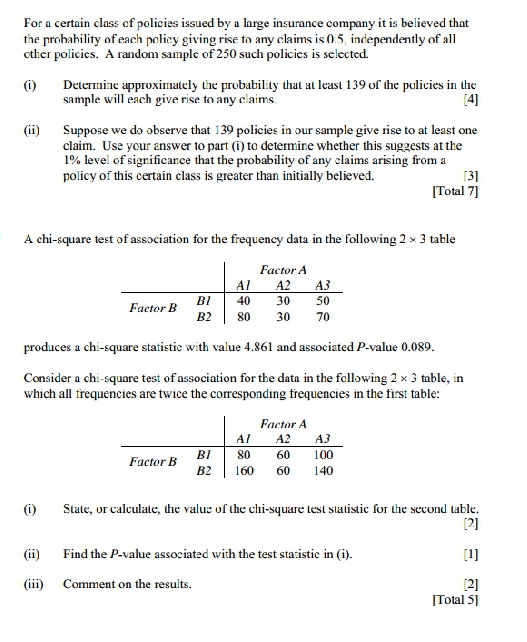
For a certain class of policies issued by a large insurance company it is believed that the probability of each policy giving rise to any claims is 0.5, independently of all other policies. A random sample of 250 such policies is selected. (i) Determine approximately the probability that at least 139 of the policies in the sample will each give rise to any claims. (4] (ii) Suppose we do observe that 139 policies in our sample give rise to at least one claim. Use your answer to part (i) to determine whether this suggests at the 1% level of significance that the probability of any claims arising from a policy of this certain class is greater than initially believed. 131 [Total 7] A chi-square test of association for the frequency data in the following 2 x 3 table Factor A Al A2 43 BI 30 Factor B 40 50 B2 80 30 70 produces a chi-square statistic with value 4.861 and associated P-value 0.089. Consider a chi-square test of association for the data in the following 2 x 3 table, in which all frequencies are twice the corresponding frequencies in the first table: Factor A A2 43 BI 80 60 100 Factor B B2 160 60 140 (i) State, or calculate, the value of the chi-square test statistic for the second table. (2] (ii) Find the P-value associated with the test statistic in (i). [1] (iii) Comment on the results. (2] [Total 51A certain town runs a training course for traffic wardens each year. The course lasts for 30 days, but the examination which enables someone to qualify as a traffic warden can be sat any day during the course. In 201 1 there were 13 participants who started the training course. The following table has been compiled to show the day cach candidate qualified or the day each candidate who did not quality left the course. Candidate Day qualified Day left without qualifying 30 21 19 12 30 10 12 30 15 10 24 (i) Explain whether the following types of censoring are present: interval censoring right censoring informative censoring (ii) Calculate the Kaplan-Meier estimate of the non-qualification function. (iii) Sketch a graph of the Kaplan-Meier estimate, labelling the axes. [2] When the data were gathered, the reasons for exit of candidates D and H were accidentally transposed, and those for candidates B and L were also accidentally transposed. (iv) Explain how your answer to part (ii) would change if you had access to the correct (i.e. untransposed) data for candidates D. H. B and L. (3] [Total 14]