Question
Please help me answer question number 5 about the work that I have done. God bless! Case Study: t-Test Comparing Means from Two Sets of
Please help me answer question number 5 about the work that I have done. God bless!
Case Study: t-Test Comparing Means from Two Sets of Data Template
- INDEPENDENT t-Test
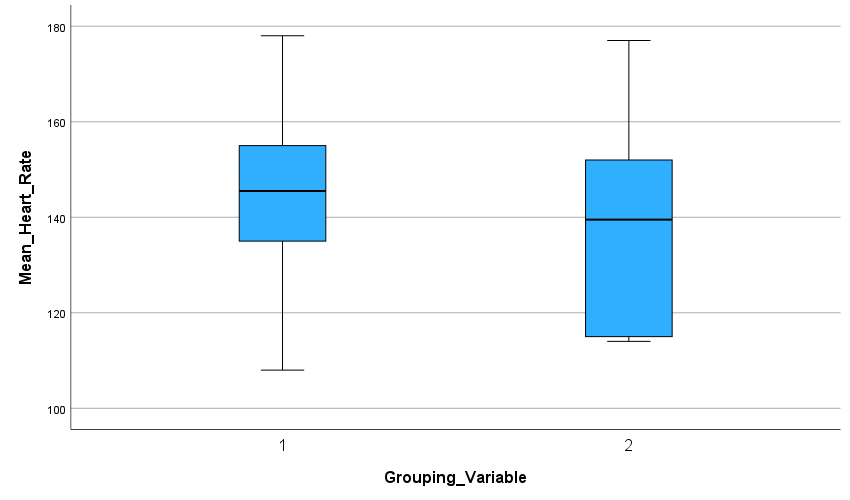
Research question "is there a statistical difference for mean heart rate between participants either exposed to a hot (Group 1) - or cold environment (Group 2)?"
Assumptions Testing
- Data level of measurement-what was the level of measurement for the variables used in this case study?
The level of measurement for the variables hot and cold was nominal, and for the variable mean heart rate, it was interval.
- Would the amount of skewness and kurtosis in these variables affect the analysis? How do you know? Give numerical values to support your conclusion.
The skewness and kurtosis in these variables did not affect the analysis because skewness =.678, kurtosis =.1.334, and they are not between -.1.96 and 1.96 intervals.
Table 1. PASTE TABLE with skewness and kurtosis BELOW
Descriptives | ||||
Grouping_Variable | Statistic | Std. Error | ||
Mean_Heart_Rate | 1 | Mean | 143.30 | 6.551 |
95% Confidence Interval for Mean | Lower Bound | 128.48 | ||
Upper Bound | 158.12 | |||
5% Trimmed Mean | 143.33 | |||
Median | 145.50 | |||
Variance | 429.122 | |||
Std. Deviation | 20.715 | |||
Minimum | 108 | |||
Maximum | 178 | |||
Range | 70 | |||
Interquartile Range | 27 | |||
Skewness | -.332 | .687 | ||
Kurtosis | .216 | 1.334 | ||
2 | Mean | 140.20 | 6.867 | |
95% Confidence Interval for Mean | Lower Bound | 124.67 | ||
Upper Bound | 155.73 | |||
5% Trimmed Mean | 139.61 | |||
Median | 139.50 | |||
Variance | 471.511 | |||
Std. Deviation | 21.714 | |||
Minimum | 114 | |||
Maximum | 177 | |||
Range | 63 | |||
Interquartile Range | 41 | |||
Skewness | .312 | .687 | ||
Kurtosis | -.679 | 1.334 |
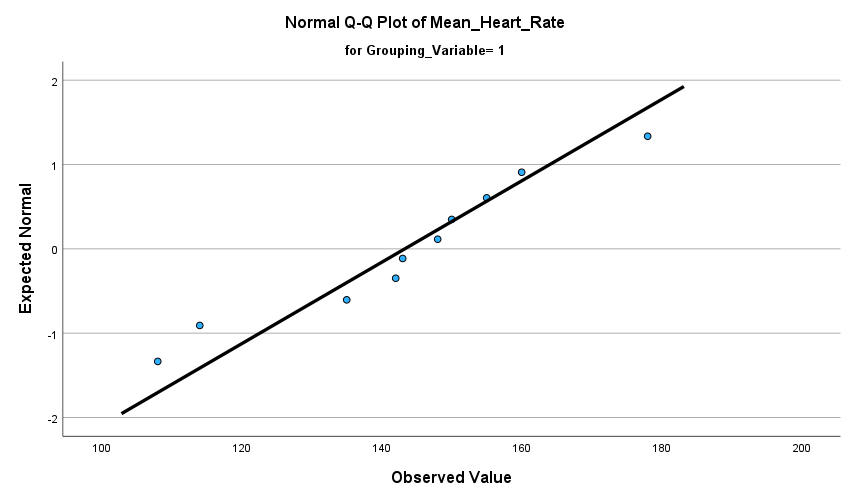
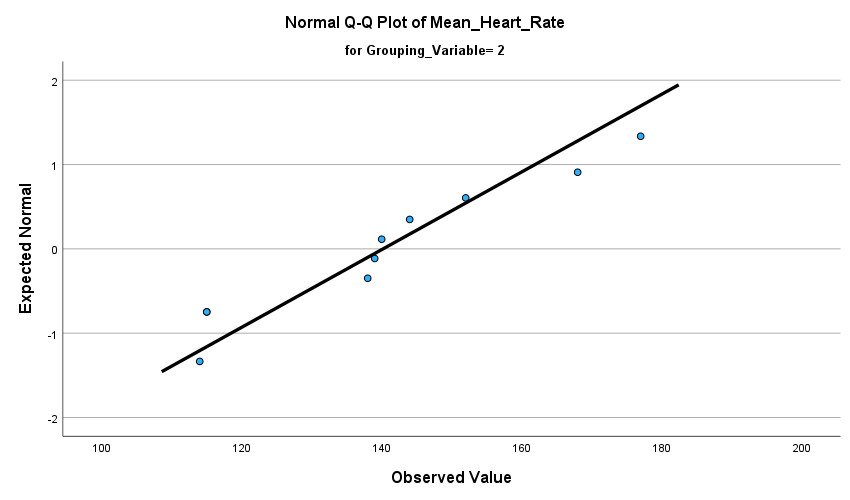
- Was the assumption of normality met and how do you know?
The assumption of normality was met because Sig. for Mean Heart Rate value 1=.739 and Sig. for Mean Heart Rate value 2=.311. They were both larger than .05.
Table 2. PASTE Tests of NORMALITY table BELOW
Tests of Normality | |||||||
Grouping_Variable | Kolmogorov-Smirnova | Shapiro-Wilk | |||||
Statistic | df | Sig. | Statistic | df | Sig. | ||
Mean_Heart_Rate | 1 | .175 | 10 | .200* | .956 | 10 | .739 |
2 | .177 | 10 | .200* | .914 | 10 | .311 | |
*. This is a lower bound of the true significance. | |||||||
a. Lilliefors Significance Correction |
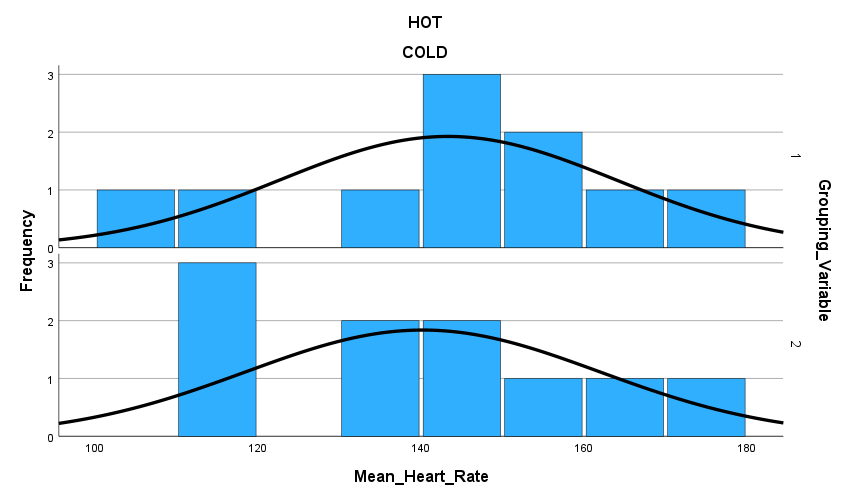
Figure 1. PASTE Histogram with normal curve for each variable BELOW
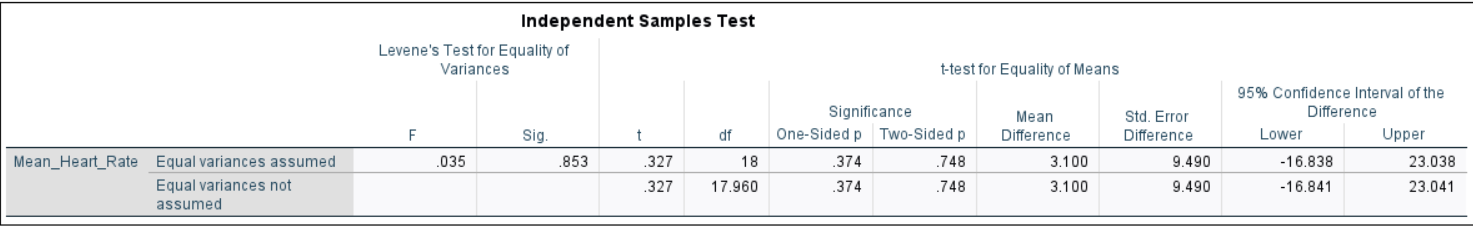
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


