Please read the Question Properly and answer all the questions properly!! Most of the time i don't correct answers or all the questions were not Answered. Thank you
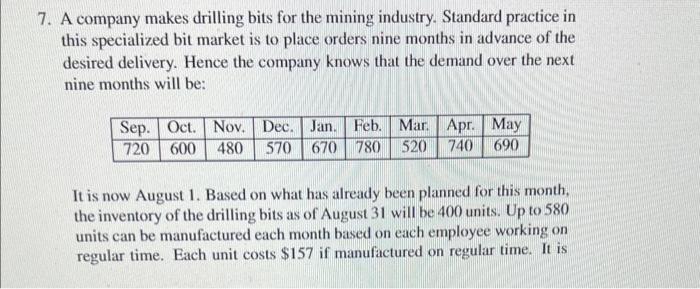
7. A company makes drilling bits for the mining industry. Standard practice in this specialized bit market is to place orders nine months in advance of the desired delivery. Hence the company knows that the demand over the next nine months will be: It is now August 1. Based on what has already been planned for this month, the inventory of the drilling bits as of August 31 will be 400 units. Up to 580 units can be manufactured each month based on each employee working on regular time. Each unit costs $157 if manufactured on regular time. It is also possible to manufacture up to 140 units per month on overtime. Each unit so produced costs $225. Drilling bits can be kept in inventory at a cost of $3 per unit per month. This charge represents the cost of tied-up capital, warehouse, and insurance expenses. As a buffer against potentially high demand at the end of the planning horizon, the ending inventory should be at least 200 units. The production level for August is 590 units ( 580 regular time plus 10 on overtime). The desired production level for May is between 500 and 620 units. The cost to increase the production level from one month to the next is $15 per unit increased, and the cost to decrease the production level from one month to the next is $22 per unit decreased. We define for t=1,,9 : Rt= the number of drilling bits produced on regular time in month t. Ot= the number of drilling bits produced on overtime in month t. It= the number of drilling bits in inventory at the end of month t. Although it is not, strictly speaking, a variable, it is useful to think of the inventory at the end of August being represented as 0. Ut= the increase in the production level from month t1 to month t. Dt= the decrease in the production level from month t1 to month t. With the restriction that all customers orders must be completed on time, we wish to formulate a model which seeks to minimize the sum of costs of regular and overtime production, inventory costs, and the costs of changing the production levels from month to month. Write the algebraic model. You will find it helpful to re-read Section 33 of Decision Modeling first. (You do not have to submit this part.) (a) Write this model using LINGO in scalar mode (as described in Section 2.6 of Decision Modeling). Submit or import both the model and solution report files. (b) Write this model using LINGO in sets mode (as described in Appendix A. 1 of Decision Modeling). Solve to verify that the solution is the same as in part (b), but just send or import the model file; you don't need to submit the solution report file as well. (a) Write this model using LINGO in scalar mode (as described in Section 2.6 of Decision Modeling). Submit or import both the model and solution report files. (b) Write this model using LINGO in sets mode (as described in Appendix A.1 of Decision Modeling). Solve to verify that the solution is the same as in part (b), but just send or import the model file; you don't need to submit the solution report file as well. 7 (c) Thinking not only of this example, but of optimization models in general, when in your opinion is one mode better than the other










