Please solve this. Thanks.

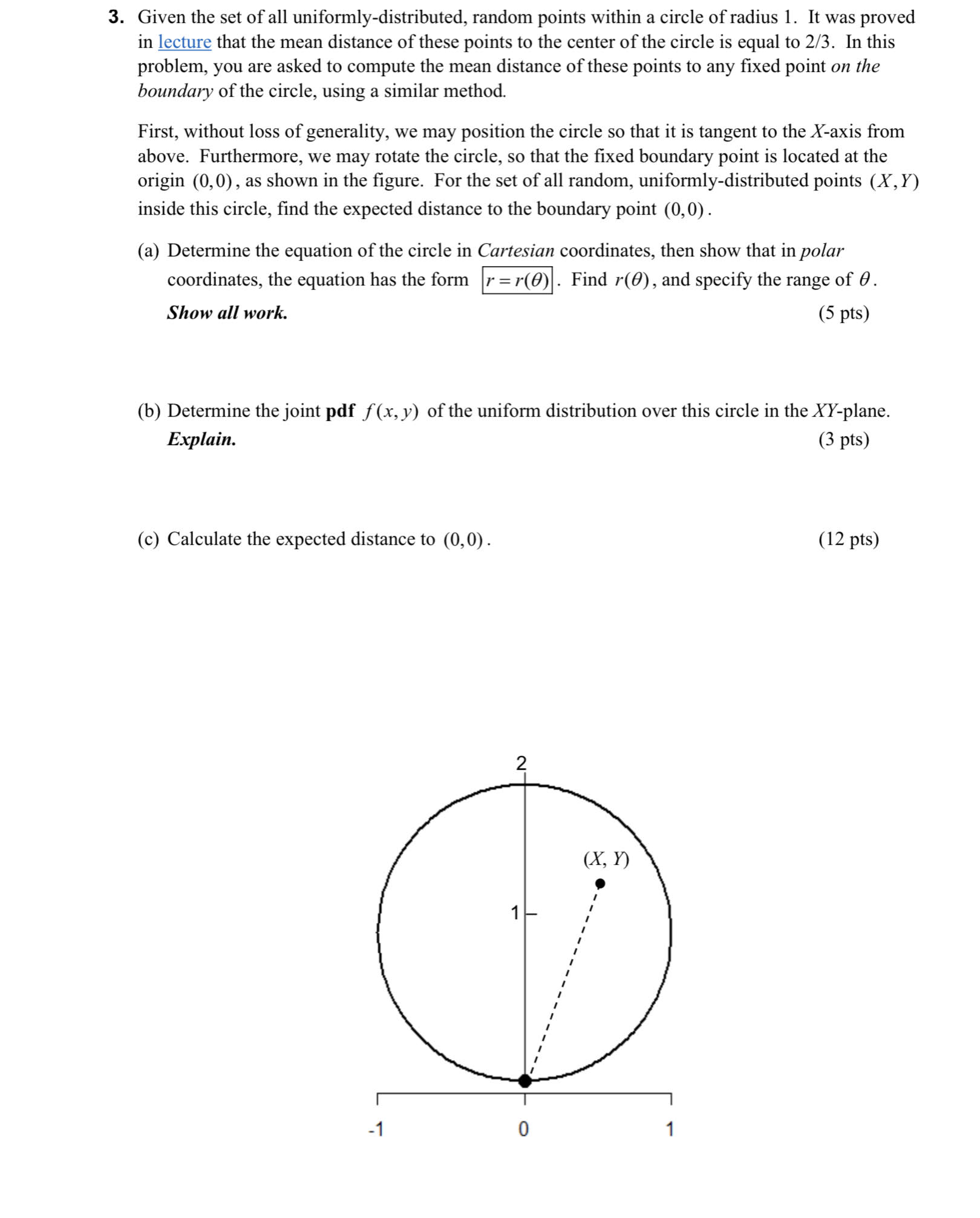3. Given the set of all uniformly-distributed, random points within a circle of radius 1. It was proved in lecture that the mean distance of these points to the center of the circle is equal to 2/3. In this problem, you are asked to compute the mean distance of these points to any xed point on the boundary of the circle, using a similar method First, without loss of generality, we may position the circle so that it is tangent to the X-axis from above. Furthermore, we may rotate the circle, so that the xed boundary point is located at the origin (0,0), as shown in the gure. For the set of all random, uniformlydistributed points (X, Y) inside this circle, nd the expected distance to the boundary point (0,0). (3) Determine the equation of the circle in Cartesian coordinates, then show that in polar coordinates, the equation has the form E. Find r09) , and specify the range of 0. Show all work. (5 pts) (b) Determine the joint pdf f (x, y) of the uniform distribution over this circle in the XYplane. Explain. (3 pts) (c) Calculate the expected distance to (0,0). (12 pts)