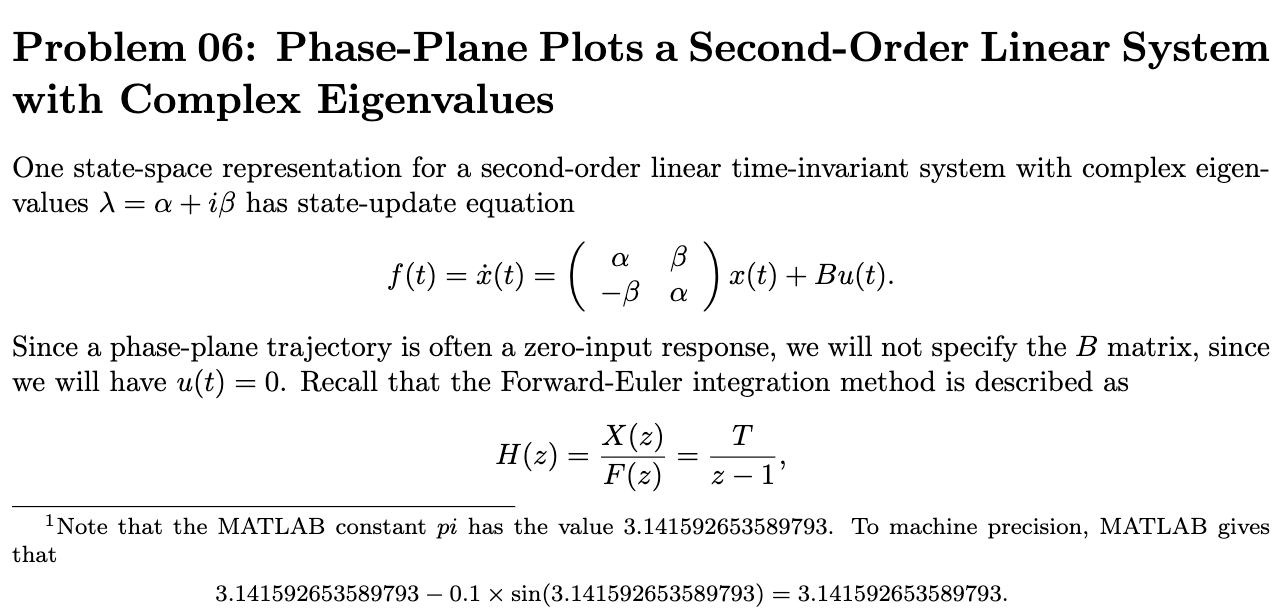
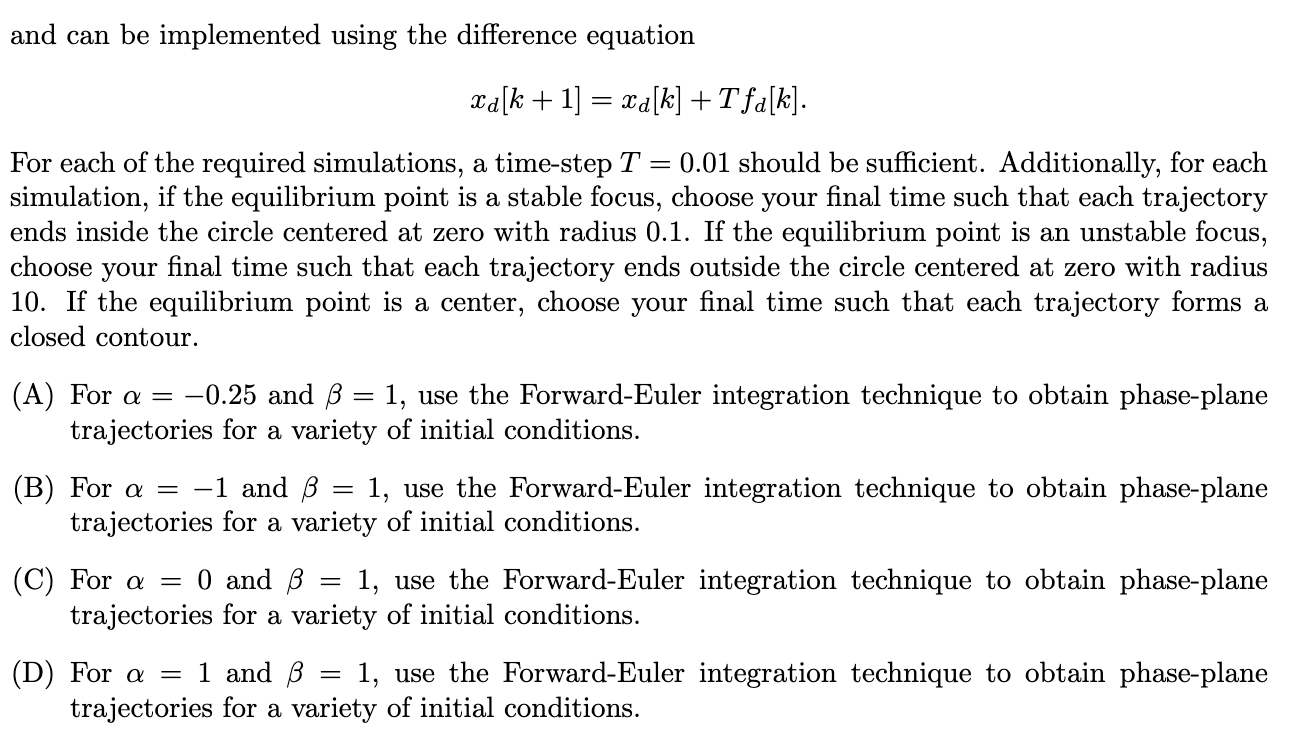
Problem 06: Phase-Plane Plots a Second-Order Linear System with Complex Eigenvalues One state-space representation for a second-order linear time-invariant system with complex eigen- values = a + i has state-update equation f(t) = c(t) = (%& ) 3(e) + Bu(t). Since a phase-plane trajectory is often a zero-input response, we will not specify the B matrix, since we will have u(t) = 0. Recall that the Forward-Euler integration method is described as X(2) T H(2) = F(2) -1' 1 Note that the MATLAB constant pi has the value 3.141592653589793. To machine precision, MATLAB gives that 3.141592653589793 0.1 x sin(3.141592653589793) = 3.141592653589793. 2 - and can be implemented using the difference equation xa[k + 1] = xd[k] + T fa[k]. = For each of the required simulations, a time-step T 0.01 should be sufficient. Additionally, for each simulation, if the equilibrium point is a stable focus, choose your final time such that each trajectory ends inside the circle centered at zero with radius 0.1. If the equilibrium point is an unstable focus, choose your final time such that each trajectory ends outside the circle centered at zero with radius 10. If the equilibrium point is a center, choose your final time such that each trajectory forms a closed contour. (A) For a = -0.25 and B = 1, use the Forward-Euler integration technique to obtain phase-plane trajectories for a variety of initial conditions. = (B) For a = -1 and B 1, use the Forward-Euler integration technique to obtain phase-plane trajectories for a variety of initial conditions. (C) For a = O and B 1, use the Forward-Euler integration technique to obtain phase-plane trajectories for a variety of initial conditions. (D) For a = 1 and 1, use the Forward-Euler integration technique to obtain phase-plane trajectories for a variety of initial conditions. Problem 06: Phase-Plane Plots a Second-Order Linear System with Complex Eigenvalues One state-space representation for a second-order linear time-invariant system with complex eigen- values = a + i has state-update equation f(t) = c(t) = (%& ) 3(e) + Bu(t). Since a phase-plane trajectory is often a zero-input response, we will not specify the B matrix, since we will have u(t) = 0. Recall that the Forward-Euler integration method is described as X(2) T H(2) = F(2) -1' 1 Note that the MATLAB constant pi has the value 3.141592653589793. To machine precision, MATLAB gives that 3.141592653589793 0.1 x sin(3.141592653589793) = 3.141592653589793. 2 - and can be implemented using the difference equation xa[k + 1] = xd[k] + T fa[k]. = For each of the required simulations, a time-step T 0.01 should be sufficient. Additionally, for each simulation, if the equilibrium point is a stable focus, choose your final time such that each trajectory ends inside the circle centered at zero with radius 0.1. If the equilibrium point is an unstable focus, choose your final time such that each trajectory ends outside the circle centered at zero with radius 10. If the equilibrium point is a center, choose your final time such that each trajectory forms a closed contour. (A) For a = -0.25 and B = 1, use the Forward-Euler integration technique to obtain phase-plane trajectories for a variety of initial conditions. = (B) For a = -1 and B 1, use the Forward-Euler integration technique to obtain phase-plane trajectories for a variety of initial conditions. (C) For a = O and B 1, use the Forward-Euler integration technique to obtain phase-plane trajectories for a variety of initial conditions. (D) For a = 1 and 1, use the Forward-Euler integration technique to obtain phase-plane trajectories for a variety of initial conditions








