Question
Problem 3. (16 points) Consider the following two-player game. Player 1 (the row player) has three strategies {, ,}, and Player 2 (the column player)
Problem 3. (16 points) Consider the following two-player game. Player 1 (the row player) has three strategies {, ,}, and Player 2 (the column player) has four strategies {, , ,}. In each cell, the first number is the pay-off of Player 1, and the second is that of Player 2. There is an unknown in the table. Consider pure strategies only. L M N R U (0, 3) (1, 0) (2, 5) (4, 2) F (6, 0) (5, 1) (, 2) (5, 1) D (3, 1) (4, 2) (1, 1) (1, 3) (1) (5 points) Find a number such that {, } is the unique Nash equilibrium. (2) (5 points) Find a number such that the game has more than one Nash equilibria. What are these Nash equilibria in this case? (3) (6 points) Is it possible to find a number such that the game has no Nash equilibrium? For each of your answers above, an explanation needs to be provided.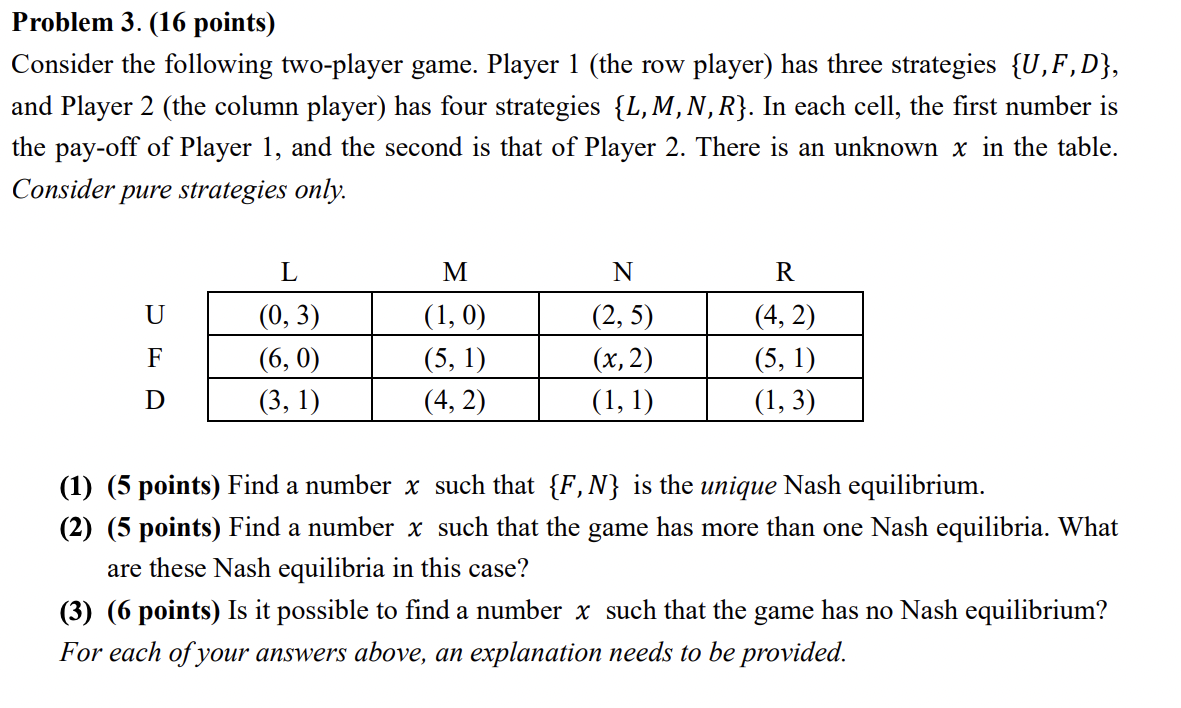
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


