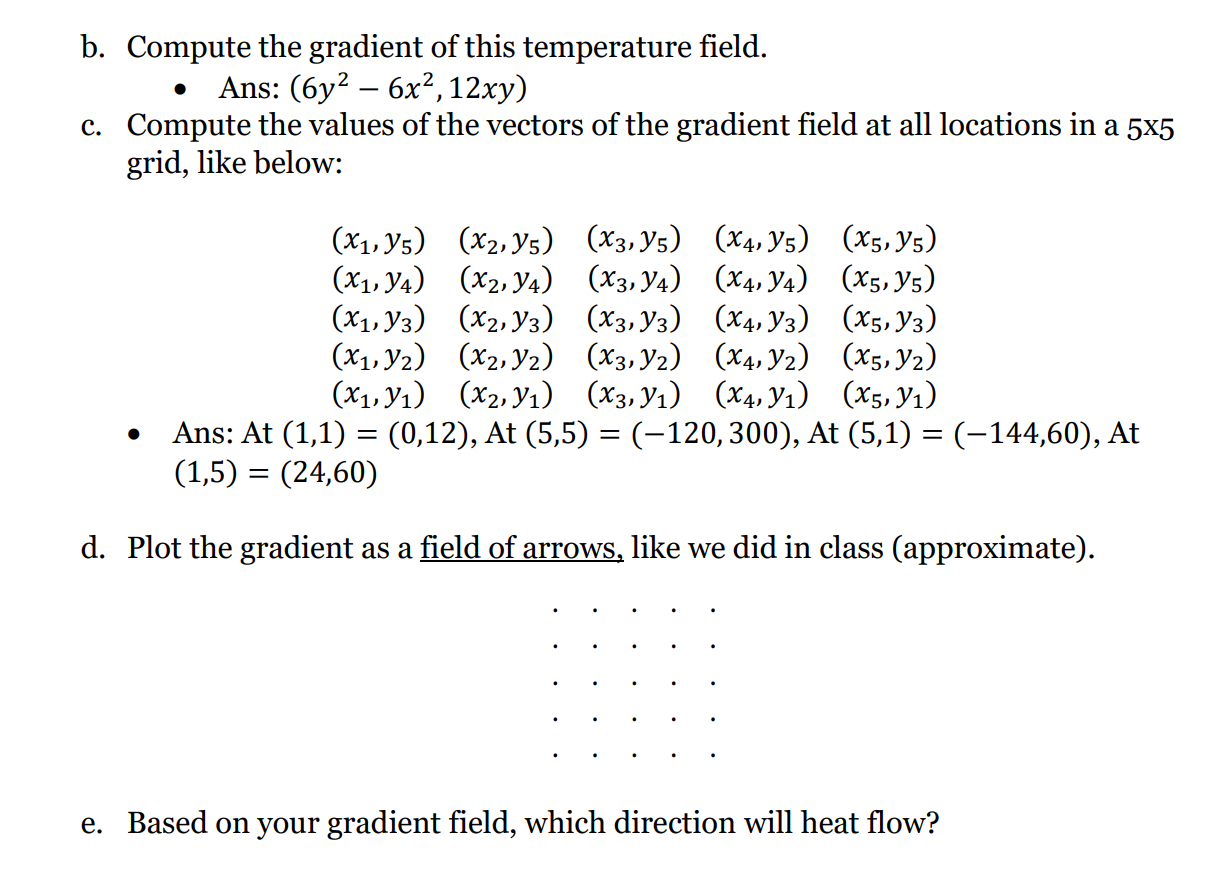
Problem 3 (Fourier's Law of Heat Flow) From a temperature field, we can use Fourier's Law to compute the heat transfer rate (which is a vector field): q(x,y) = kVT Where k is a constant known as the thermal conductivity. For the purposes of this exercise, we can assume that k is 1. We wish to apply Fourier's Law to the temperature field given in Problem 2 to find the heat transfer in that rectangular plate. Answer the following: a. Based on the unofficial rule of heat transfer (i.e. heat flows from hot stuff the cold stuff), briefly describe how you expect heat to flow in our rectangular plate. Which corner will the heat go to? b. Compute the gradient of this temperature field. Ans: (6y-6x2, 12xy) c. Compute the values of the vectors of the gradient field at all locations in a 5x5 grid, like below: (x1,y5) (X2,Y5) (X3, 5) (X4, Y5) (X5, Y5) (x1, 4) (X2,Y4) (X3, 4) (X4, 4) (X5, y5) (X1,Y3) (X2,Y3) (X3, Y3) (X4, Y3) (X5, Y3) (x1,y2) (X2, y2) (X3, Y2) (x4, y2) (x5, y2) (x1,y1) (X2,Y) (X3, Y1) (x4, Y1) (5, Y1) Ans: At (1,1) = (0,12), At (5,5) = (-120,300), At (5,1) = (144,60), At (1,5) = (24,60) d. Plot the gradient as a field of arrows, like we did in class (approximate). e. Based on your gradient field, which direction will heat flow?