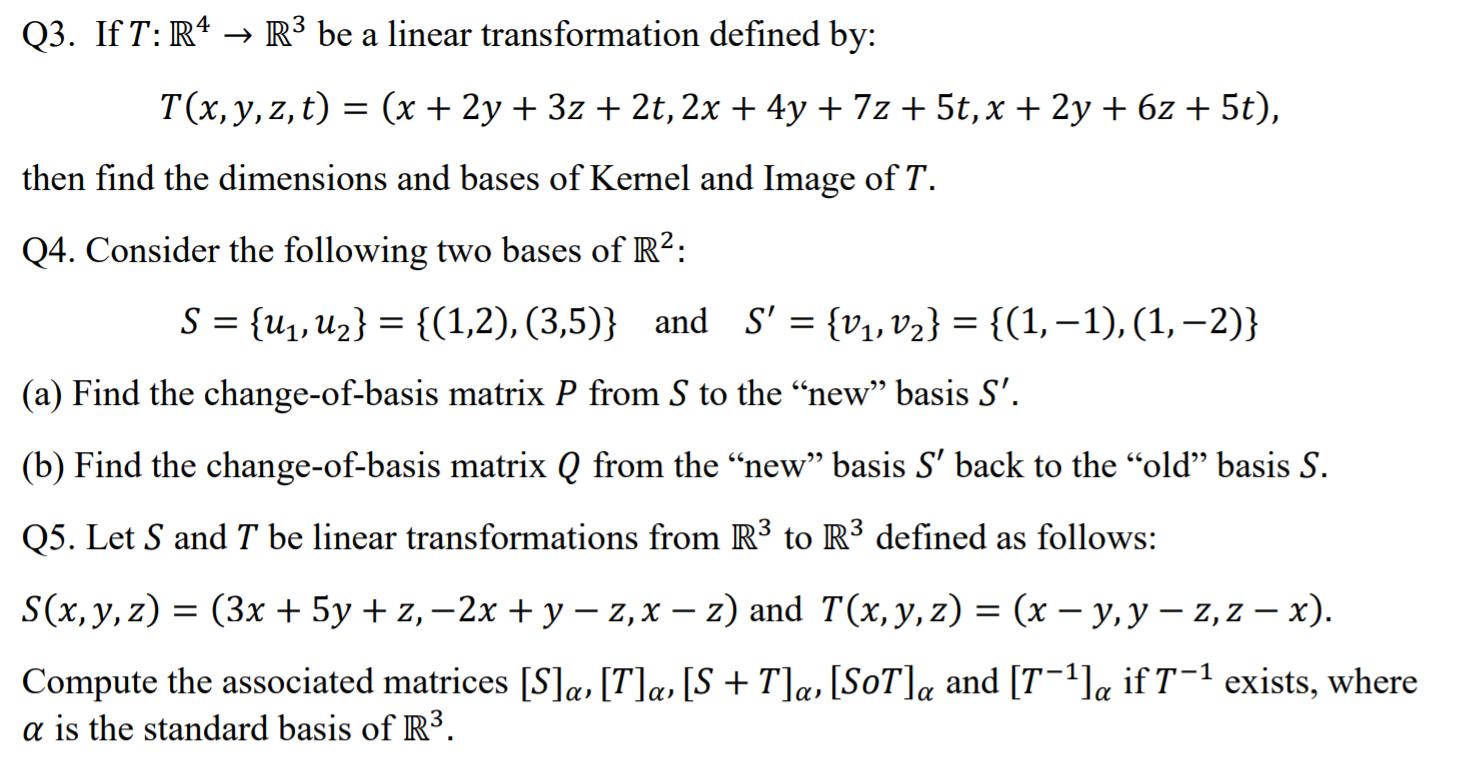
Q3. If T: R4 R be a linear transformation defined by: T(x,y, z, t) = (x + 2y + 3z + 2t, 2x + 4y + 7z + 5t, x + 2y + 6z + 5t), then find the dimensions and bases of Kernel and Image of T. Q4. Consider the following two bases of R2: S = {u1, Uz} = {(1,2), (3,5)} and S' = {v1, v2} = {(1,1), (1, 2)} (a) Find the change-of-basis matrix P from S to the "new" basis S'. (b) Find the change-of-basis matrix Q from the "new" basis S' back to the "old" basis S. Q5. Let S and T be linear transformations from R3 to R3 defined as follows: S(x, y, z) = (3x + 5y + z, 2x + y z, x z) and T(x,y,z) = (x y, y 2, z x). Compute the associated matrices [S]a, [T]a [S+ T]]a, [SoT]a and [T-1]a if T-1 exists, where a is the standard basis of R.