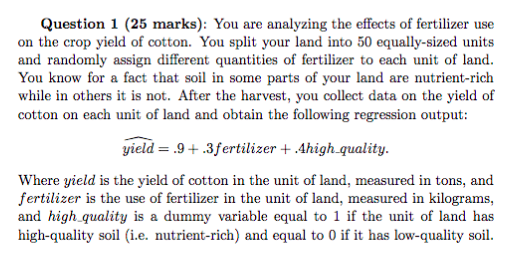

Question 1 (25 marks): You are analyzing the effects of fertilizer use on the crop yield of cotton. You split your land into 50 equally-sized units and randomly assign different quantities of fertilizer to each unit of land. You know for a fact that soil in some parts of your land are nutrient-rich while in others it is not. After the harvest, you collect data on the yield of cotton on each unit of land and obtain the following regression output: yield = 9+.3fertilizer +.Ahighquality. Where yield is the yield of cotton in the unit of land, measured in tons, and fertilizer is the use of fertilizer in the unit of land, measured in kilograms, and high quality is a dummy variable equal to 1 if the unit of land has high-quality soil (i.e. nutrient-rich) and equal to 0 if it has low-quality soil. For parts (c) to (f): When harvesting the cotton, you suspect the fertilizer attracted spider mites, a common pest found in cotton leaves. You collect data on the density of spider mites in each unit of land, re-run your regression, and obtain: yield =1+6fertilizer + .Ahigh-quality 2 spider mites. Where spider mites is a standardized measure of spider mites density on your units of land: density of mites --average density of mites atd. dev. of density of mites (c) (4 marks) Interpret the coefficient on spider mites. (d) (4 marks) Use the partialling out interpretation to explain the fact that the OLS estimate on fertilizer went from 3 in the regression without spider_mites to .6 in the regression with spider_mites. (e) (4 marks) Suppose you are deciding whether to invest in fertilizer or in improving soil quality for the next season. You will invest in fertilizer only if sufficient evidence suggests the yield increase of an additional kg of fertilizer exceeds the yield increase of having a high-quality soil. Write down the null and alternative hypothesis for the hypothesis test you would conduct to inform your investment decision. Use the algebra trick 0 = Bfertil-Bhigh qual and write down the econometric model you would estimate after plugging in Bertil = 8+Bhigh qual in the regression of yield on fertilizer, high-quality, and spider-mites. (f) (5 marks) Suppose you estimate the model in part (e) and obtain the standard error se(@OLS) = .11. Conduct the hypothesis test you set up in part (e) at a 5% significance level. Include the decision rule, as well as a sketch with the rejection region. (Hint: you should be able to calculate from the regression output relating yield to fertilizer, high quality, and spider mites.) Question 1 (25 marks): You are analyzing the effects of fertilizer use on the crop yield of cotton. You split your land into 50 equally-sized units and randomly assign different quantities of fertilizer to each unit of land. You know for a fact that soil in some parts of your land are nutrient-rich while in others it is not. After the harvest, you collect data on the yield of cotton on each unit of land and obtain the following regression output: yield = 9+.3fertilizer +.Ahighquality. Where yield is the yield of cotton in the unit of land, measured in tons, and fertilizer is the use of fertilizer in the unit of land, measured in kilograms, and high quality is a dummy variable equal to 1 if the unit of land has high-quality soil (i.e. nutrient-rich) and equal to 0 if it has low-quality soil. For parts (c) to (f): When harvesting the cotton, you suspect the fertilizer attracted spider mites, a common pest found in cotton leaves. You collect data on the density of spider mites in each unit of land, re-run your regression, and obtain: yield =1+6fertilizer + .Ahigh-quality 2 spider mites. Where spider mites is a standardized measure of spider mites density on your units of land: density of mites --average density of mites atd. dev. of density of mites (c) (4 marks) Interpret the coefficient on spider mites. (d) (4 marks) Use the partialling out interpretation to explain the fact that the OLS estimate on fertilizer went from 3 in the regression without spider_mites to .6 in the regression with spider_mites. (e) (4 marks) Suppose you are deciding whether to invest in fertilizer or in improving soil quality for the next season. You will invest in fertilizer only if sufficient evidence suggests the yield increase of an additional kg of fertilizer exceeds the yield increase of having a high-quality soil. Write down the null and alternative hypothesis for the hypothesis test you would conduct to inform your investment decision. Use the algebra trick 0 = Bfertil-Bhigh qual and write down the econometric model you would estimate after plugging in Bertil = 8+Bhigh qual in the regression of yield on fertilizer, high-quality, and spider-mites. (f) (5 marks) Suppose you estimate the model in part (e) and obtain the standard error se(@OLS) = .11. Conduct the hypothesis test you set up in part (e) at a 5% significance level. Include the decision rule, as well as a sketch with the rejection region. (Hint: you should be able to calculate from the regression output relating yield to fertilizer, high quality, and spider mites.)








