Question 1. 3D Coordinate conformal transformation is frequently used in geodesy and land surveying. The geodetic datum transformation from Hong Kong geodetic coordinates system (ITRF96) to Macao geodetic coordinates system (ITRF2005) have the following rigorous formula: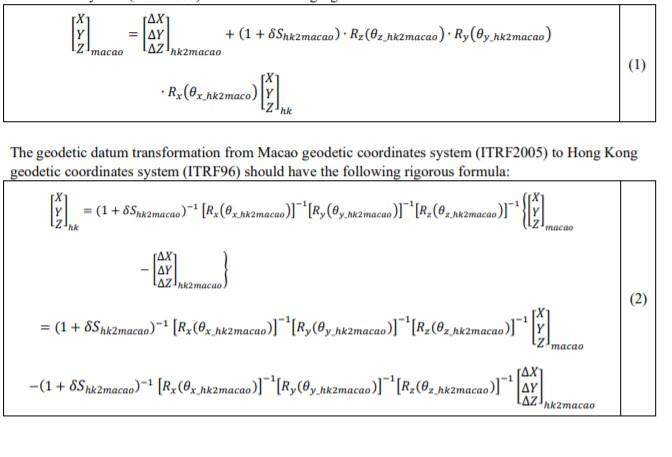
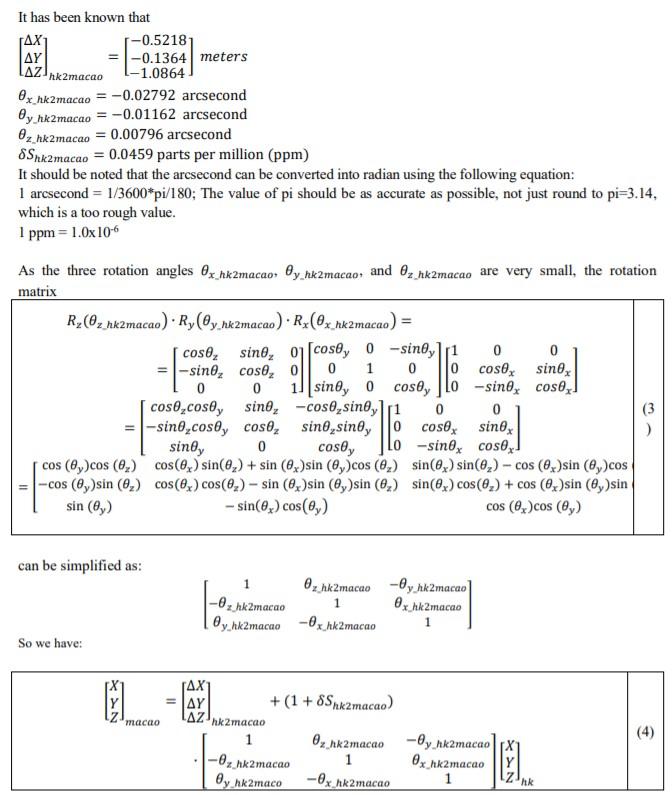
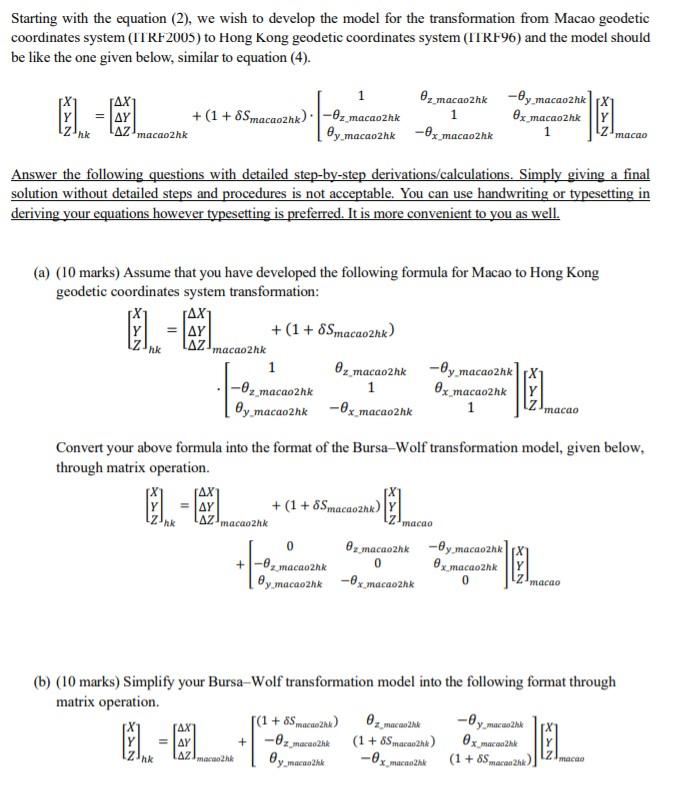
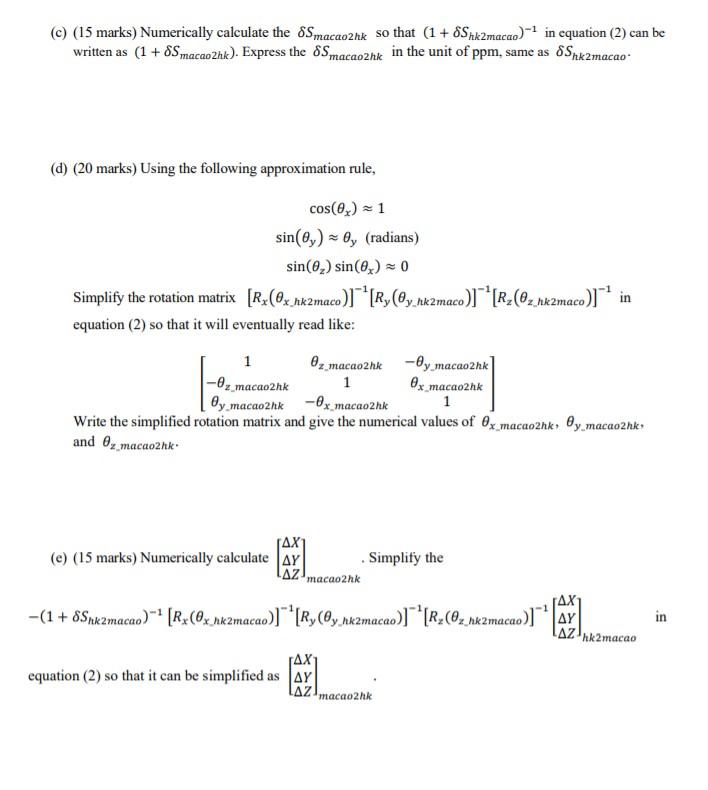
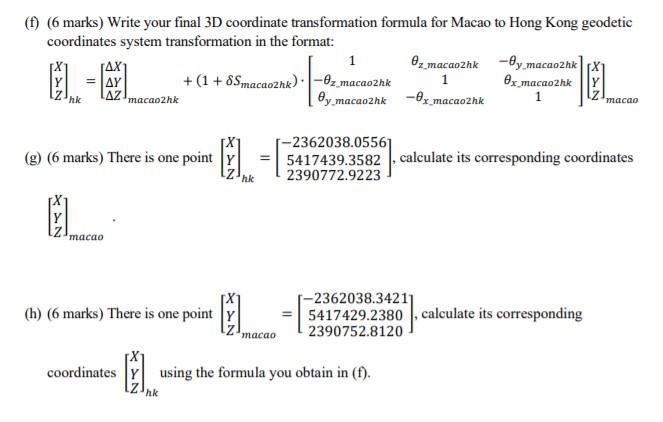
L- TAX = |) (1 + 8Snk2macao) Rz(@z_hk2macao). Ryby hk2macao) macao AZ hk2macao (1) Ry(Oxnkzmad maco) CEL The geodetic datum transformation from Macao geodetic coordinates system (ITRF2005) to Hong Kong geodetic coordinates system (ITRF96) should have the following rigorous formula: = (1 + 6Sumaca)" [8: (0xnamaca)*(R,CO, namacan)."[R_CO-semaca) " CEIL Rk macao AX AY AZ A2macao 2) = (1 + 8Sukamacao) "" [R_Ox nxzmacao) *(R,Wy hamacao))"[R:(0xnxzmacao)? -EL macao ( -(1 + 8Snk2macao) (R4(0x.hkzmacao))"[R.COy.nkzmacao))"[R,(@z_hkzmacao))" AY AZ hk2macao Lazlhk2macao It has been known that -0.5218 AY -0.1364 meters -1.0864 Ox_hk2macao = -0.02792 arcsecond By nk2macao = -0.01162 arcsecond 02 hk2macao = 0.00796 arcsecond 8Snk2macao = 0.0459 parts per million (ppm) It should be noted that the arcsecond can be converted into radian using the following equation: 1 arcsecond = 1/3600*pi/180; The value of pi should be as accurate as possible, not just round to pi=3.14, which is a too rough value. 1 ppm = 1.0x106 As the three rotation angles Ox_nk2macao Oy nk2macao, and Oz_hk2macao are very small, the rotation matrix R_C0z hk2macao). R,(@y_nk2macao hkemacao). Rx (@x_nk2macao) cose, sine, o cosey 0 -sino, 11 0 0 1-sino, cos00 0 1 0 cos, sinox 0 0 11 sin@y 0 cosey Lo-sine, cosec] cos,cose, sino, -cos,sino,1,1 0 0 |-sin8,cose, cose, sin,sino, Ilocoso, sinex 0 cosey lo-sinox cosex! cos (@y)cos (0) cos(0) sin((,) + sin (@x)sin (0)cos (@) sin(x) sin((,) - cos (0x)sin (@y)cos - cos (,)sin ((,) cos(x) cos(0.) - sin (@)sin (,)sin (0.) sin(x) cos(0) + cos (0)sin (@)sin sin (@y) - sin(x) cos(x) cos (@_)cos (@y) siny can be simplified as: Oz hk2macao Oy hk2macao -0.hk2macao Ox_hk2macao .hk2 hk2 1 1 So we have: 14X1 = LAY +(1 + 8Snk2macao) ) macao hk 2 macao 1 0, nk2macao -Oz_n2macao 1 @y_hkamaco -Ox_hk2macao - Oy_nkamacao 8x_hk2macao 1 hk Starting with the equation (2), we wish to develop the model for the transformation from Macao geodetic coordinates system (ITRF2005) to Hong Kong geodetic coordinates system (ITRF96) and the model should be like the one given below, similar to equation (4). - TAX 1 z_macaozhk-By_macao2hk = AY + (1 + 8Smacaozhk) - 8z macaozhk 1 ozhk hk Z macaozhk By macao2hk - 8x macao2hk 1 ( ) macao Answer the following questions with detailed step-by-step derivations/calculations. Simply giving a final solution without detailed steps and procedures is not acceptable. You can use handwriting or typesetting in deriving your equations however typesetting is preferred. It is more convenient to you as well. (a) (10 marks) Assume that you have developed the following formula for Macao to Hong Kong geodetic coordinates system transformation: = JAY + (1 + SSmacao2nk) 1 Oz.macao2nk -y macao2nk -Oz_macao2hk 1 0x macao2hk By macao2nk-Ox_macao2nk 1 EL hk macao2hk macao Convert your above formula into the format of the Bursa-Wolf transformation model, given below, through matrix operation. FAX1 + (1 + 8Smacaozhe) y LAZ 0 @z macaozhk-Oymacaozne lix - z macao2hk 0 ex macao2hk ly 8 y.macaozn - xmacaozink 0 IL-L = | hk 'macao2hk macao macao (b) (10 marks) Simplify your Bursa-Wolf transformation model into the following format through matrix operation. |(1 + 8 macao2x) (1 + 8Smaran2x) Ozma TAX maca21 L-OL -z_maazhk = Lazima -y_macao24 Ox_marok (1 + 8 m2 EL y_macok -Ox_mocaozhat macao () (15 marks) Numerically calculate the 8Smacao2hk so that (1 + 8Shk2macao)-4 in equation (2) can be written as (1 + 8Smacao2hk). Express the 8Smacao2nk in the unit of ppm, same as 8Shk2macao (d) (20 marks) Using the following approximation rule, cos(x) =1 sin(y) 0, (radians) sin((,) sin(x) = 0 Simplify the rotation matrix [R_(Ox hk2maco)]*[Ry@yhk2maco)) *[R (@z,hk2maco) in equation (2) so that it will eventually read like: 1 Oz_macao2hk -Oy macao2nk -Oz_macao2hk 1 Ox_macao2hk Oy.macao2nk -Ox.macao2nk 1 Write the simplified rotation matrix and give the numerical values of 0x macao2nk, Oy.macaoank and Oz macaozik TAX (e) (15 marks) Numerically calculate AY Simplify the LAZ macao2hk (1 + 8Shx2macao)[R: (0x nk?macao)** [R, Cynx2macao) "[R: (0_ nxzmacao)** | AY in Laz'h2macao equation (2) so that it can be simplified as AY Lazimacaoank (1) (6 marks) Write your final 3D coordinate transformation formula for Macao to Hong Kong geodetic coordinates system transformation in the format: 1 @z_macaozhk -By_macao2h = |AY + (1 + SSmacaozhk).- Oz_macao2hk 1 ex_macao2hk ly ) macao hk y macaozhk - 8x macao2hk 1 Y hk macao (g) (6 marks) There is one point r-2362038.05561 = 5417439.3582, calculate its corresponding coordinates 2390772.9223 - hk Y . macao (h) (6 marks) There is one point y 1-2362038.34211 5417429.2380 calculate its corresponding 2390752.8120 macao coordinates y using the formula you obtain in (f). hk L- TAX = |) (1 + 8Snk2macao) Rz(@z_hk2macao). Ryby hk2macao) macao AZ hk2macao (1) Ry(Oxnkzmad maco) CEL The geodetic datum transformation from Macao geodetic coordinates system (ITRF2005) to Hong Kong geodetic coordinates system (ITRF96) should have the following rigorous formula: = (1 + 6Sumaca)" [8: (0xnamaca)*(R,CO, namacan)."[R_CO-semaca) " CEIL Rk macao AX AY AZ A2macao 2) = (1 + 8Sukamacao) "" [R_Ox nxzmacao) *(R,Wy hamacao))"[R:(0xnxzmacao)? -EL macao ( -(1 + 8Snk2macao) (R4(0x.hkzmacao))"[R.COy.nkzmacao))"[R,(@z_hkzmacao))" AY AZ hk2macao Lazlhk2macao It has been known that -0.5218 AY -0.1364 meters -1.0864 Ox_hk2macao = -0.02792 arcsecond By nk2macao = -0.01162 arcsecond 02 hk2macao = 0.00796 arcsecond 8Snk2macao = 0.0459 parts per million (ppm) It should be noted that the arcsecond can be converted into radian using the following equation: 1 arcsecond = 1/3600*pi/180; The value of pi should be as accurate as possible, not just round to pi=3.14, which is a too rough value. 1 ppm = 1.0x106 As the three rotation angles Ox_nk2macao Oy nk2macao, and Oz_hk2macao are very small, the rotation matrix R_C0z hk2macao). R,(@y_nk2macao hkemacao). Rx (@x_nk2macao) cose, sine, o cosey 0 -sino, 11 0 0 1-sino, cos00 0 1 0 cos, sinox 0 0 11 sin@y 0 cosey Lo-sine, cosec] cos,cose, sino, -cos,sino,1,1 0 0 |-sin8,cose, cose, sin,sino, Ilocoso, sinex 0 cosey lo-sinox cosex! cos (@y)cos (0) cos(0) sin((,) + sin (@x)sin (0)cos (@) sin(x) sin((,) - cos (0x)sin (@y)cos - cos (,)sin ((,) cos(x) cos(0.) - sin (@)sin (,)sin (0.) sin(x) cos(0) + cos (0)sin (@)sin sin (@y) - sin(x) cos(x) cos (@_)cos (@y) siny can be simplified as: Oz hk2macao Oy hk2macao -0.hk2macao Ox_hk2macao .hk2 hk2 1 1 So we have: 14X1 = LAY +(1 + 8Snk2macao) ) macao hk 2 macao 1 0, nk2macao -Oz_n2macao 1 @y_hkamaco -Ox_hk2macao - Oy_nkamacao 8x_hk2macao 1 hk Starting with the equation (2), we wish to develop the model for the transformation from Macao geodetic coordinates system (ITRF2005) to Hong Kong geodetic coordinates system (ITRF96) and the model should be like the one given below, similar to equation (4). - TAX 1 z_macaozhk-By_macao2hk = AY + (1 + 8Smacaozhk) - 8z macaozhk 1 ozhk hk Z macaozhk By macao2hk - 8x macao2hk 1 ( ) macao Answer the following questions with detailed step-by-step derivations/calculations. Simply giving a final solution without detailed steps and procedures is not acceptable. You can use handwriting or typesetting in deriving your equations however typesetting is preferred. It is more convenient to you as well. (a) (10 marks) Assume that you have developed the following formula for Macao to Hong Kong geodetic coordinates system transformation: = JAY + (1 + SSmacao2nk) 1 Oz.macao2nk -y macao2nk -Oz_macao2hk 1 0x macao2hk By macao2nk-Ox_macao2nk 1 EL hk macao2hk macao Convert your above formula into the format of the Bursa-Wolf transformation model, given below, through matrix operation. FAX1 + (1 + 8Smacaozhe) y LAZ 0 @z macaozhk-Oymacaozne lix - z macao2hk 0 ex macao2hk ly 8 y.macaozn - xmacaozink 0 IL-L = | hk 'macao2hk macao macao (b) (10 marks) Simplify your Bursa-Wolf transformation model into the following format through matrix operation. |(1 + 8 macao2x) (1 + 8Smaran2x) Ozma TAX maca21 L-OL -z_maazhk = Lazima -y_macao24 Ox_marok (1 + 8 m2 EL y_macok -Ox_mocaozhat macao () (15 marks) Numerically calculate the 8Smacao2hk so that (1 + 8Shk2macao)-4 in equation (2) can be written as (1 + 8Smacao2hk). Express the 8Smacao2nk in the unit of ppm, same as 8Shk2macao (d) (20 marks) Using the following approximation rule, cos(x) =1 sin(y) 0, (radians) sin((,) sin(x) = 0 Simplify the rotation matrix [R_(Ox hk2maco)]*[Ry@yhk2maco)) *[R (@z,hk2maco) in equation (2) so that it will eventually read like: 1 Oz_macao2hk -Oy macao2nk -Oz_macao2hk 1 Ox_macao2hk Oy.macao2nk -Ox.macao2nk 1 Write the simplified rotation matrix and give the numerical values of 0x macao2nk, Oy.macaoank and Oz macaozik TAX (e) (15 marks) Numerically calculate AY Simplify the LAZ macao2hk (1 + 8Shx2macao)[R: (0x nk?macao)** [R, Cynx2macao) "[R: (0_ nxzmacao)** | AY in Laz'h2macao equation (2) so that it can be simplified as AY Lazimacaoank (1) (6 marks) Write your final 3D coordinate transformation formula for Macao to Hong Kong geodetic coordinates system transformation in the format: 1 @z_macaozhk -By_macao2h = |AY + (1 + SSmacaozhk).- Oz_macao2hk 1 ex_macao2hk ly ) macao hk y macaozhk - 8x macao2hk 1 Y hk macao (g) (6 marks) There is one point r-2362038.05561 = 5417439.3582, calculate its corresponding coordinates 2390772.9223 - hk Y . macao (h) (6 marks) There is one point y 1-2362038.34211 5417429.2380 calculate its corresponding 2390752.8120 macao coordinates y using the formula you obtain in (f). hk











