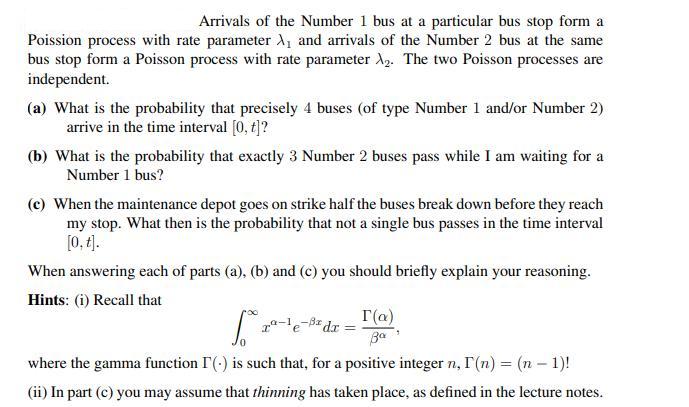
Arrivals of the Number 1 bus at a particular bus stop form a Poission process with rate parameter X, and arrivals of the Number 2 bus at the same bus stop form a Poisson process with rate parameter X. The two Poisson processes are independent. (a) What is the probability that precisely 4 buses (of type Number 1 and/or Number 2) arrive in the time interval [0, t]? (b) What is the probability that exactly 3 Number 2 buses pass while I am waiting for a Number 1 bus? (c) When the maintenance depot goes on strike half the buses break down before they reach my stop. What then is the probability that not a single bus passes in the time interval [0.t]. When answering each of parts (a), (b) and (c) you should briefly explain your reasoning. Hints: (i) Recall that 5 2 -le-Br dr I'(a) 30 where the gamma function I(-) is such that, for a positive integer n, I(n) = (n 1)! (ii) In part (c) you may assume that thinning has taken place, as defined in the lecture notes. Question 2. [10 marks]. Daleks from the planet Skaro are invading Earth to master the planet for their own use. The creator of the Dalek race, Davros, sent out N Daleks to the battlefield on earth to attack humans. Here N is a Poisson random variable with mean X. The earth has its own defence system put up by the Torchwood Institute, which specialises in alien weaponry. Some Daleks in the battle field can be shot down by humans. Assume each Dalek dies independently in the battlefield. Let X; be the lifetime of the ith Dalek in the battlefield, which is a positive random variable with PDF f and CDF F. Let N(t) be the number of Daleks who have died by time t. (a) Find the mean of N (t) for each t > 0. (b) Identify the distribution of N(t) for each t > 0. (c) Show that for any 0 t < t t3 < , N(t) - N(t) and N() - N(t3) are independent. What type of process is N(t)? (d) Let Y; be a Gamma random variable with parameter (a, 3). Assume (Y;) are indepen- dent. Define a compound Poisson process N(t) Y(t) = . i=1 Find E[Y (t)] where t > 0. (e) In the context of part (d), find Cov (Y(s), Y(s+t)) for each s, t > 0. = Question 3. [12 Marks]. Consider a Markov process {X(t)}20 with state space S {0, 1,2,3} and Q-matrix, or genarator, -90 2 0 0 2 -91 0 0 0 0 2 -92 1 -93, Determine the following quantities. (a) The expected holding time of each state (i.e. the expected amount of time spent in each state before a jump). (b) The stationary distribution TQ associated with Q. (c) The proportion of time the process spends in state 3 in the long run. (d) The expected return time, m, for state 2. (e) The transition matrix R of the embedded chain. (f) A stationary distribution of the embedded chain. Is the stationary distribution TR that you found unique? Explain your reasoning. de-At Question 4. [9 Marks]. Recall that the exponential PDF has the form f(t; X) where t, > > 0 and X is known as the rate parameter. Consider a job shop that consists of 3 identical machines and 2 technicians. Suppose that, the amount of time each machine operates before breaking down is exponentially distributed with rate parameter 0.1 and, a technician takes to fix a machine is exponentially distributed with rate parameter 0.4. Sup- pose that all the times to breakdown and times to repair are independent random variables and let X(t) be the number of machines which are operating at time t. (a) Determine the Q-matrix for this Markov process. (b) Write the forward equations involving the Poj(t), j = 0, 1,2, 3, in terms of Pj(t)= Poj(t) = P(X(t) = j|X (0) = 0). (c) Obtain the equilibrium probabilities p;= lim Pj (t). t- = (d) What is the average number of busy technicians in the long-run? [Note: do not expect your answer to be an integer.]