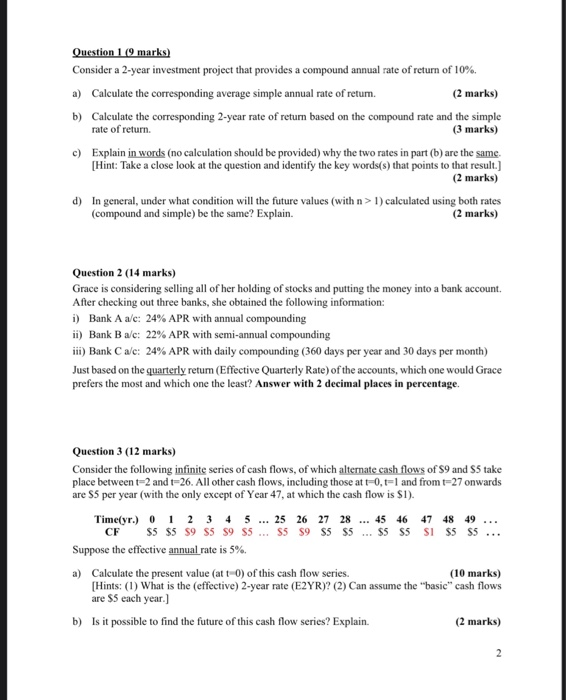
Question 1 (9 marks) Consider a 2-year investment project that provides a compound annual rate of return of 10% a) Calculate the corresponding average simple annual rate of retum. (2 marks) b) Calculate the corresponding 2-year rate of return based on the compound rate and the simple rate of return. (3 marks) c) Explain in words (no calculation should be provided) why the two rates in part (1) are the same [Hint: Take a close look at the question and identify the key words(s) that points to that result.) (2 marks) d) In general, under what condition will the future values (with n > 1) calculated using both rates (compound and simple) be the same? Explain. (2 marks) Question 2 (14 marks) Grace is considering selling all of her holding of stocks and putting the money into a bank account. After checking out three banks, she obtained the following information: i) Bank A a/e: 24% APR with annual compounding ii) Bank B a/c: 22% APR with semi-annual compounding iii) Bank Cale: 24% APR with daily compounding (360 days per year and 30 days per month) Just based on the quarterly retum (Effective Quarterly Rate) of the accounts, which one would Grace prefers the most and which one the least? Answer with 2 decimal places in percentage. Question 3 (12 marks) Consider the following infinite series of cash flows, of which alternate cash flows of $9 and 5 take place between t-2 and t-26. All other cash flows, including those att 0.t-1 and from t27 onwards are S5 per year with the only except of Year 47, at which the cash flow is Sl). Time(yr.) 0 1 2 3 4 5 ... 25 CF S5 S5 S9 S5 S9 S5 ... S5 Suppose the effective annual rate is 5%. 26 S9 27 S5 28 ... 45 46 S5 ... S5 S5 47 SI 48 S5 49 ... S5 ... a) Calculate the present value (att-0) of this cash flow series. (10 marks) [Hints: (1) What is the effective) 2-year rate (E2YR)? (2) Can assume the basic" cash flows are SS cach year.) b) Is it possible to find the future of this cash flow series? Explain. (2 marks) Question 1 (9 marks) Consider a 2-year investment project that provides a compound annual rate of return of 10% a) Calculate the corresponding average simple annual rate of retum. (2 marks) b) Calculate the corresponding 2-year rate of return based on the compound rate and the simple rate of return. (3 marks) c) Explain in words (no calculation should be provided) why the two rates in part (1) are the same [Hint: Take a close look at the question and identify the key words(s) that points to that result.) (2 marks) d) In general, under what condition will the future values (with n > 1) calculated using both rates (compound and simple) be the same? Explain. (2 marks) Question 2 (14 marks) Grace is considering selling all of her holding of stocks and putting the money into a bank account. After checking out three banks, she obtained the following information: i) Bank A a/e: 24% APR with annual compounding ii) Bank B a/c: 22% APR with semi-annual compounding iii) Bank Cale: 24% APR with daily compounding (360 days per year and 30 days per month) Just based on the quarterly retum (Effective Quarterly Rate) of the accounts, which one would Grace prefers the most and which one the least? Answer with 2 decimal places in percentage. Question 3 (12 marks) Consider the following infinite series of cash flows, of which alternate cash flows of $9 and 5 take place between t-2 and t-26. All other cash flows, including those att 0.t-1 and from t27 onwards are S5 per year with the only except of Year 47, at which the cash flow is Sl). Time(yr.) 0 1 2 3 4 5 ... 25 CF S5 S5 S9 S5 S9 S5 ... S5 Suppose the effective annual rate is 5%. 26 S9 27 S5 28 ... 45 46 S5 ... S5 S5 47 SI 48 S5 49 ... S5 ... a) Calculate the present value (att-0) of this cash flow series. (10 marks) [Hints: (1) What is the effective) 2-year rate (E2YR)? (2) Can assume the basic" cash flows are SS cach year.) b) Is it possible to find the future of this cash flow series? Explain. (2 marks)