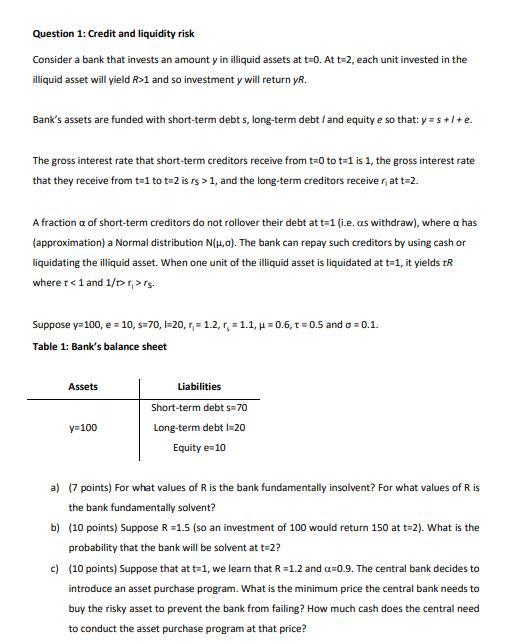
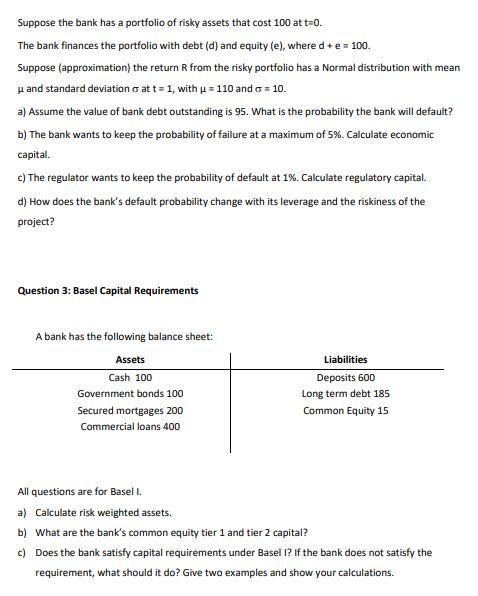
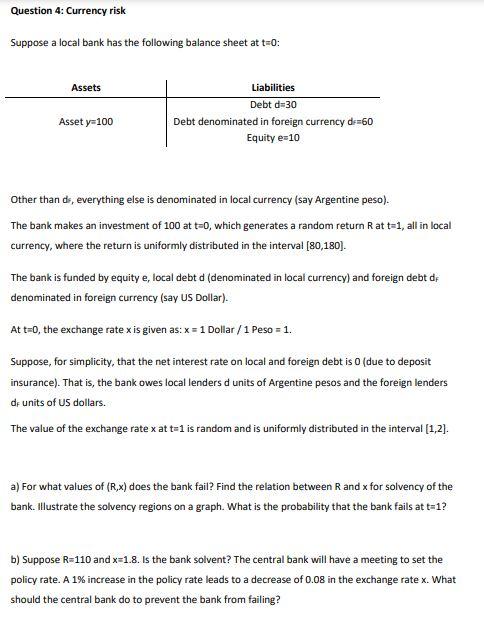
Question 1: Credit and liquidity risk Consider a bank that invests an amount y in illiquid assets at t=0. At t=2, each unit invested in the illiquid asset will yield R>1 and so investment y will return yR. Bank's assets are funded with short-term debts, long-term debt / and equity e so that: y=s+l+e. The gross interest rate that short-term creditors receive from t=0 to t=1 is 1, the gross interest rate that they receive from t=1 to t=2 is rs > 1, and the long-term creditors receiver, at t=2. A fraction a of short-term creditors do not rollover their debt at t=1(i.e. as withdraw), where a has (approximation) a Normal distribution N(1,0). The bank can repay such creditors by using cash or liquidating the illiquid asset. When one unit of the illiquid asset is liquidated at t=1, it yields TR wherer rs. Suppose y=100, e = 10,s=70, I=20, = 1.2, 1, = 1.1, h = 0.6, t = 0.5 and o=0.1. Table 1: Bank's balance sheet Assets Liabilities Short-term debts-70 Long-term debt 120 Equity e-10 y=100 a) (7 points) For what values of R is the bank fundamentally insolvent? For what values of Ris the bank fundamentally solvent? b) (10 points) Suppose R=1.5 (so an investment of 100 would return 150 at t=2). What is the probability that the bank will be solvent at t=2? c) (10 points) Suppose that at t=1, we learn that R=1.2 and a=0.9. The central bank decides to introduce an asset purchase program. What is the minimum price the central bank needs to buy the risky asset to prevent the bank from failing? How much cash does the central need to conduct the asset purchase program at that price? Suppose the bank has a portfolio of risky assets that cost 100 at t=0. The bank finances the portfolio with debt (d) and equity (e), where d + e = 100. Suppose (approximation) the return R from the risky portfolio has a Normal distribution with mean u and standard deviation o att = 1, with u = 110 and o = 10. a) Assume the value of bank debt outstanding is 95. What is the probability the bank will default? b) The bank wants to keep the probability of failure at a maximum of 5%. Calculate economic capital. c) The regulator wants to keep the probability of default at 1%. Calculate regulatory capital. d) How does the bank's default probability change with its leverage and the riskiness of the project? Question 3: Basel Capital Requirements A bank has the following balance sheet: Assets Cash 100 Government bonds 100 Secured mortgages 200 Commercial loans 400 Liabilities Deposits 600 Long term debt 185 Common Equity 15 All questions are for Baselt. a) Calculate risk weighted assets. b) What are the bank's common equity tier 1 and tier 2 capital? c) Does the bank satisfy capital requirements under Basel 1? If the bank does not satisfy the requirement, what should it do? Give two examples and show your calculations. Question 4: Currency risk Suppose a local bank has the following balance sheet at t=0: Assets Liabilities Debt d=30 Debt denominated in foreign currency d=60 Equity e-10 Asset ya 100 Other than ds, everything else is denominated in local currency (say Argentine peso). The bank makes an investment of 100 at t=0, which generates a random return R at t=1, all in local currency, where the return is uniformly distributed in the interval [80,180). The bank is funded by equity e, local debt d (denominated in local currency) and foreign debt de denominated in foreign currency (say US Dollar). At t=0, the exchange rate x is given as: x = 1 Dollar / 1 Peso = 1. Suppose, for simplicity, that the net interest rate on local and foreign debt is o (due to deposit insurance). That is, the bank owes local lenders d units of Argentine pesos and the foreign lenders de units of US dollars. The value of the exchange rate x at t=1 is random and is uniformly distributed in the interval [1,2]. a) For what values of (R,x) does the bank fail? Find the relation between R and x for solvency of the bank. Illustrate the solvency regions on a graph. What is the probability that the bank fails at t=1? b) Suppose R=110 and x=1.8. Is the bank solvent? The central bank will have a meeting to set the policy rate. A 1% increase in the policy rate leads to a decrease of 0.08 in the exchange rate x. What should the central bank do to prevent the bank from failing? Question 1: Credit and liquidity risk Consider a bank that invests an amount y in illiquid assets at t=0. At t=2, each unit invested in the illiquid asset will yield R>1 and so investment y will return yR. Bank's assets are funded with short-term debts, long-term debt / and equity e so that: y=s+l+e. The gross interest rate that short-term creditors receive from t=0 to t=1 is 1, the gross interest rate that they receive from t=1 to t=2 is rs > 1, and the long-term creditors receiver, at t=2. A fraction a of short-term creditors do not rollover their debt at t=1(i.e. as withdraw), where a has (approximation) a Normal distribution N(1,0). The bank can repay such creditors by using cash or liquidating the illiquid asset. When one unit of the illiquid asset is liquidated at t=1, it yields TR wherer rs. Suppose y=100, e = 10,s=70, I=20, = 1.2, 1, = 1.1, h = 0.6, t = 0.5 and o=0.1. Table 1: Bank's balance sheet Assets Liabilities Short-term debts-70 Long-term debt 120 Equity e-10 y=100 a) (7 points) For what values of R is the bank fundamentally insolvent? For what values of Ris the bank fundamentally solvent? b) (10 points) Suppose R=1.5 (so an investment of 100 would return 150 at t=2). What is the probability that the bank will be solvent at t=2? c) (10 points) Suppose that at t=1, we learn that R=1.2 and a=0.9. The central bank decides to introduce an asset purchase program. What is the minimum price the central bank needs to buy the risky asset to prevent the bank from failing? How much cash does the central need to conduct the asset purchase program at that price? Suppose the bank has a portfolio of risky assets that cost 100 at t=0. The bank finances the portfolio with debt (d) and equity (e), where d + e = 100. Suppose (approximation) the return R from the risky portfolio has a Normal distribution with mean u and standard deviation o att = 1, with u = 110 and o = 10. a) Assume the value of bank debt outstanding is 95. What is the probability the bank will default? b) The bank wants to keep the probability of failure at a maximum of 5%. Calculate economic capital. c) The regulator wants to keep the probability of default at 1%. Calculate regulatory capital. d) How does the bank's default probability change with its leverage and the riskiness of the project? Question 3: Basel Capital Requirements A bank has the following balance sheet: Assets Cash 100 Government bonds 100 Secured mortgages 200 Commercial loans 400 Liabilities Deposits 600 Long term debt 185 Common Equity 15 All questions are for Baselt. a) Calculate risk weighted assets. b) What are the bank's common equity tier 1 and tier 2 capital? c) Does the bank satisfy capital requirements under Basel 1? If the bank does not satisfy the requirement, what should it do? Give two examples and show your calculations. Question 4: Currency risk Suppose a local bank has the following balance sheet at t=0: Assets Liabilities Debt d=30 Debt denominated in foreign currency d=60 Equity e-10 Asset ya 100 Other than ds, everything else is denominated in local currency (say Argentine peso). The bank makes an investment of 100 at t=0, which generates a random return R at t=1, all in local currency, where the return is uniformly distributed in the interval [80,180). The bank is funded by equity e, local debt d (denominated in local currency) and foreign debt de denominated in foreign currency (say US Dollar). At t=0, the exchange rate x is given as: x = 1 Dollar / 1 Peso = 1. Suppose, for simplicity, that the net interest rate on local and foreign debt is o (due to deposit insurance). That is, the bank owes local lenders d units of Argentine pesos and the foreign lenders de units of US dollars. The value of the exchange rate x at t=1 is random and is uniformly distributed in the interval [1,2]. a) For what values of (R,x) does the bank fail? Find the relation between R and x for solvency of the bank. Illustrate the solvency regions on a graph. What is the probability that the bank fails at t=1? b) Suppose R=110 and x=1.8. Is the bank solvent? The central bank will have a meeting to set the policy rate. A 1% increase in the policy rate leads to a decrease of 0.08 in the exchange rate x. What should the central bank do to prevent the bank from failing