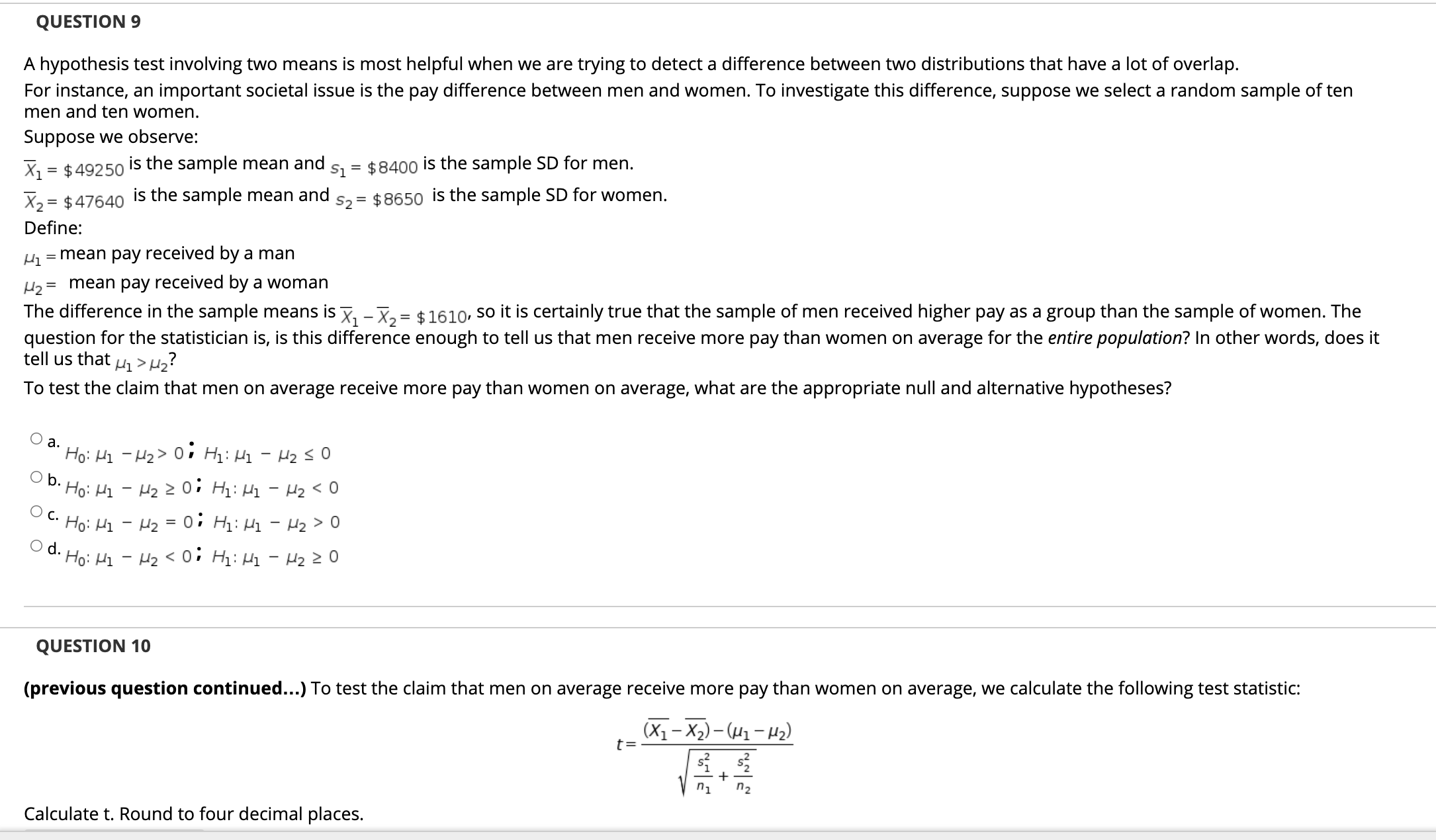
QUESTION 1 Smoking Cessation Programs Among 198 smokers who underwent a "sustained ca re" program, 51 were no longer smoking after six months. Among 199 smokers who underwent a "standard care" program, 30 were no longer smoking after six months (based on data from "Sustained Care Intervention and Postdischarge Smoking Cessation Among Hospitalized Adults," by Rigotti et al., Journal of the American Medical Association, Vol. 312, No.7) Let p1 = the proportion of smokers who no longer smoke six months after undergoing "sustained care". Let p2 = the proportion of smokers who no longer smoke six months after undergoing "standard care". To test the claim that "sustained care" is more effective (i.e., that it leads to a higher proportion of smoking cessation), which of the following are the appropriate null and alternative hypotheses? O a, Ho:p1p2 $0; H1:p1 p2 >0 0 b. H01P1'P2 =0: H1:p1_p2 >0 0 c. HoiP1P2 20$H11P1P2 05H1=P1'P2 50 QUESTION 2 _ X1+X2 (previous question continued...) Given the sample data above, what is the value of the pooled proportion p = ? Round to "1+ n2 four decimal places. QUESTION 3 (previous question continued...) Given the sample data above, what is the value of the test statistic z ? Round to four decimal places. (For best accuracy, do the calculation in Excel with no rounding until the end.) Z = . (P1 -P2) -(P1 - P2) P.q + P.q n1 n2 QUESTION 4 (previous question continued...) Using the test statistic z calculated above, what is the P-value for the sample data provided? Round to four decimal places. QUESTION 5 (previous question continued...) What is the appropriate conclusion, at the 1% significance level? O a. We have sufficient evidence to support the claim that "sustained care" has a higher rate of success than "standard care" at helping smokers to stop smoking. O b. We have insufficient evidence to support the claim that "sustained care" has a higher rate of success than "standard care" at helping smokers to stop smoking. . c. We have sufficient evidence to reject the claim that "sustained care" has a higher rate of success than "standard care" at helping smokers to stop smoking. O d. We have insufficient evidence to reject the claim that "sustained care" has a higher rate of success than "standard care" at helping smokers to stop smoking.QUESTION 6 (previous question continued...) Instead of doing a formal hypothesis test, we can use the sample data to determine a confidence interval for the difference p1- P2 . To do this, we calculate the margin of error E = Za/2 P1 91 , P292 Calculate E for a 99% confidence level. Round to four decimal n1 n2 places. QUESTION 7 (previous question continued...) What is the 99% confidence interval for p1 - p2 at the 99% confidence level? Final values are rounded to three decimal places. O a. (0.003, 0.210) O b. (0.003, 0.211) O c. (0.066, 0.147) O d. (0.067, 0.147) QUESTION 8 (previous question continued...) The fact that the 99% confidence interval only contains positive values indicates what? a. That p1 and p2 are positive, with 99% confidence. O b. That Pi and P2 are positive, with 99% confidence. O c. That p1 is larger than p2, with 99% confidence. O d. That pis larger than p2 , with 99% confidence.QUESTION 9 A hypothesis test involving two means is most helpful when we are trying to detect a difference between two distributions that have a lot of overlap. For instance, an important societal issue is the pay difference between men and women. To investigate this difference, suppose we select a random sample of ten men and ten women. Suppose we observe: X1 = $49250 is the sample mean and s1 = $8400 is the sample SD for men. X2= $47640 is the sample mean and $2 = $8650 is the sample SD for women. Define: M1 = mean pay received by a man H2= mean pay received by a woman The difference in the sample means is X, - X, = $1610, so it is certainly true that the sample of men received higher pay as a group than the sample of women. The question for the statistician is, is this difference enough to tell us that men receive more pay than women on average for the entire population? In other words, does it tell us that 1 > H2! To test the claim that men on average receive more pay than women on average, what are the appropriate null and alternative hypotheses? 0a. Ho: M1 - 12 > 0i H1: M1 - H2 50 Ob. Ho: M - H2 2 0; HI: HI - H2 0 Od . Ho: M1 - 12