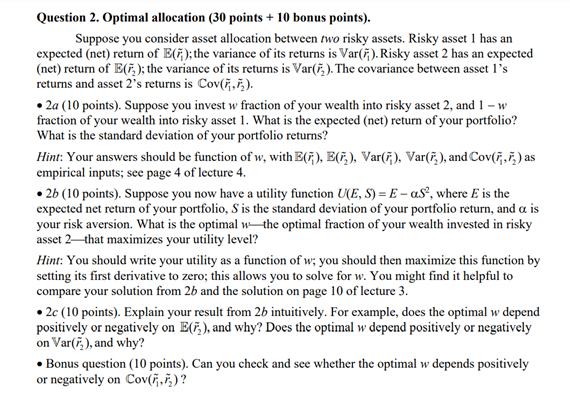
Question 2. Optimal allocation ( 30 points +10 bonus points). Suppose you consider asset allocation between two risky assets. Risky asset 1 has an expected (net) return of E(r~1); the variance of its returns is Var(r~1). Risky asset 2 has an expected (net) return of E(r~2); the variance of its returns is Var(r~2). The covariance between asset l's returns and asset 2 's returns is Cov(r~1,r~2). - 2a (10 points). Suppose you invest w fraction of your wealth into risky asset 2 , and 1w fraction of your wealth into risky asset 1. What is the expected (net) return of your portfolio? What is the standard deviation of your portfolio returns? Hint: Your answers should be function of w, with E(r~1),E(r~2),Var(r~1),Var(r~2), and Cov(r~1,r~2) as empirical inputs; see page 4 of lecture 4. - 2b (10 points). Suppose you now have a utility function U(E,S)=ES2, where E is the expected net return of your portfolio, S is the standard deviation of your portfolio return, and is your risk aversion. What is the optimal w the optimal fraction of your wealth invested in risky asset 2 - that maximizes your utility level? Hint: You should write your utility as a function of w; you should then maximize this function by setting its first derivative to zero; this allows you to solve for w. You might find it helpful to compare your solution from 2b and the solution on page 10 of lecture 3 . - 2c (10 points). Explain your result from 2b intuitively. For example, does the optimal w depend positively or negatively on E(r~2), and why? Does the optimal w depend positively or negatively on Var(r~2), and why? - Bonus question ( 10 points). Can you check and see whether the optimal w depends positively or negatively on Cov(r~1,r~2) ? Question 2. Optimal allocation ( 30 points +10 bonus points). Suppose you consider asset allocation between two risky assets. Risky asset 1 has an expected (net) return of E(r~1); the variance of its returns is Var(r~1). Risky asset 2 has an expected (net) return of E(r~2); the variance of its returns is Var(r~2). The covariance between asset l's returns and asset 2 's returns is Cov(r~1,r~2). - 2a (10 points). Suppose you invest w fraction of your wealth into risky asset 2 , and 1w fraction of your wealth into risky asset 1. What is the expected (net) return of your portfolio? What is the standard deviation of your portfolio returns? Hint: Your answers should be function of w, with E(r~1),E(r~2),Var(r~1),Var(r~2), and Cov(r~1,r~2) as empirical inputs; see page 4 of lecture 4. - 2b (10 points). Suppose you now have a utility function U(E,S)=ES2, where E is the expected net return of your portfolio, S is the standard deviation of your portfolio return, and is your risk aversion. What is the optimal w the optimal fraction of your wealth invested in risky asset 2 - that maximizes your utility level? Hint: You should write your utility as a function of w; you should then maximize this function by setting its first derivative to zero; this allows you to solve for w. You might find it helpful to compare your solution from 2b and the solution on page 10 of lecture 3 . - 2c (10 points). Explain your result from 2b intuitively. For example, does the optimal w depend positively or negatively on E(r~2), and why? Does the optimal w depend positively or negatively on Var(r~2), and why? - Bonus question ( 10 points). Can you check and see whether the optimal w depends positively or negatively on Cov(r~1,r~2)