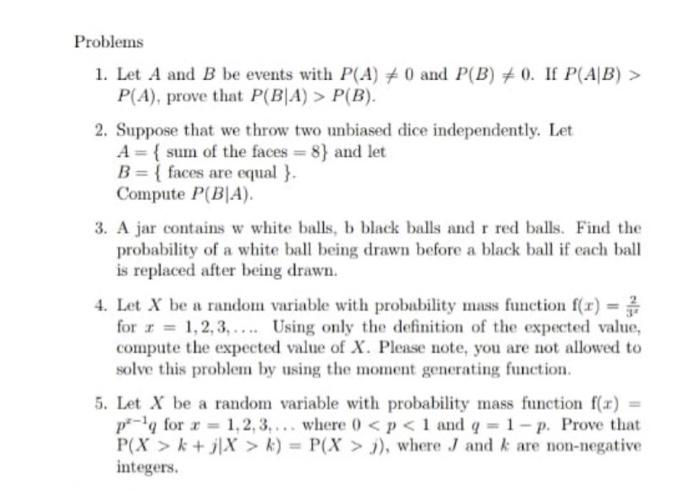
Problems 1. Let A and B be events with P(A) #0 and P(B) 0. If P(A/B) > P(A), prove that P(BA) > P(B). 2. Suppose that we throw two unbiased dice independently. Let A = { sum of the faces = 8} and let B = { faces are equal}. Compute P(BIA). 3. A jar contains w white balls, b black balls and r red balls. Find the probability of a white ball being drawn before a black ball if each ball is replaced after being drawn. 4. Let X be a random variable with probability mass function f(x) = for x = 1,2,3,.... Using only the definition of the expected value, compute the expected value of X. Please note, you are not allowed to solve this problem by using the moment generating function. 5. Let X be a random variable with probability mass function f(x) = pq for x = 1,2,3.... where 0 < p < 1 and q=1-p. Prove that P(X>k+j|X > k) = P(X> j), where J and k are non-negative integers.