 QUESTION 4. Crime Scene Investigator. [Choose the best solution / Write out the step-by-step solution including the script clearly] {Mark: 12; 2, 5, 5} The following information is useful for solving this problem. Newtons Law of Cooling Newtons law of cooling says that the temperature of a body changes at a rate proportional to the difference between its temperature and that of its surrounding medium (the ambient temperature), dT dt kT Ta ( ) where T = the temperature of the body in EC, t = time in hours, the proportionality constant, k = 0.12/hr, Ta = the ambient temperature in EC. Eulers Method New value = Old value + Slope Step size After mastering Numerical Analysis for Engineering, you became a crime scene investigator. Part I. Which of the following two items are most appropriate for you to carry along your watch while investigating a crime scene? [Circle only 2; otherwise you will be graded according to the number of wrong choices you pick]. A) a bottle of water B) a bungee jumping rope C) a calculator D) a candle E) a compass F) a copy of the textbook for NAE G) a mirror H) a parachute I) a temperature sensor J) a toothbrush You were called to investigate a murder. You arrived at the crime scene at 6:00 am on January 30 and immediately took the temperature of the homicide victim and found the body to be at EC, where, = 2 + average of the last 4 digits of your student ID; e.g., 107 333 456 = 2 + (3+4+5+6)/4 = 6
QUESTION 4. Crime Scene Investigator. [Choose the best solution / Write out the step-by-step solution including the script clearly] {Mark: 12; 2, 5, 5} The following information is useful for solving this problem. Newtons Law of Cooling Newtons law of cooling says that the temperature of a body changes at a rate proportional to the difference between its temperature and that of its surrounding medium (the ambient temperature), dT dt kT Ta ( ) where T = the temperature of the body in EC, t = time in hours, the proportionality constant, k = 0.12/hr, Ta = the ambient temperature in EC. Eulers Method New value = Old value + Slope Step size After mastering Numerical Analysis for Engineering, you became a crime scene investigator. Part I. Which of the following two items are most appropriate for you to carry along your watch while investigating a crime scene? [Circle only 2; otherwise you will be graded according to the number of wrong choices you pick]. A) a bottle of water B) a bungee jumping rope C) a calculator D) a candle E) a compass F) a copy of the textbook for NAE G) a mirror H) a parachute I) a temperature sensor J) a toothbrush You were called to investigate a murder. You arrived at the crime scene at 6:00 am on January 30 and immediately took the temperature of the homicide victim and found the body to be at EC, where, = 2 + average of the last 4 digits of your student ID; e.g., 107 333 456 = 2 + (3+4+5+6)/4 = 6
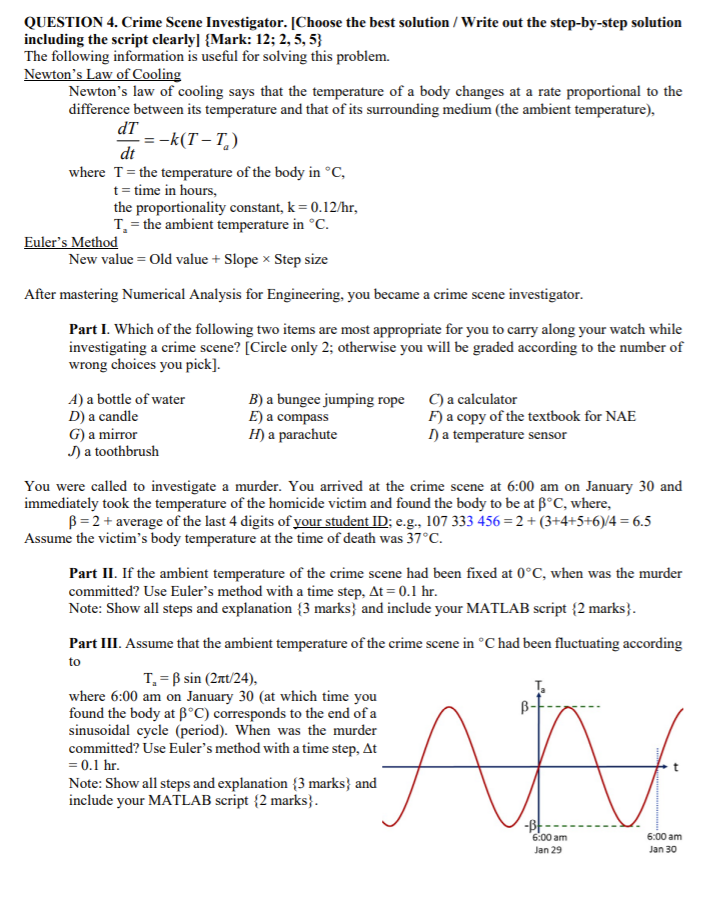
QUESTION 4. Crime Scene Investigator. (Choose the best solution /Write out the step-by-step solution including the script clearly] {Mark: 12; 2,5,5} The following information is useful for solving this problem. Newton's Law of Cooling Newton's law of cooling says that the temperature of a body changes at a rate proportional to the difference between its temperature and that of its surrounding medium (the ambient temperature), dT "=-k(T-T.) dt where T = the temperature of the body in C, t = time in hours, the proportionality constant, k=0.12/hr, T = the ambient temperature in C. Euler's Method New value = Old value + Slope Step size After mastering Numerical Analysis for Engineering, you became a crime scene investigator. Part I. Which of the following two items are most appropriate for you to carry along your watch while investigating a crime scene? (Circle only 2; otherwise you will be graded according to the number of wrong choices you pick]. A) a bottle of water B) a bungee jumping rope ) a calculator D) a candle E) a compass F) a copy of the textbook for NAE G) a mirror H) a parachute 1) a temperature sensor J) a toothbrush You were called to investigate a murder. You arrived at the crime scene at 6:00 am on January 30 and immediately took the temperature of the homicide victim and found the body to be at BC, where, B = 2 + average of the last 4 digits of your student ID; e.g., 107 333 456 = 2 + (3+4+5+6)/4= 6.5 Assume the victim's body temperature at the time of death was 37C. Part II. If the ambient temperature of the crime scene had been fixed at 0C, when was the murder committed? Use Euler's method with a time step, At=0.1 hr. Note: Show all steps and explanation {3 marks; and include your MATLAB script {2 marks). Part III. Assume that the ambient temperature of the crime scene in C had been fluctuating according to T = B sin (2nt/24), where 6:00 am on January 30 (at which time you found the body at BC) corresponds to the end of a B- sinusoidal cycle (period). When was the murder committed? Use Euler's method with a time step, At = 0.1 hr. Note: Show all steps and explanation {3 marks) and include your MATLAB script {2 marks). -BH AN t 6:00 am Jan 29 6:00 am Jan 30 QUESTION 4. Crime Scene Investigator. (Choose the best solution /Write out the step-by-step solution including the script clearly] {Mark: 12; 2,5,5} The following information is useful for solving this problem. Newton's Law of Cooling Newton's law of cooling says that the temperature of a body changes at a rate proportional to the difference between its temperature and that of its surrounding medium (the ambient temperature), dT "=-k(T-T.) dt where T = the temperature of the body in C, t = time in hours, the proportionality constant, k=0.12/hr, T = the ambient temperature in C. Euler's Method New value = Old value + Slope Step size After mastering Numerical Analysis for Engineering, you became a crime scene investigator. Part I. Which of the following two items are most appropriate for you to carry along your watch while investigating a crime scene? (Circle only 2; otherwise you will be graded according to the number of wrong choices you pick]. A) a bottle of water B) a bungee jumping rope ) a calculator D) a candle E) a compass F) a copy of the textbook for NAE G) a mirror H) a parachute 1) a temperature sensor J) a toothbrush You were called to investigate a murder. You arrived at the crime scene at 6:00 am on January 30 and immediately took the temperature of the homicide victim and found the body to be at BC, where, B = 2 + average of the last 4 digits of your student ID; e.g., 107 333 456 = 2 + (3+4+5+6)/4= 6.5 Assume the victim's body temperature at the time of death was 37C. Part II. If the ambient temperature of the crime scene had been fixed at 0C, when was the murder committed? Use Euler's method with a time step, At=0.1 hr. Note: Show all steps and explanation {3 marks; and include your MATLAB script {2 marks). Part III. Assume that the ambient temperature of the crime scene in C had been fluctuating according to T = B sin (2nt/24), where 6:00 am on January 30 (at which time you found the body at BC) corresponds to the end of a B- sinusoidal cycle (period). When was the murder committed? Use Euler's method with a time step, At = 0.1 hr. Note: Show all steps and explanation {3 marks) and include your MATLAB script {2 marks). -BH AN t 6:00 am Jan 29 6:00 am Jan 30
 QUESTION 4. Crime Scene Investigator. [Choose the best solution / Write out the step-by-step solution including the script clearly] {Mark: 12; 2, 5, 5} The following information is useful for solving this problem. Newtons Law of Cooling Newtons law of cooling says that the temperature of a body changes at a rate proportional to the difference between its temperature and that of its surrounding medium (the ambient temperature), dT dt kT Ta ( ) where T = the temperature of the body in EC, t = time in hours, the proportionality constant, k = 0.12/hr, Ta = the ambient temperature in EC. Eulers Method New value = Old value + Slope Step size After mastering Numerical Analysis for Engineering, you became a crime scene investigator. Part I. Which of the following two items are most appropriate for you to carry along your watch while investigating a crime scene? [Circle only 2; otherwise you will be graded according to the number of wrong choices you pick]. A) a bottle of water B) a bungee jumping rope C) a calculator D) a candle E) a compass F) a copy of the textbook for NAE G) a mirror H) a parachute I) a temperature sensor J) a toothbrush You were called to investigate a murder. You arrived at the crime scene at 6:00 am on January 30 and immediately took the temperature of the homicide victim and found the body to be at EC, where, = 2 + average of the last 4 digits of your student ID; e.g., 107 333 456 = 2 + (3+4+5+6)/4 = 6
QUESTION 4. Crime Scene Investigator. [Choose the best solution / Write out the step-by-step solution including the script clearly] {Mark: 12; 2, 5, 5} The following information is useful for solving this problem. Newtons Law of Cooling Newtons law of cooling says that the temperature of a body changes at a rate proportional to the difference between its temperature and that of its surrounding medium (the ambient temperature), dT dt kT Ta ( ) where T = the temperature of the body in EC, t = time in hours, the proportionality constant, k = 0.12/hr, Ta = the ambient temperature in EC. Eulers Method New value = Old value + Slope Step size After mastering Numerical Analysis for Engineering, you became a crime scene investigator. Part I. Which of the following two items are most appropriate for you to carry along your watch while investigating a crime scene? [Circle only 2; otherwise you will be graded according to the number of wrong choices you pick]. A) a bottle of water B) a bungee jumping rope C) a calculator D) a candle E) a compass F) a copy of the textbook for NAE G) a mirror H) a parachute I) a temperature sensor J) a toothbrush You were called to investigate a murder. You arrived at the crime scene at 6:00 am on January 30 and immediately took the temperature of the homicide victim and found the body to be at EC, where, = 2 + average of the last 4 digits of your student ID; e.g., 107 333 456 = 2 + (3+4+5+6)/4 = 6





