Question:

Hello! I do not understand what the following question asks for a. subquestion. I feel confused. Can someone provide me with a detailed explanation? Thank you!

1. Go to the All Locations worksheet, where Benicio wants to summarize the quarterly and annual totals from the three locations for each type of product.

Consolidate the sales data from the three locations as follows:

a. In cell B5, enter a formula using the SUM function and 3-D references that totals the Mini sales values (cell B5) in Quarter 1 from the U.S., Canada, and Mexico worksheets.

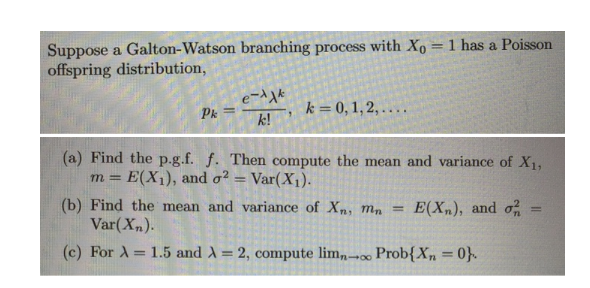
Question 2 (a) A particle executes an unrestricted random walk on the line starting at the origin The ith step, Z, has the following distribution P(Z: = 2) = p. P(Z = 5) = q= 1p Find the mean of Z, (A) 6p -3 B) Sp - 5 (9) 7p - 5 (D) 6p -4 (b) In a Galton-Watson branching process starting with a single individual in generation zero, the offspring distribution is binomial B(3, 0.5). Calculate the variance of the number of individual in the second generation. Provide your answer in three decimal places.Qs.3 [25 pts] a. If a Random Process (RP) is 5" order SSS: - Is it also 2nd order SSS? Is it also WSS? b. Given two RPs X(t) and Y(t), define what it means for them to be - Uncorrelated - Orthogonal - Independent c. What makes a Random Process a Gauss-Markov process? d. Using appropriate sketches and equations, describe what it means to say a WSS RP is also Ergodic - i.e. time averages equal ensemble averages. Your answer should not exceed a page including figures e. A real valued RP X(t) has a PSD Sex(f) i. What are the units for the PSD? Sketch an arbitrary (but valid) PSD and indicate how you would compute the power in the RP for a limited band of frequencies, say from f, to f2Question 7 Each of the probability distributions listed in the options below is the offspring distribution for a Galton- Watson branching process. Select the THREE options that give distributions for which eventual extinction of the branching process is possible but not certain. Select one or more: Poisson(1.7) D GI() O B(8, 0.125) B(6, 0.2) O Modified geometric with parameters a = 6, b = 2, c = 9 and d = 5 O Negative binomial with range {0,1,...} and parameters r = 5 and p = 0.2Suppose a Galton-Watson branching process with Xo = 1 has a Poisson offspring distribution, e Pk k = 0, 1, 2, ... . k! (a) Find the p.g.f. f. Then compute the mean and variance of X1, m = E(X,), and o? = Var(X1). (b) Find the mean and variance of Xn, mn = E(Xn), and on = Var(Xn). (c) For A = 1.5 and A = 2, compute lim,- Prob{ X, = 0}