Question: QUESTION FOUR (a) Express the function f(x) = (1, when |x| 1 10, when |x|>1 as a Fourier Integral, hence evaluate sin cos x

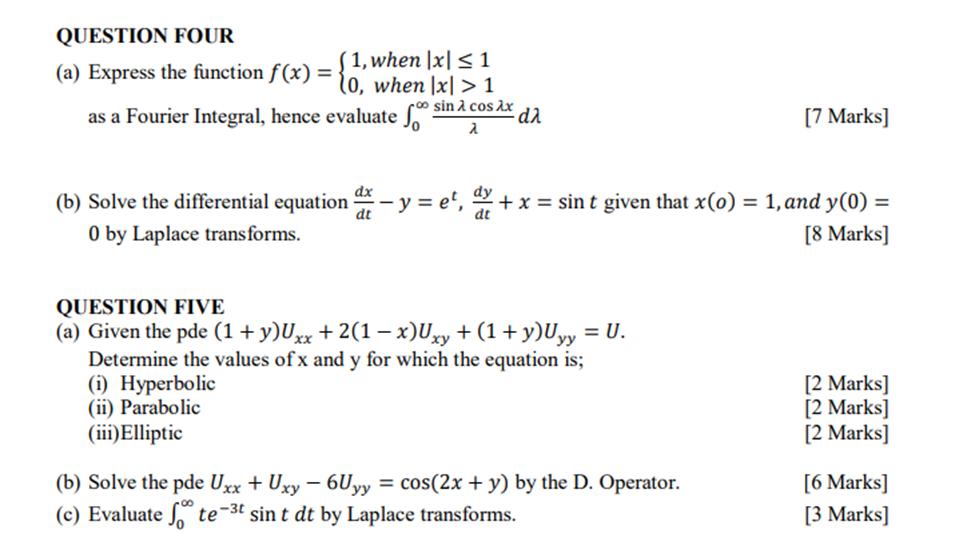
QUESTION FOUR (a) Express the function f(x) = (1, when |x| 1

QUESTION FOUR (a) Express the function f(x) = (1, when |x| 1 10, when |x|>1 as a Fourier Integral, hence evaluate sin cos x d (i) Hyperbolic (ii) Parabolic (iii) Elliptic dx (b) Solve the differential equation - y = et, + x = sin t given that x(o) = 1, and y(0) = dt dt 0 by Laplace transforms. [8 Marks] QUESTION FIVE (a) Given the pde (1 + y)Uxx + 2(1 x)Uxy + (1+y)Uyy = U. Determine the values of x and y for which the equation is; [7 Marks] (b) Solve the pde Uxx + Uxy - 6Uyy = cos(2x + y) by the D. Operator. (c) Evaluate te-3t sin t dt by Laplace transforms. [2 marks] [2 marks] [2 marks] [6 Marks] [3 Marks]

Step by Step Solution

★★★★★

3.49 Rating (152 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

The Fourier integral representation of fx is given by fx 12 infinity infinity Feixd where F is the Fourier transform of fx To evaluate the integral we can use the following properties of the Fourier t... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Old MathJax webview First read the "Hawley Lighting Company" case study. Conduct necessary calculations and answer the questions listed below. Submit your Excel spreadsheet(s) with calculations. Part...

Determine the values of KM and Vmax for the de-carboxylation of a -keto acid given the following data. Substrate Concentration (mol L1) Velocity (mM min-1) 2.500.................. 0.588...

Determine the values of the diffusion coefficients for the interdiffusion of carbon in both iron (BCC) and iron (FCC) at 900C Which is larger Explain why this is the case

Determine the values of x=(x 2 x 2 ) 1/2 and p=(p 2 p 2 ) 1/2 for the ground state of (a) A particle in a box of length L (b) An harmonic oscillator. Discuss these quantities with reference to the...

Ted, a project manager, wants to invest in a project with an initial cost of $58,500 and cash flows of $32,400 and $38,500 in Years 1 and 2. Rosita, his boss, requires a discount rate of 10 percent...

1. 3 2 Consider the function f(x) = x - 3x - 9x + 10, x (a) . Find the equation of the straight line passing through the maximum and minimum points of the graph y = f(x). (4) (b) Show that the point...

Please answer the following questions by showing all work and providing explanation when needed- thank you. 6. (5 pts.) Use a method of your choice to determine if the series converges absolutely,...

Question 3 Graph the given function f x 1 x 1 3 5 4 3 2 54 4 3 2 1 0 1 c W N 4 2 3 4 5 X H 1 2 3 4 5

College Algebra MATH 107 Summer, 2017, V1.8 MATH 107 FINAL EXAMINATION This is an open-book exam. You may refer to your text and other course materials as you work on the exam, and you may use a...

College Algebra MATH 107 Spring, 2017, V4.9 MATH 107 FINAL EXAMINATION This is an open-book exam. You may refer to your text and other course materials as you work on the exam, and you may use a...

Math 107 Final Examination Fall, 2017 1 Math 107 College Algebra Name______________________________ Final Examination: Fall, 2017 Instructor __________________________ Answer Sheet Instructions: This...

A 5.9 ft-tall girl stands on level ground. The sun is 30 degrees above the horizon. How long is her shadow? l=? ft

(a) Using dissociation constants from Appendix D, determine the value for the equilibrium constant for each of the following reactions. (b) We usually use single arrows for reactions when the forward...

What determines if measures reported to CMS on behalf of the Hospital Value - Based Purchasing Program are considered valid?

1) Air at 1 atm and 15C with a mass flow rate at 0.15 kg/s flows through a long rectangular duct 7.5 cm by 15 cm. A section of the duct is maintained at 120C, and the average air temperature at exit...

On January 1, Year 1, Shannon Corporation (SC) acquired 70 percent (70,000 shares of $2 par common stock) of Kanada Corporation (KC) for $30 per share. Shortly after the acquisition the remaining KC...

David Wong, the product manager of KiKi Company, was reviewing the production schedule for the last quarter of 2020. He noted that the company planned to sell 4,000 units during the year and keep a...

Give the two isomers of [Ru(CO):(k-dppe)]. Draw them using wedge dash diagrams and assign the exact point group to each of them. Using group theory ( i.e. character tables and the reducing formula to...

3-2. Two long cylindrical shells of radii ra and r, sre arranged coaxially and are charged to the potentials , and U, respectively. Find the potential at points between the eylindrical shelis.

28. What is the term for whole units that could have been produced in a period given the amount of manufacturing inputs used? a. FIFO units. b. Transferred in units. c. Weighted average units. d....

Find the adjacency matrix of: (3) 4) (2) (1)

Evaluate the integral for the given data. Describe the kind of surface. Show the details of your work. F = [y 2 , x 2 , z 4 ], S: z = 4 x 2 + y 2 , 0 z 8, y 0

Let z = x + iy. Showing details, find, in terms of x and y: Im (1/z 2 )

5.3 Summarize contemporary views about self-concepts across cultures.

1. Piaget describes two ways that we learn: through assimilation and accommodation. Give an example of each process. For instance, think about a new piece of information that you have learned. Did...

Are there cultural variations within, rather than between, Piagets stages? There is considerable cultural variation in the order in which children acquire specific skills within Piagets stages. In a...