Questions on microeconomics, try and provide a solutions for these

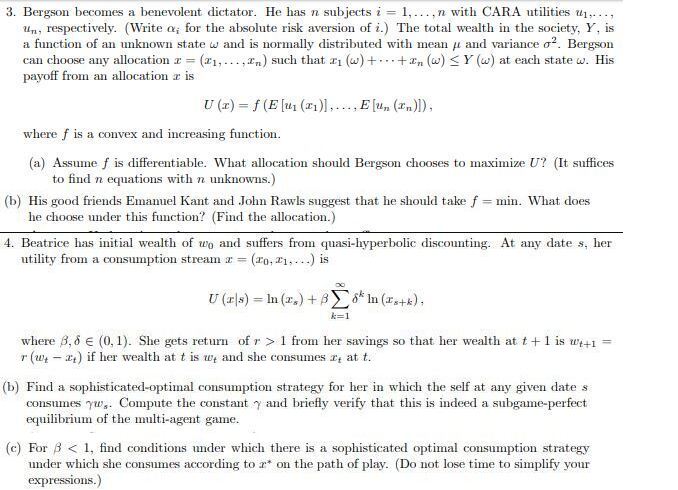
Consider an economy with two types of individuals: skilled and unskilled workers. The only differ- ence between the two is that the skilled have a higher hourly wage w. = 40 than the unskilled do, wu = 10. Suppose that there are 400 unskilled and 100 skilled workers in this economy. Suppose that each individual has a utility function over consumption (c) and leisure (1) of the following form: U(c, 1) = In(c) + 2In(1) where I E [0, 24]. 1. (a) (3 minutes) Write down the individual's budget constraint in terms of consumption and leisure. Draw the budget constraints for the skilled and unskilled workers in the same graph with leisure on the r-axis. (b) (7 minutes) Solve for each individual's optimal leisure, labor, and consumption choice. 2. (10 minutes) Now suppose that the government wants to redistribute from the skilled to the unskilled workers. It levies an income tax which collects 20% of each skilled worker's earnings and then uses the tax revenue to give an equal amount (lump-sum transfer) T to each unskilled worker. So only the skilled workers are taxed and only the unskilled workers receive the transfer. (a) (4 minutes) On the same set of axes, draw the new budget constraints faced by the two types of individuals. (b) (4 minutes) Solve for each skilled individual's new optimal leisure, labor, and consump- tion. Does labor supply change? What is the intuition behind this result? (c) (2 minutes) Compute the total tax revenue collected by the government from taxing the skilled individuals. 3. (a) (4 minutes) Suppose that for every tax dollar collected, 6.25 cents are lost due to admin- istrative costs. Suppose that the government sets 7 so that it spends what it collects (the government balances its budget). How large is T"?3. Bergson becomes a benevolent dictator. He has a subjects i = 1,... .n with CARA utilities w1...., un, respectively. (Write o, for the absolute risk aversion of i.) The total wealth in the society, Y, is a function of an unknown state w and is normally distributed with mean / and variance of. Bergson can choose any allocation r = (21, ... .2,) such that an (@) + ... +2, (w) I from her savings so that her wealth at t + 1 is my+1 = " (wt - 2,) if her wealth at t is wy and she consumes r, at t. (b) Find a sophisticated-optimal consumption strategy for her in which the self at any given date s consumes yes. Compute the constant y and briefly verify that this is indeed a subgame-perfect equilibrium of the multi-agent game. (c) For 8