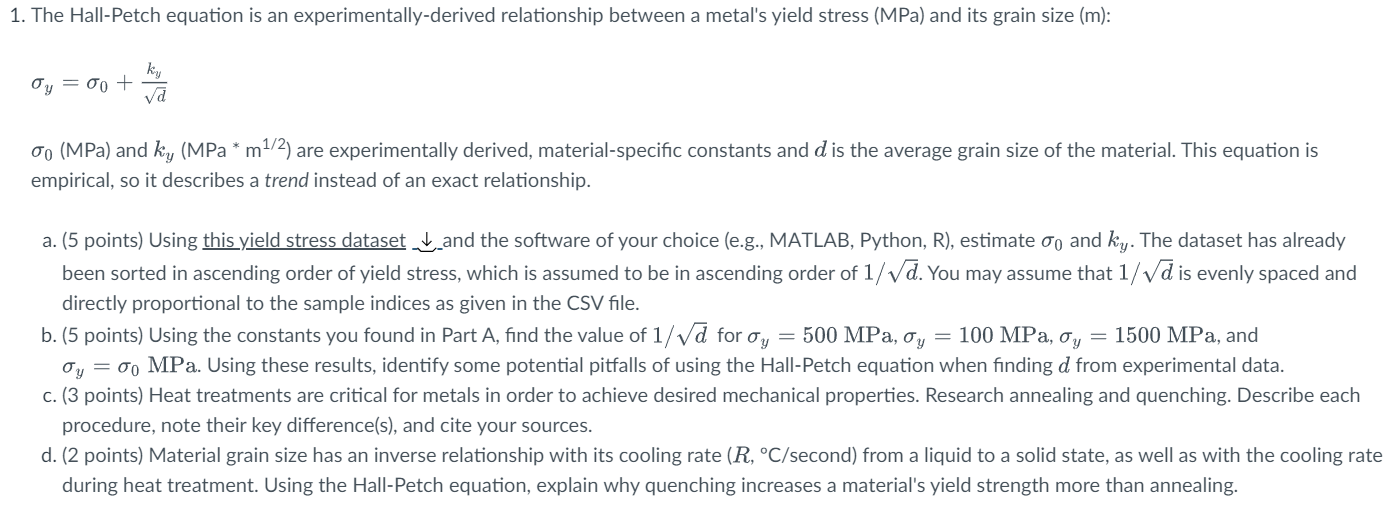
Sample Yield Stres 1 216.1279 2 342.9647 3 355.9578 4 387.4022 5 396.8129 6 399.8067 7 406.4461 8 407.5466 9 411.867 10 414.4823 11 423.4072 12 426.9947 13 431.1878 14 436.0694 15 442.7782 16 443.8467 17 444.8881 18 454.2986 19 456.6522 20 457.1242 21 457.1315 22 460.6629 23 461.6712 24 463.042 25 465.1413 26 466.8928 27 468.23 28 468.532 29 469.3857 30 469.9638 31 472.6856 32 478.0172 33 478.3248 34 482.0975 35 482.9827 36 483.4894 37 485.1821 38 486.6637 39 487.9177 40 488.5264 41 491.6955 42 493.0948 43 493.9317 44 495.5067 45 498.1727 46 501.0211 47 502.7543 48 504.9957 49 506.4135 50 507.2008 51 507.4456 52 507.5306 53 509.4897 54 509.6727 55 511.0985 56 511.3595 57 512.1312 58 512.2677 59 515.0216 60 517.8128 61 518.8252 62 519.059 63 519.0973 64 519.8048 65 520.0699 66 520.99 67 521.9532 68 523.8716 69 524.2986 70 524.9364 71 524.9762 72 525.1625 73 527.7768 74 528.8571 75 529.655 76 530.2236 77 532.3625 78 532.9899 79 534.3595 80 534.7791 81 535.7608 82 537.1099 83 537.3127 84 539.3926 85 542.9539 86 543.4836 87 543.5537 88 545.1256 89 545.742 90 546.1774 91 546.1895 92 546.382 93 546.8731 94 547.1258 95 547.3154 96 549.5162 97 552.1307 98 553.2879 99 554.0379 100 554.1526 101 555.9184 102 560.4668 103 565.1205 104 567.2018 105 568.2772 106 568.9874 107 569.0359 108 569.4456 109 570.9067 110 575.0549 111 576.0115 112 577.3732 113 581.4008 114 581.9379 115 583.6227 116 584.3436 117 585.9864 118 586.1091 119 587.0526 120 587.6937 121 588.7976 122 589.9794 123 590.1085 124 590.2123 125 591.0318 126 596.6888 127 598.7609 128 602.4406 129 604.6087 130 604.9004 131 609.5547 132 618.0842 133 618.8581 134 627.4909 135 638.6223 1. The Hall-Petch equation is an experimentally-derived relationship between a metal's yield stress (MPa) and its grain size (m): ky Oy = 00 + va * 00 (MPa) and ky (MPa* m1/2) are experimentally derived, material-specific constants and dis the average grain size of the material. This equation is empirical, so it describes a trend instead of an exact relationship. V a. (5 points) Using this yield stress dataset x and the software of your choice (e.g., MATLAB, Python, R), estimate oo and ky. The dataset has already been sorted in ascending order of yield stress, which is assumed to be in ascending order of 1/ Vd. You may assume that 1/Vd is evenly spaced and directly proportional to the sample indices as given in the CSV file. b. (5 points) Using the constants you found in Part A, find the value of 1/ Vd for Oy = 500 MPa, Oy = 100 MPa, Oy = 1500 MPa, and Oy = 00 MPa. Using these results, identify some potential pitfalls of using the Hall-Petch equation when finding d from experimental data. c. (3 points) Heat treatments are critical for metals in order to achieve desired mechanical properties. Research annealing and quenching. Describe each procedure, note their key difference(s), and cite your sources. d. (2 points) Material grain size has an inverse relationship with its cooling rate (R, C/second) from a liquid to a solid state, as well as with the cooling rate during heat treatment. Using the Hall-Petch equation, explain why quenching increases a material's yield strength more than annealing. Sample Yield Stres 1 216.1279 2 342.9647 3 355.9578 4 387.4022 5 396.8129 6 399.8067 7 406.4461 8 407.5466 9 411.867 10 414.4823 11 423.4072 12 426.9947 13 431.1878 14 436.0694 15 442.7782 16 443.8467 17 444.8881 18 454.2986 19 456.6522 20 457.1242 21 457.1315 22 460.6629 23 461.6712 24 463.042 25 465.1413 26 466.8928 27 468.23 28 468.532 29 469.3857 30 469.9638 31 472.6856 32 478.0172 33 478.3248 34 482.0975 35 482.9827 36 483.4894 37 485.1821 38 486.6637 39 487.9177 40 488.5264 41 491.6955 42 493.0948 43 493.9317 44 495.5067 45 498.1727 46 501.0211 47 502.7543 48 504.9957 49 506.4135 50 507.2008 51 507.4456 52 507.5306 53 509.4897 54 509.6727 55 511.0985 56 511.3595 57 512.1312 58 512.2677 59 515.0216 60 517.8128 61 518.8252 62 519.059 63 519.0973 64 519.8048 65 520.0699 66 520.99 67 521.9532 68 523.8716 69 524.2986 70 524.9364 71 524.9762 72 525.1625 73 527.7768 74 528.8571 75 529.655 76 530.2236 77 532.3625 78 532.9899 79 534.3595 80 534.7791 81 535.7608 82 537.1099 83 537.3127 84 539.3926 85 542.9539 86 543.4836 87 543.5537 88 545.1256 89 545.742 90 546.1774 91 546.1895 92 546.382 93 546.8731 94 547.1258 95 547.3154 96 549.5162 97 552.1307 98 553.2879 99 554.0379 100 554.1526 101 555.9184 102 560.4668 103 565.1205 104 567.2018 105 568.2772 106 568.9874 107 569.0359 108 569.4456 109 570.9067 110 575.0549 111 576.0115 112 577.3732 113 581.4008 114 581.9379 115 583.6227 116 584.3436 117 585.9864 118 586.1091 119 587.0526 120 587.6937 121 588.7976 122 589.9794 123 590.1085 124 590.2123 125 591.0318 126 596.6888 127 598.7609 128 602.4406 129 604.6087 130 604.9004 131 609.5547 132 618.0842 133 618.8581 134 627.4909 135 638.6223 1. The Hall-Petch equation is an experimentally-derived relationship between a metal's yield stress (MPa) and its grain size (m): ky Oy = 00 + va * 00 (MPa) and ky (MPa* m1/2) are experimentally derived, material-specific constants and dis the average grain size of the material. This equation is empirical, so it describes a trend instead of an exact relationship. V a. (5 points) Using this yield stress dataset x and the software of your choice (e.g., MATLAB, Python, R), estimate oo and ky. The dataset has already been sorted in ascending order of yield stress, which is assumed to be in ascending order of 1/ Vd. You may assume that 1/Vd is evenly spaced and directly proportional to the sample indices as given in the CSV file. b. (5 points) Using the constants you found in Part A, find the value of 1/ Vd for Oy = 500 MPa, Oy = 100 MPa, Oy = 1500 MPa, and Oy = 00 MPa. Using these results, identify some potential pitfalls of using the Hall-Petch equation when finding d from experimental data. c. (3 points) Heat treatments are critical for metals in order to achieve desired mechanical properties. Research annealing and quenching. Describe each procedure, note their key difference(s), and cite your sources. d. (2 points) Material grain size has an inverse relationship with its cooling rate (R, C/second) from a liquid to a solid state, as well as with the cooling rate during heat treatment. Using the Hall-Petch equation, explain why quenching increases a material's yield strength more than annealing