SECTION 5.1 REFERENCE:

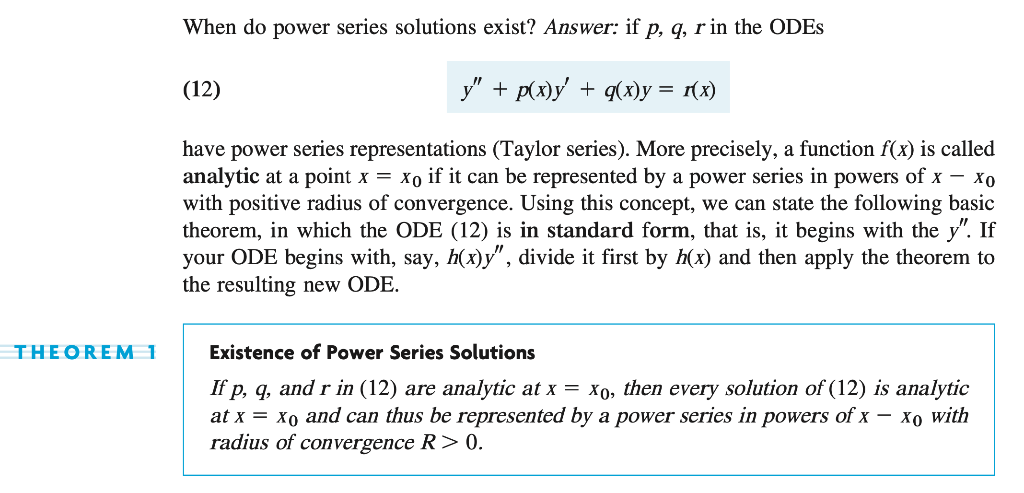
Please neatly show all work. Thank you!

Problem 1. Does this ODE x+y" + (3x 1)y' + y = 0 have such a power series solution y = En anxwith radius of convergence R > 0? If so, please find it; if not, please prove your judgement and explain why this does not contradict the main theorem in Section 5.1. (Hint: Recall Cauchy - Hadamard formula: 1 R lim n-00 an+1 an provided the limit exists (can be infinity).) When do power series solutions exist? Answer: if p, q, r in the ODES (12) y + 2xy + g(x)) = (x) have power series representations (Taylor series). More precisely, a function f(x) is called analytic at a point x = xo if it can be represented by a power series in powers of x Xo with positive radius of convergence. Using this concept, we can state the following basic theorem, in which the ODE (12) is in standard form, that is, it begins with the y". If your ODE begins with, say, h(x))", divide it first by h(x) and then apply the theorem to the resulting new ODE. THEOREM 1 Existence of Power Series Solutions If p, q, and r in (12) are analytic at x = Xo, then every solution of (12) is analytic at x = Xo and can thus be represented by a power series in powers of x Xo with radius of convergence R>0