


..solve the following questions.,
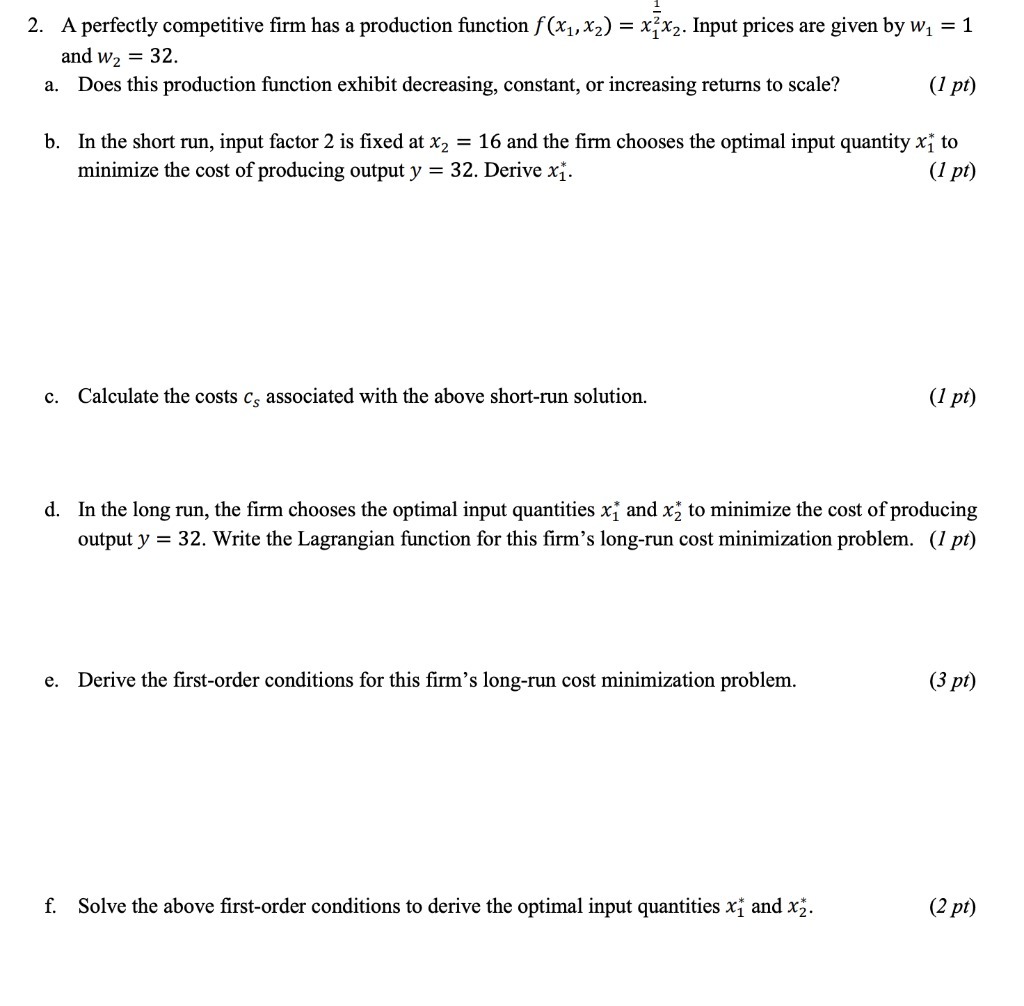
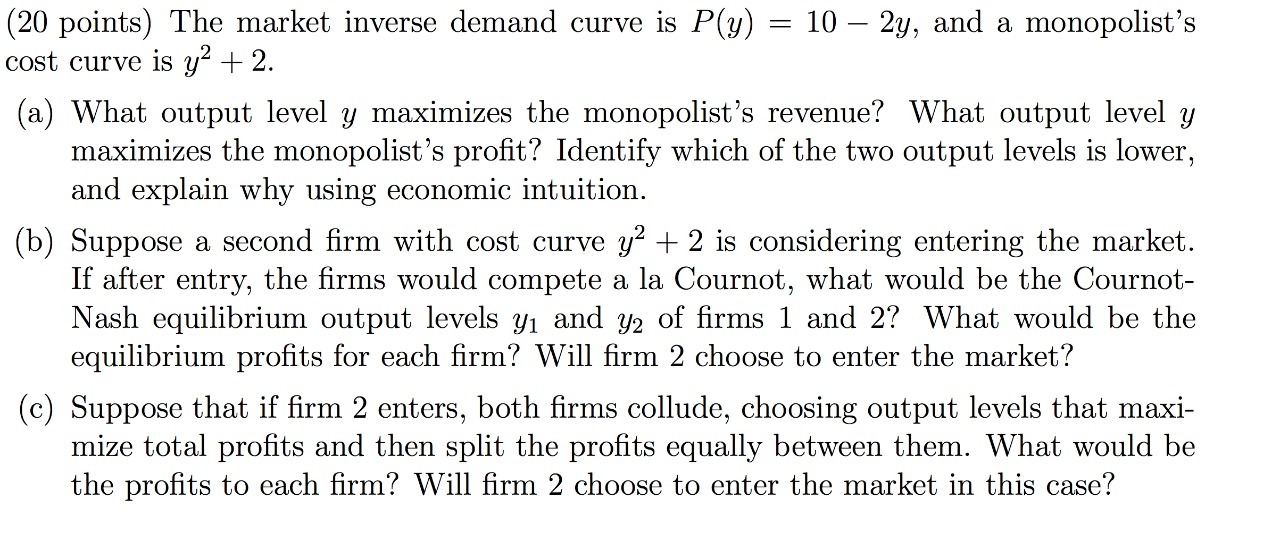
(15 points) Suppose the consumer considers goods 1 and 2 to be perfect complements, so that her utility function is \"($1,132) = min{:r1, .132}. What is her demand fer goods 1 and 2 as a function of income so and prices p1 and 332? Show the derivationj rather than just writing down the demand function if you have it memorized. 1 2. Aperfectly competitive rm has a production function f(x1, x2) = xix? Input prices are given by W1 = 1 b. and WE = 32. Does this production function exhibit decreasing, constant, or increasing returns to scale? (I pt) In the short run, input factor 2 is xed at x2 = 16 and the rm chooses the optimal input quantity x; to minimize the cost of producing output 3: = 32. Derive xi\". (1 pt) Calcalate the costs cs associated with the above short-run solution. (1 pt) In the long run, the rm chooses the optimal input quanties x; and x; to minimize the cost of producing output y = 32. Write the Lagrangian function for this rm's long-run cost minimization problem. (I pt) Derive the rst-order conditions for this rm's long-run cost minimization problem. (3 pt) Solve the above rst-order conditions to derive the optimal input quantities x; and x3. (2 pt) 1 Externalities Problem 1 Answer questions (a) and (b) for each of the following four examples: 1. Smoking by individuals; 2. Toxic waste production by firms; 3. R&D by a high-tech firm; and 4. Individual vaccination against communicable illness. (a) Is there an externality? If so, describe it, including references to whether it is positive or negative, and whether it is a consumption or production externality (b) If there is an externality, does it seem likely that private markets will arise that allow this externality to be internalized? Why or why not? Problem 2 Brookhaven has two regions. In Stony Brook, the marginal benefit associated with pollution cleanup is AB - 300 - 100, while in Port Jefferson, the marginal benefit associated with pol- lution cleanup is MB - 200 - 40. Suppose that the marginal cost of cleanup is constant at $12 per unit. What is the optimal level of pollution cleanup in each of the two regions? Problem 3 The private marginal benefit associated with a product's consumption is PAD - 360 - 49 and the private marginal cost associated with its production is PAC - 60. Furthermore, the marginal external damage associated with this good's production is MD - 20. To correct the externality, the government decides to impose a tax of 7 per unit sold. What tax T should it set to achieve the social optimumn?(20 points) The market inverse demand curve is P(y) = 10 2y, and a monopolist's cost curve is y2 + 2. (a) (b) What output level y maximizes the monopolist's revenue? What output level 3; maximizes the monopolist's prot? Identify which of the two output levels is lower, and explain Why using economic intuition. Suppose a second rm with cost curve y2 + 2 is considering entering the market. If after entry, the rms would compete a la Cournot, What would be the Cournot Nash equilibrium output levels yl and gm of rms 1 and 2? What would be the equilibrium prots for each rm? Will rm 2 choose to enter the market? Suppose that if rm 2 enters, both rms collude, choosing output levels that maxi mize total prots and then split the prots equally between them. What would be the prots to each rm? Will rm 2 choose to enter the market in this case? Given the following information for a monopoly firm: Demand: P = 76 - 5(Q) Marginal revenue: MR = 76 - 10(Q) Marginal cost: MC = 2(Q) + 2 Average total cost at equilibrium is 20 1. At what output (Q) will this firm maximize profit? 2. At what price (P) will this firm maximize profit 3. What is the total revenue (TR) earned at this output level 4. What is the total cost (TC) accrued at this output 5. What profit is earned Assume this firm is to be regulated. Answer the following questions: 6. What is the social optimal quantity 7. What is the social optimal price
















