solve the whole question

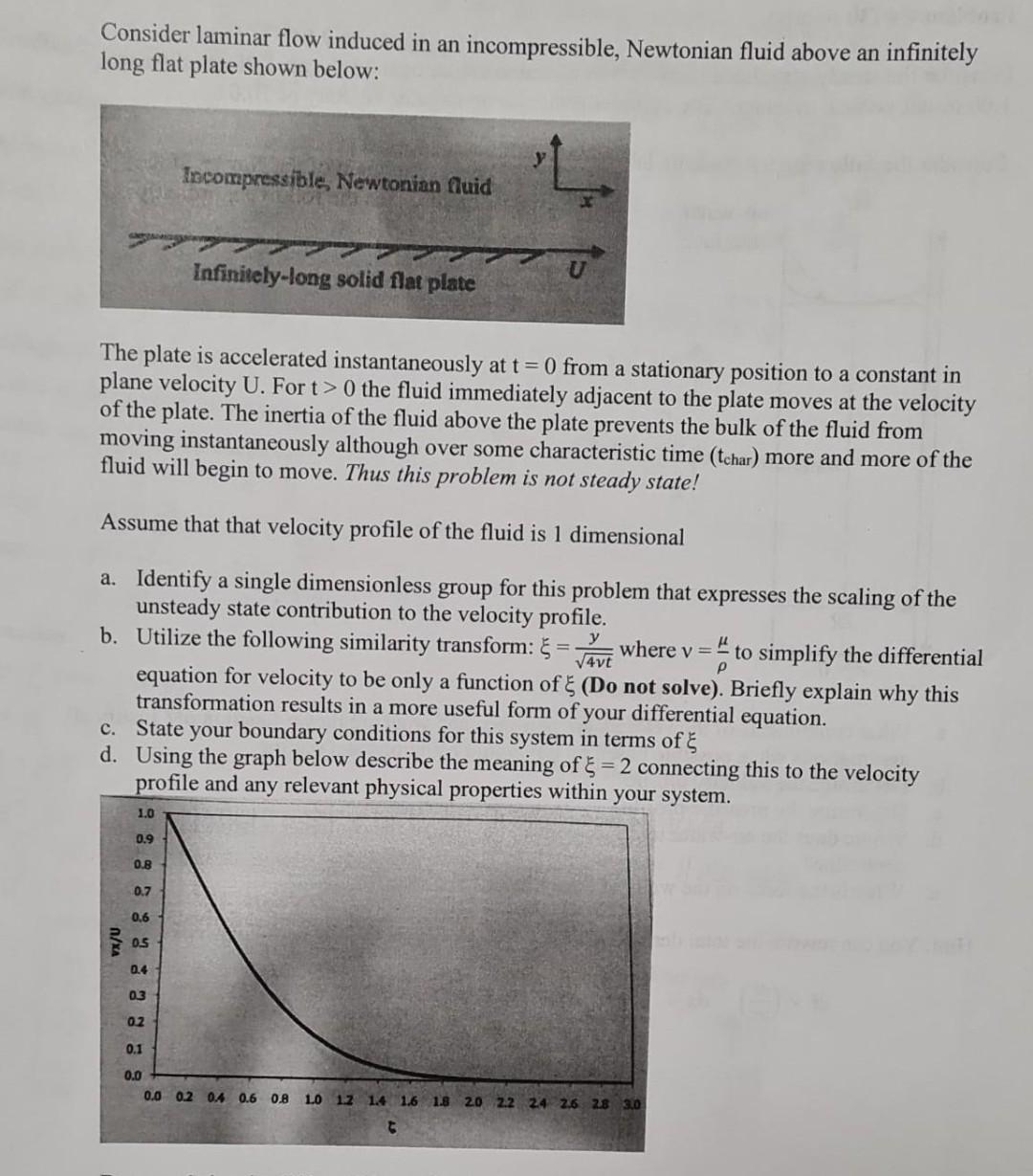
Consider laminar flow induced in an incompressible, Newtonian fluid above an infinitely long flat plate shown below: The plate is accelerated instantaneously at t=0 from a stationary position to a constant in plane velocity U. For t>0 the fluid immediately adjacent to the plate moves at the velocity of the plate. The inertia of the fluid above the plate prevents the bulk of the fluid from moving instantaneously although over some characteristic time (tchar) more and more of the fluid will begin to move. Thus this problem is not steady state! Assume that that velocity profile of the fluid is 1 dimensional a. Identify a single dimensionless group for this problem that expresses the scaling of the unsteady state contribution to the velocity profile. b. Utilize the following similarity transform: =4vty where v= to simplify the differential equation for velocity to be only a function of (Do not solve). Briefly explain why this transformation results in a more useful form of your differential equation. c. State your boundary conditions for this system in terms of d. Using the graph below describe the meaning of =2 connecting this to the velocity profile and any relevant physical properties within vour system