Answered step by step
Verified Expert Solution
Question
1 Approved Answer
some code to that I have but lost Please Help me with this because I am lost right now! I just realized that the def

some code to that I have but lost
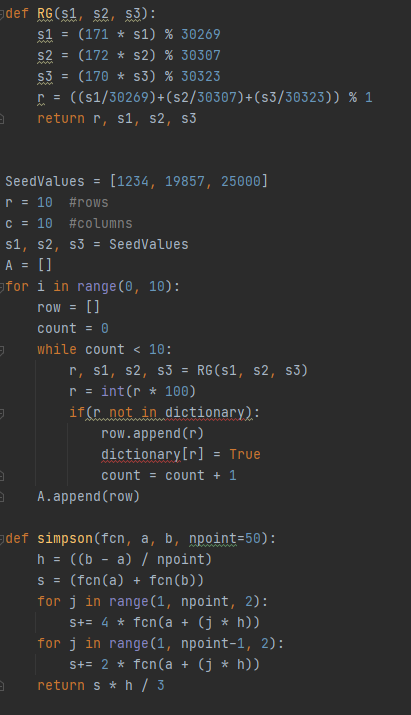
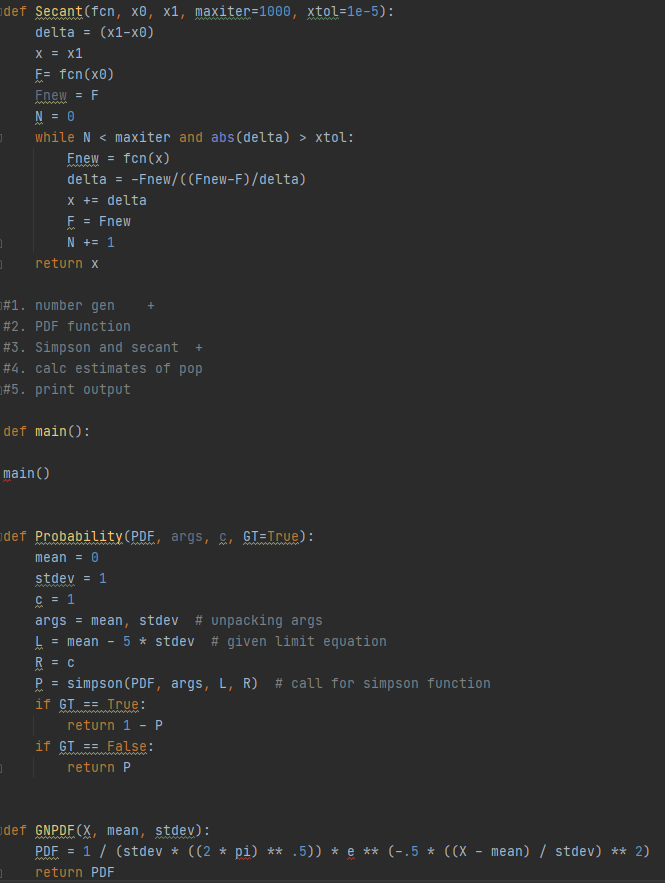
Please Help me with this because I am lost right now!
I just realized that the def probability is not needed in this so please look past that
Use your pseudorandom number generator from question 4 to generate a list of 1000 random floating point numbers between 0 and 1 (duplicates are okay). Consider these values to represent probability levels that can be found by integrating the Gaussian probability density function (PDF) between x=u-5.0 to x=x; such that pi=P(xStep by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


