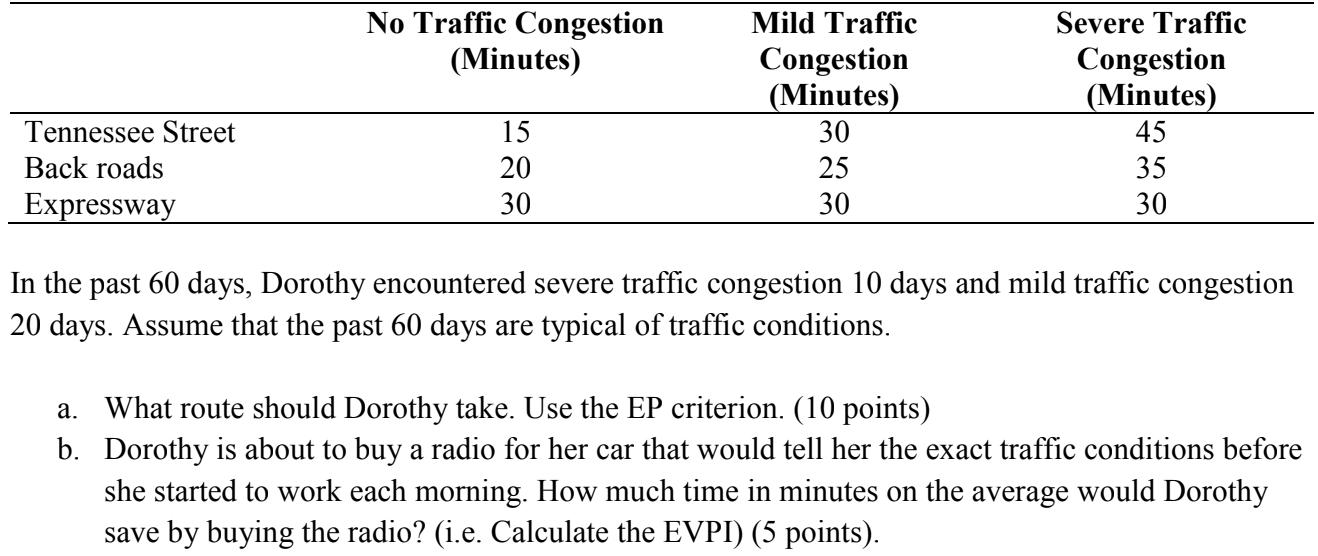
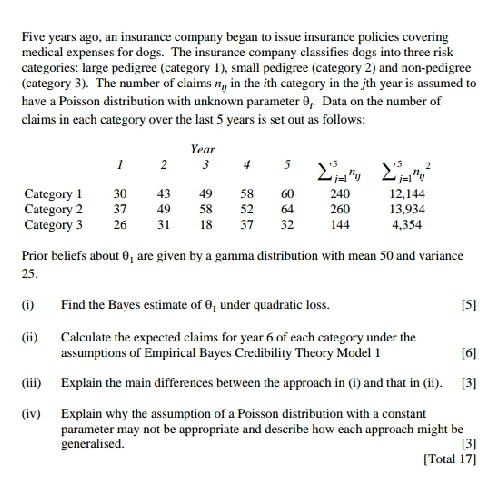
Suppose a market featuring two goods that differ in quality. Good 1 is of quality s and good 2 is of quality $2, with $2> 51. There is a mass of n = 1 consumers, of different types, identified by a taste parameter ~ U[ab]. Consumer's utility function is given by u(e): es-p if it buys one unit of good with quality s 0 otherwise In what follows, assume s = 1 and 52 = 2. In other words, firms cannot choose their quality, this is given and does not change. Firms cost functions are given by: Ci(qi) = csq; for i=1,2 where q; is the output of firm i. We will also conjecture that parameter values are such that there is full coverage. a) (4 points) Find the taste parameter that identifies a consumer who is indifferent between buying a unit of good 1 or a unit of good 2. b) (4 points) Derive an expression for the demand functions of each good (i.e., the demand functions that each firm is facing separately). c) (4 points) Set up each firm's problem. Find the firms' reaction functions and solve for the equilibrium prices d) (4 points) Assume now that c = 0.1, a = 1 and b = 3. Find the prices, quantities and profits for each firm. What are the market shares for each firm? Verify that the market is indeed fully covered (Hint: Think on the condition that needs to be met so that the market is fully covered) Tennessee Street Back roads Expressway No Traffic Congestion (Minutes) 15 20 30 Mild Traffic Congestion (Minutes) 30 25 30 Severe Traffic Congestion (Minutes) 45 35 30 In the past 60 days, Dorothy encountered severe traffic congestion 10 days and mild traffic congestion 20 days. Assume that the past 60 days are typical of traffic conditions. a. What route should Dorothy take. Use the EP criterion. (10 points) b. Dorothy is about to buy a radio for her car that would tell her the exact traffic conditions before she started to work each morning. How much time in minutes on the average would Dorothy save by buying the radio? (i.e. Calculate the EVPI) (5 points). Five years ago, an insurance company began to issue insurance policies covering medical expenses for dogs. The insurance company classifies dogs into three risk categories: large pedigree (category 1), small pedigree (category 2) and non-pedigree (category 3). The number of claims n, in the ith category in the jth year is assumed to have a Poisson distribution with unknown parameter 9, Data on the number of claims in each category over the last 5 years is set out as follows: Category 1 Category 2 Category 3 1 (1) 30 37 26 Year 2 3 4 43 49 58 49 58 52 31 18 37 5 60 64 32 2 2 j=1 240 260 144 12,144 13,934 4,354 Prior beliefs about 0, are given by a gamma distribution with mean 50 and variance 25. Find the Bayes estimate of 0, under quadratic loss. Calculate the expected claims for year 6 of each category under the assumptions of Empirical Bayes Credibility Theory Model 1 Explain the main differences between the approach in (1) and that in (ii). [5] [6] [3] (iii) (iv) Explain why the assumption of a Poisson distribution with a constant parameter may not be appropriate and describe how each approach might be generalised. [3] [Total 17]