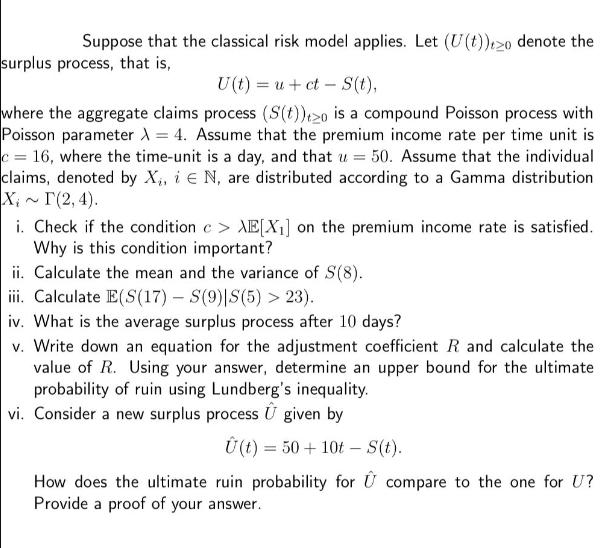
Suppose that the classical risk model applies. Let (U(t))+zo denote the surplus process, that is, U(t)=u+ct - S(t), where the aggregate claims process (S(t))+zo is a compound Poisson process with Poisson parameter = 4. Assume that the premium income rate per time unit is c=16, where the time-unit is a day, and that u = 50. Assume that the individual claims, denoted by X, EN, are distributed according to a Gamma distribution X,~ T(2, 4). i. Check if the condition c> XE[X] on the premium income rate is satisfied. Why is this condition important? ii. Calculate the mean and the variance of S(8). iii. Calculate E(S(17) S(9)|S(5) > 23). iv. What is the average surplus process after 10 days? v. Write down an equation for the adjustment coefficient R and calculate the value of R. Using your answer, determine an upper bound for the ultimate probability of ruin using Lundberg's inequality. vi. Consider a new surplus process U given by U(t) = 50+10t - S(t). How does the ultimate ruin probability for compare to the one for U? Provide a proof of your answer.