Question: Suppose that we consider two algorithms for some Problem. One of them has (worst-case) time complexity function T(n) = 12n (log2 n) + n

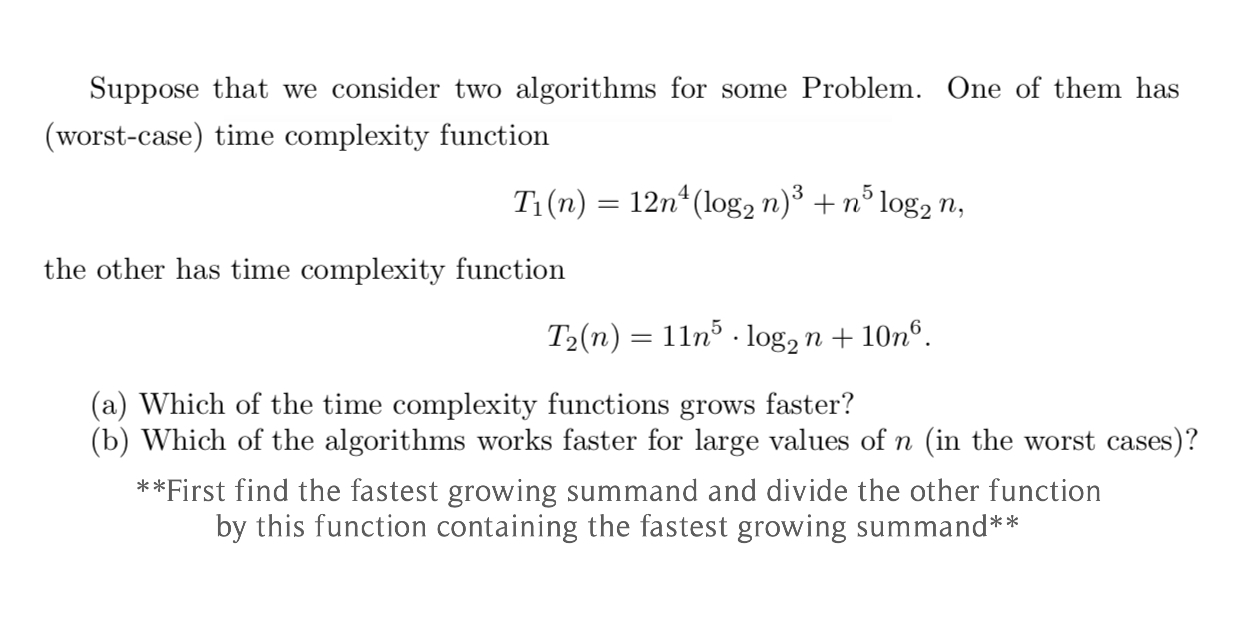
Suppose that we consider two algorithms for some Problem. One of them has (worst-case) time complexity function T(n) = 12n (log2 n) + n log2 n, the other has time complexity function T2(n) = 11n5 log2 n + 10n6. (a) Which of the time complexity functions grows faster? (b) Which of the algorithms works faster for large values of n (in the worst cases)? **First find the fastest growing summand and divide the other function by this function containing the fastest growing summand**

Step by Step Solution

★★★★★

3.32 Rating (143 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

To compare the growth rates of the two time complexity functions we need to analyze the dom... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

5. Statement of cash flows are included A. operating activities B. investing activities C. financing activities D. all of above

Python and most Python libraries are free to download or use, though many users use Python through a paid service. Paid services help IT organizations manage the risks associated with the use of...

Q1. You have identified a market opportunity for home media players that would cater for older members of the population. Many older people have difficulty in understanding the operating principles...

Credit Problem 2 (5 pts). Suppose that we consider two algorithms for some Problem. One of them has (worth-case) time complexity function the other has time complexity function (a) Which of the time...

Credit Problem 1 (5 pts). Suppose that we consider two algorithms for T, (n) = 12n'(log2 n)3 + n6 log2 n, T2(n) = 11 ns . log2 n + 1 0n6. some Problem. One of them has (worth-case) time complexity...

The Mandrill and Baboon are two (fictitious) problems that accept a weighted graph and return an integer. Consider the reduction below, which reduces the Mandrill problem to the Baboon problem. 1....

it is just disjoint set structure and algorithms Q3 (15 points) This question is related to disjoint set operations. Assume that we are using union by rank and find with path compression. Suppose...

Three alternative representations for non-negative integers, n, are: Peano: values have the form S(... S(Z) ...), applying S n times to Z where S and Z are constructors or constants of some data...

Question 1 2 points Which behaviors change the contents of a bag? clear ( ) add ( ) remove ( ) all of the above Signaler cette question Question 2 2 points Which method removes all entries of a bag?...

The Mandrill and Baboon are two (fictitious) problems that accept a weighted graph and return an integer. Consider the reduction below, which reduces the Mandrill problem to the Baboon problem....

Which of the following are means by which the body temperature of an organism is increased? I) perspiration II) piloerection (hairs standing up) III) vasoconstriction (constriction of blood vessels)...

For the following 13 real estate prices, calculate the IQR and determine if any prices are potential outliers. (Prices are in thosands of dollars) 389 230, 158, 479; 639 114: a) The IQR is: Number...

Question 1 0 I f s i n y = x , then t a n y = 1 - x 2 1 + x 2 2 x 1 - x 2 2 x 1 + x 2 2

Q) Abdullahs mother is a wonderful cook and her specialty is baking. Abdullah is a good student and he wants to implement his knowledge. One day while enjoying homemade oven fresh cookies with her...

Q) The research department of the Corn Flakes Corporation (CFC estimated the following regression for the demand of the corn flakes it sells: Qd = 15,000 - 10 P + 1500 A + 4 P x + 2 I Where Q d =...

Show that the matrix given here, with entries in GF (2 4 ), is the inverse of the matrix used in the Mix Columns step of S-AES. +1 x 3+) +1 +3

The informal economy refers to commercial activities that occur at least partly outside a governing bodys observation, taxation, and regulation. In slightly differ- ent words, sociologists Manuel...

Uncle Vern has just come into some money $100,000 and is thinking about putting this away into some investment accounts for a while. A: Vern is a simple guy so he goes to the bank and asks them...

It is widely recognized that the social security systems in many western democracies will face substantial shortfalls between anticipated revenues and promised benefits over the coming decades. A:...

In exercise 2.13, you considered the food stamp programs in the US. Under this program, poor households receive a certain quantity of food stamps stamps that contain a dollar value which is accepted...

The Lazer Company has a contract to produce a part for Boeing Corporation that must have an average diameter of 6 inches and a standard deviation of 0.10 inch. The Lazer Company has developed the...

Sanchez Electronics sells electronic components for car stereos. It claims that the average life of a component exceeds 4,000 hours. To test this claim, it has selected a random sample of n = 12 of...

Discuss why it is necessary to use an estimate of the standard error for a confidence interval and not for a hypothesis test concerning a population proportion.