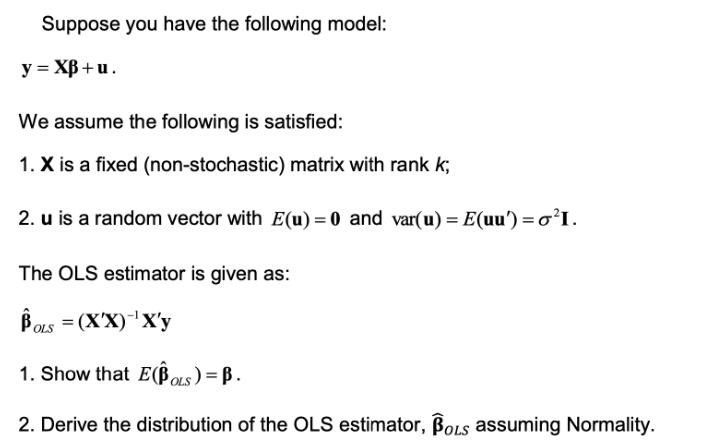
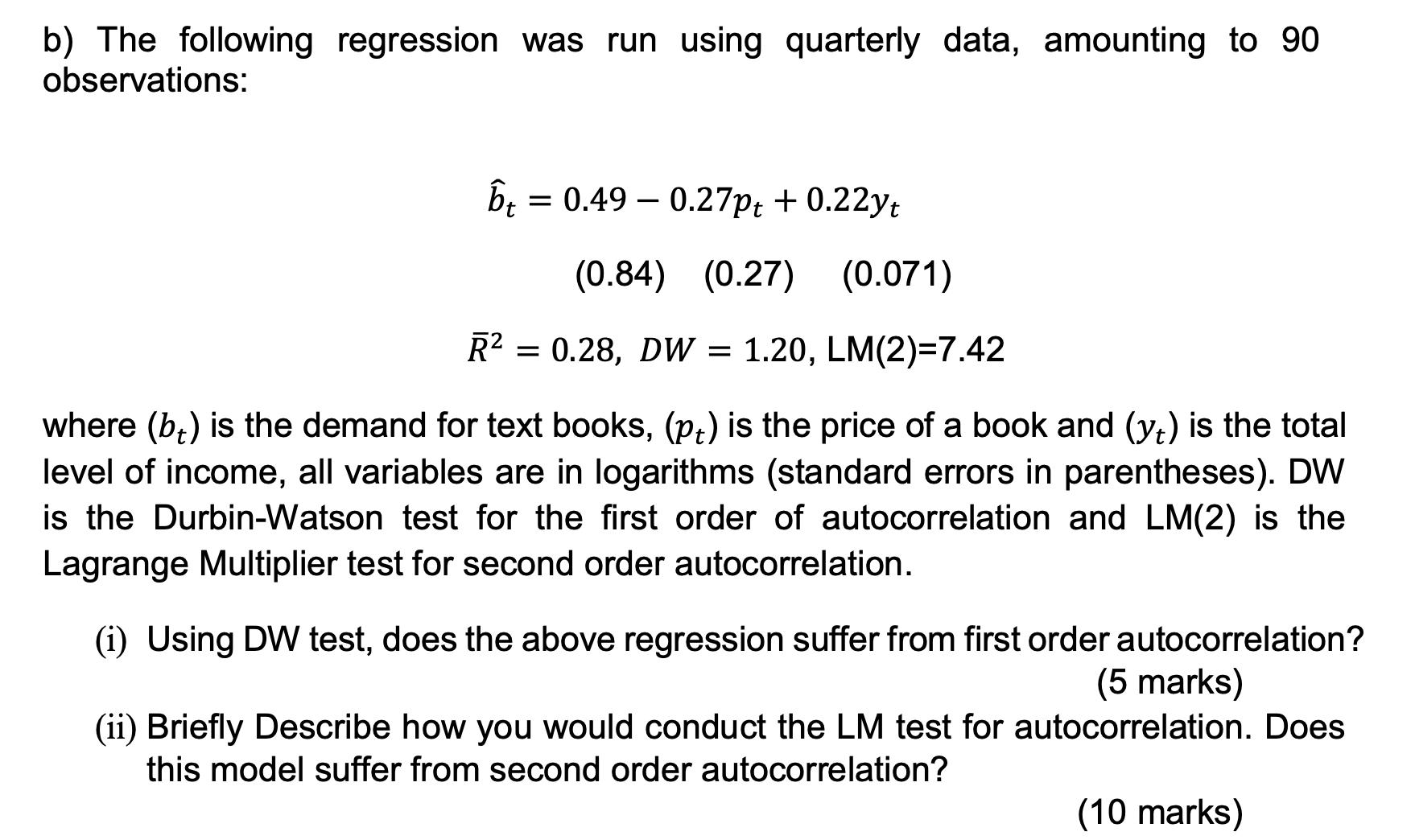
Suppose you have the following model: y = XB+u. We assume the following is satisfied: 1. X is a fixed (non-stochastic) matrix with rank k; 2. u is a random vector with E(u) = 0 and var(u) = E(uu')=oI. The OLS estimator is given as: BOLS = (X'X)-X'y 1. Show that E(BOLS) = B. 2. Derive the distribution of the OLS estimator, BoLs assuming Normality. b) The following regression was run using quarterly data, amounting to 90 observations: bt = = 0.49 -0.27pt + 0.22yt (0.84) (0.27) (0.071) R = 0.28, DW = 1.20, LM(2)=7.42 where (b) is the demand for text books, (pt) is the price of a book and (y) is the total level of income, all variables are in logarithms (standard errors in parentheses). DW is the Durbin-Watson test for the first order of autocorrelation and LM(2) is the Lagrange Multiplier test for second order autocorrelation. (i) Using DW test, does the above regression suffer from first order autocorrelation? (5 marks) (ii) Briefly Describe how you would conduct the LM test for autocorrelation. Does this model suffer from second order autocorrelation? (10 marks)