SURVIVAL ANALYSIS

NEED HELP ON QUESTION 3.4

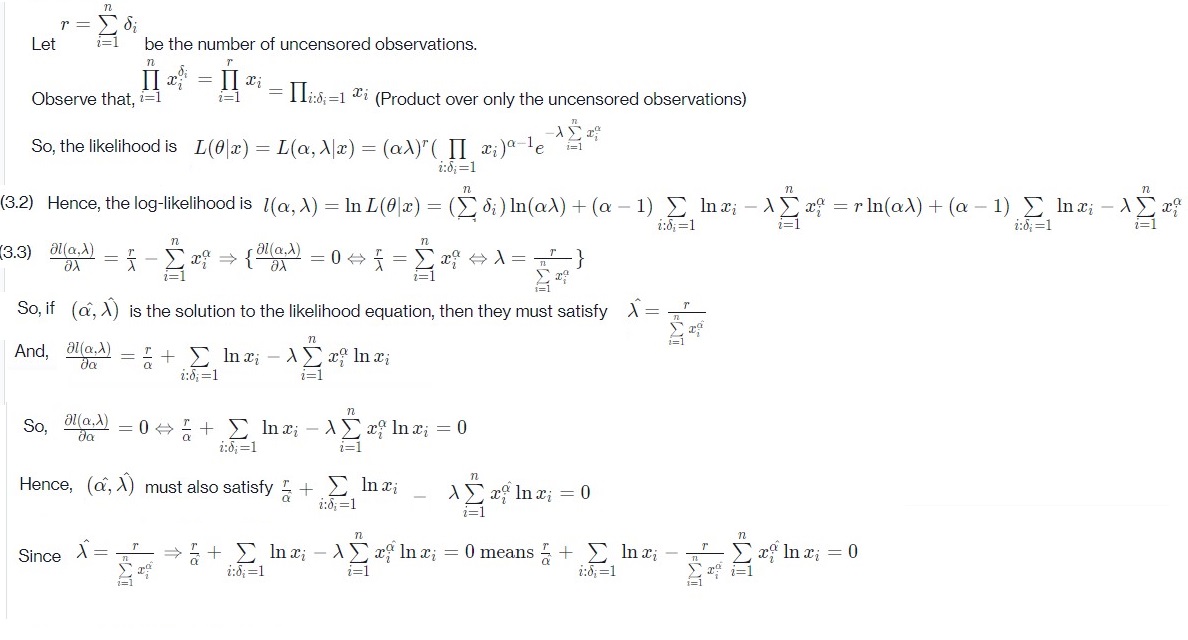
QUESTION 3 Parameter estimation in Survival analysis is done by maximizing the partial likelihood function F! L(9lw) = H [been son '3 1) i:1 with respect to the parameters contained in the p x 1 parameter vector 0 = (91, . . . ,Qp)'. In equation (E1), the n x 1 vector 3: represents the time values and the indicator variables a; take the value 1 when the I'm observation corresponds to an event and the value 0 when the rm observation is censored. It is often easier to maximise the natural logarithm, 3(9) : ln[L(9l:r:)], instead 0fL(9|m). Consider the Weibull distribution with probability density function given by f(x) : aixail exp {Zx'x} , where a > 0, A > O and x 3 0. (3.1) Write down the likelihood function (E1). Note that 9 : (a, A)'. (2) (3.2) Show that the log-likelihood function corresponding to the likelihood function (E1) is Haj):rhl(a2)+(a1):lnxianlle (E2) where r is the number of uhlcensoredlobservations (that is for which 51- = 1). (4) (3.3) Using equation (E2) in part (3.2), show that the likelihood estimator A of A is given by A r [I = n 2&- 21:1 xi and the likelihood estimator if of a is solution to the nonlinear equation 1 I 31 .- = . 3 3+2 um 2x. um () Suppose that in a clinical trial for a new drug, the investigator decides to stop the trial after observ- ing serious side effects on three individuals. The survival times (in months) of 10 volunteers in the study are: 2 5* 6* 7* 9 8* 7* 11* 9* 12 where the superscript * indicates a censored observation. Assume that the time to serious side effect follows a Weibull distribution with probability density function given above. (3.4) For these data use the results in part (3.3) to write down the equations for estimating a and A. You have to rearrange the data so that the equations make sense. (4) (3.1) The likelihood is L(0|x) = L(a, Xx) = (ax)'( II x; )o-le II xi = Product over all uncensored xis 1:6; =1 (3.2) The correct expression for log-likelihood is 1(0, 1) = rIn(ax) + (a -1) _ Inxi 1:0;=1 Inxi M- It should not be =1 . The sum is over the r observations which are uncensored but i=1 is not the correct way to say it. That is why I have used 1:6;=1 E (3.3) The second criterion should be : + _ Inx; Xx, Inc; = 0 Explanation: (3.1) The density function is f(x) = ada le d" 1220 Let F(.) denote the c.d.f. Since the density is supported on [0, Do), this means F(x) = 0 V x Ex, = rIn(a>) +(a -1) > Inc; -> I'M = 1:0; =1 1:6; =1 (3.3) al(a,A) n YE ax = I So, if (a, )) is the solution to the likelihood equation, then they must satisfy 1 = And, al(a,A) da 1:6;=1 So, al(a,X) da =09:+ > Ina; - 1Ca,Inc, = 0 1:6;=1 i=1 Hence, (a, )) must also satisfy , + _ Inci _ 1:6; =1 AEx Inc; = 0 n Since 1 = -+ > Inx; -ACafInx; = 0 means : + > Inc; "Inxi =0 1:01=1 1:6;=1