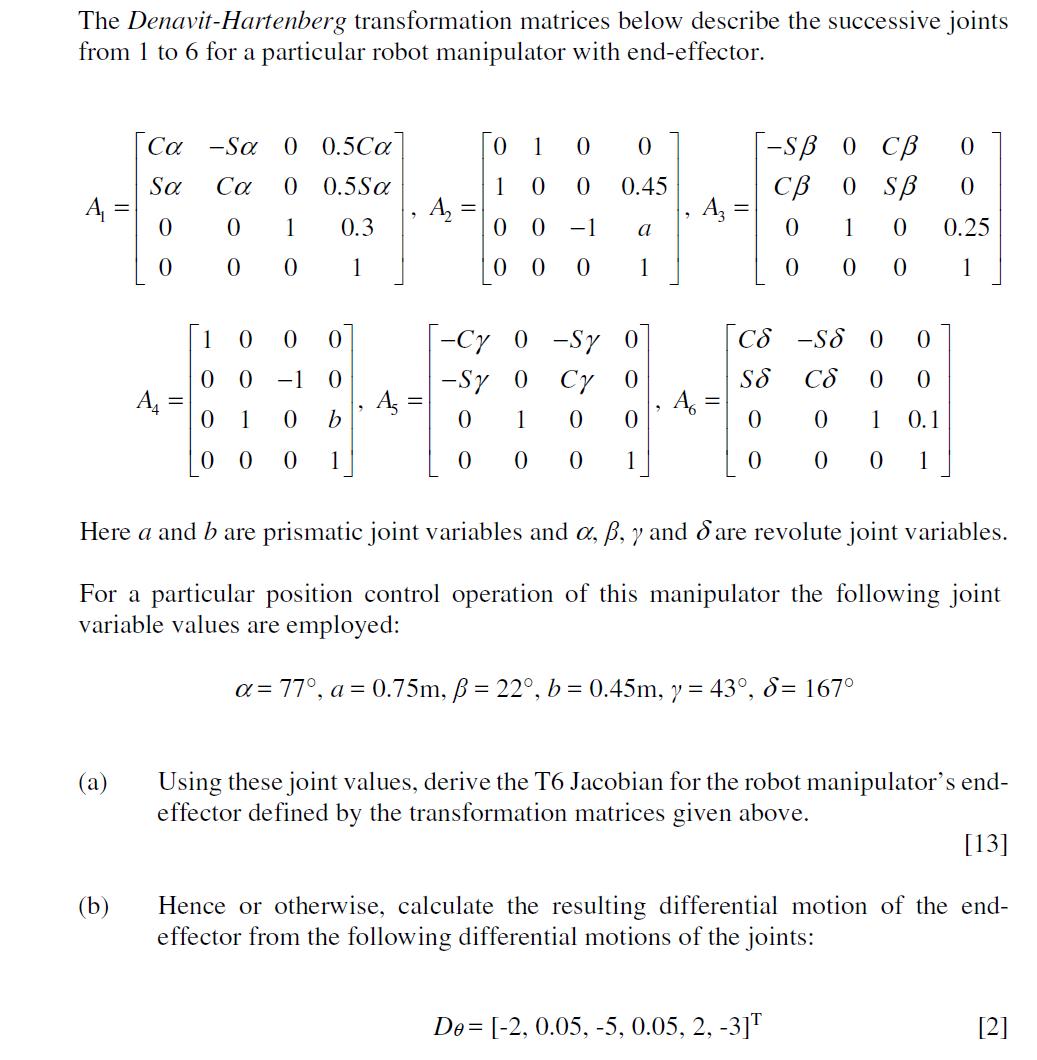
The Denavit-Hartenberg transformation matrices below describe the successive joints from 1 to 6 for a particular robot manipulator with end-effector. Ca -Sa 0 0.5C 0 1 0 0 -S 0 CB 0 Sa Ca 0 0.5Sa 1 0 0 0.45 CB 0 sp 0 A A = A3 0 0 1 0.3 00-1 a 0 1 0 0.25 0 0 0 1 00 0 1 0 0 0 1 A4 1 0 0 0 -Cy 0 -Sy 0 C8 -S8 0 0 0 0 -1 0 -S7 0 Cy 0 SE 0 0 = A A6 0 1 0 b 0 1 0 0 0 0 1 0.1 0 0 0 1 0 00 1 0 00 1 Here a and b are prismatic joint variables and , , y and are revolute joint variables. For a particular position control operation of this manipulator the following joint variable values are employed: =77, a = 0.75m, = 22, b = 0.45m, y = 43, &= 167 (a) Using these joint values, derive the T6 Jacobian for the robot manipulator's end- effector defined by the transformation matrices given above. (b) [13] Hence or otherwise, calculate the resulting differential motion of the end- effector from the following differential motions of the joints: De [-2, 0.05, -5, 0.05, 2, -3]T [2]