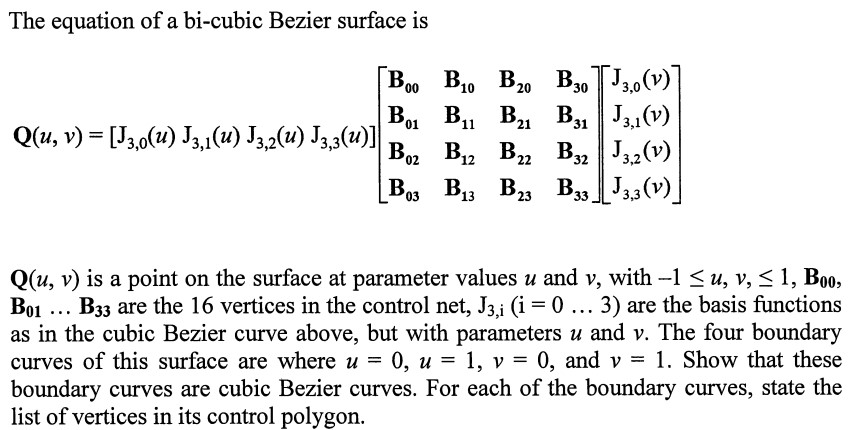
The equation of a Bezier curve is 11 P(t) = BiJni(t), i=0 0t1 where Bi are the control points and the basis function Jni(t) -()*^ (1-1)**, with n n! == i!(n-i)! The equation of a bi-cubic Bezier surface is [B00 Boo B10 B20 B30 B20 B303,0(V) Q(u, v) = [J3,0(u) J3,1(U) J3,2(u) J3,3(u)]| B. B01 B1 B2 B31 3,1 (V) 11 21 J3,1 B02 B12 B22 B32 3,2(V) B03 B13 B23 B333,3 (V) ... Q(u, v) is a point on the surface at parameter values u and v, with -1 u, v, 1, Boo, B01 B33 are the 16 vertices in the control net, J3,i (i = 0 ... 3) are the basis functions as in the cubic Bezier curve above, but with parameters u and v. The four boundary curves of this surface are where u = 0, u = 1, v = 0, and v = 1. Show that these boundary curves are cubic Bezier curves. For each of the boundary curves, state the list of vertices in its control polygon.