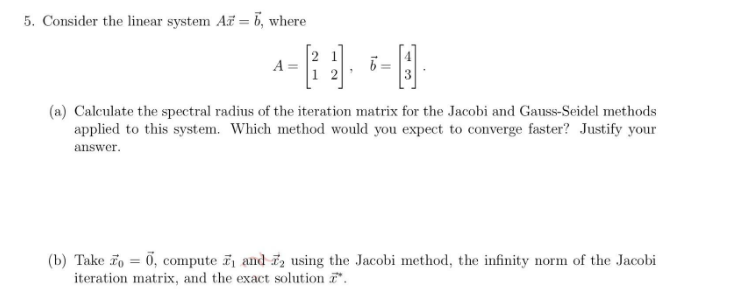
Question
The following table is a transportation matrix showing the cost ($) per unit of shipping from Plant i to Distributor j. The objective is to
The following table is a transportation matrix showing the cost ($) per unit of shipping from Plant i to Distributor j. The objective is to minimize shipping costs while meeting the demand placed by each Distributor. There is no shipment between Plant 2 and Distributor 3.
Distributor 1 Distributor 2 Distributor 3 Supply
Plant 1. 9 22. 24. 430
Plant 2. 14. 10 ..... 360
Plant 3. 18. 12. 13 220
Demand 400 390 190. ---
Let xij = number units shipped from Plant i to Distributor j
Solve the transportation problem and use the result to fill the boxes below.
All answers are equally weighted.
a) At optimal solution:
x11=
x12=
x13=
x21=
x22=
x23=
x31=
x32=
x33=
Minimum Cost = $
b) At optimal solution, how much unused capacity ...
[6:02 AM, 4/4/2021] Kossieh: Question:
In the `MASS` library, combine the two datasets `Pima.te` and `Pima.tr` back into one complete dataset, call it `pima`. (Try function `rbind()`.) How many observations are there?
*Train-Test split 3:1*
In order to perform KNN analysis, we need to separate the X-variables and the y-labels. (Which should be our y-variable?) Before we separate them out, create vector/array of 1 and 2 to create train-test split in the ratio of 3:1. (Set a constant seed value so that we can duplicate the results.) So eventually, you will get the training Xs as a dataframe, training y-label (a vector), as well as the test sets together in four groups. Make sure the train-X and train-y are not mixed up in the ordering during the process. Same for test-X and test-y.
*KNN results*
Perform the KNN analysis, with different k values. You do not need to show all the results from different k, but please include the one with the best (total) accuracy in your submission. How does the accuracy compared to the percentages of being T/F in the dataset?
Perform inn in Pima Dataset .
[6:11 AM, 4/4/2021] Kossieh: Question:
Please help keep getting it wrong!
According to the WHO MONICA Project the mean blood pressure for people in China is 128 mmHg with a standard deviation of 23 mmHg. Assume that blood pressure is normally distributed.
Round the probabilities to four decimal places.
b) Find the probability that a randomly selected person in China has a blood pressure of 110.9 mmHg or more.
c) Find the probability that a randomly selected person in China has a blood pressure of 152.3 mmHg or less.
d) Find the probability that a randomly selected person in China has a blood pressure between 110.9 and 152.3 mmHg.
e) Find the probability that randomly selected person in China has a blood pressure that is at most 70.5 mmHg.
[6:37 AM, 4/4/2021] Kossieh: Question:
1) The statistics being tested in a linear regression is called
F
T
Z
R
2) If we reject the null hypothesis for a linear regression, we are saying that the two variables
are related.
are not related.
are irrelevant.
positively correlated.
3) Another term for the error in the regression line is
residuals.
surplus.
deficit.
type II.
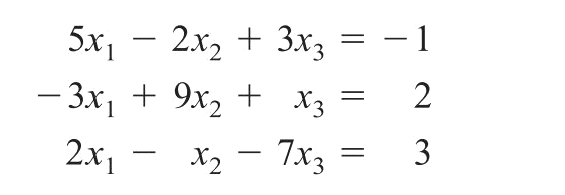

Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


