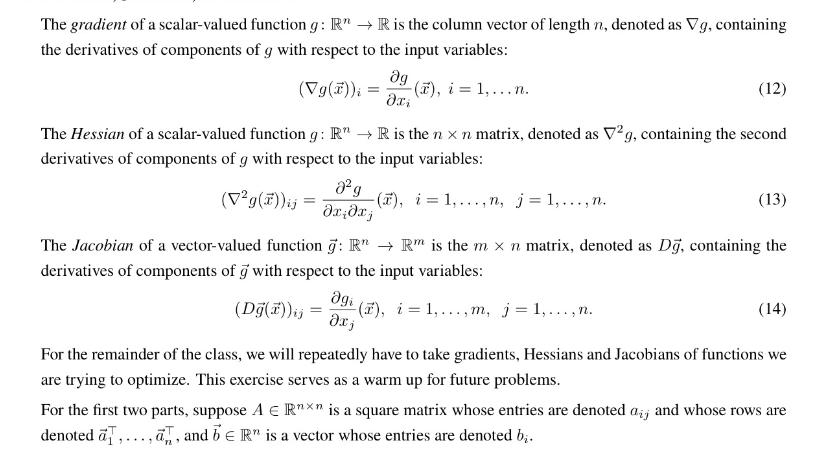
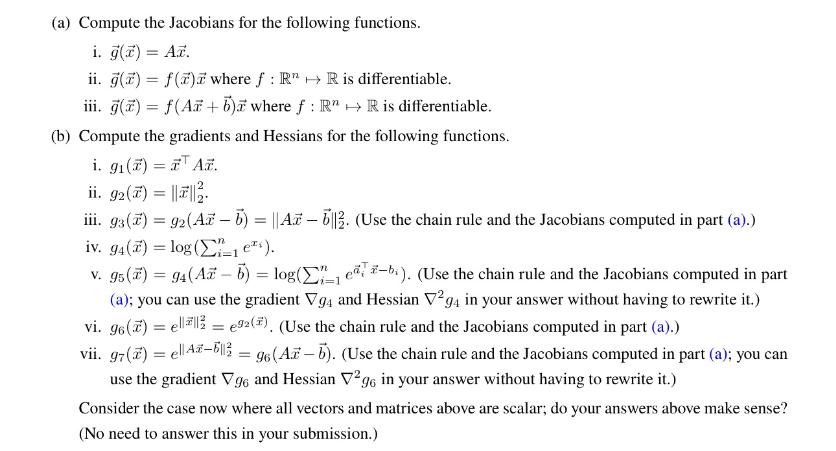
The gradient of a scalar-valued function g: RR is the column vector of length n, denoted as Vg, containing the derivatives of components of g with respect to the input variables: (Vg()); = (a), i = 1,...n. (12) The Hessian of a scalar-valued function g: R" R is the n x n matrix, denoted as V2g, containing the second derivatives of components of g with respect to the input variables: (Vg())ij = (a), i=1,...,n, j = 1,..., n. (13) The Jacobian of a vector-valued function : R R is the m x n matrix, denoted as D, containing the derivatives of components of g with respect to the input variables: xj (14) (Dg(x))ij = (), i = 1,..., m, j = 1,..., n. For the remainder of the class, we will repeatedly have to take gradients, Hessians and Jacobians of functions we are trying to optimize. This exercise serves as a warm up for future problems. For the first two parts, suppose A E Rnxn is a square matrix whose entries are denoted a; and whose rows are denoted a ,...,, and b R" is a vector whose entries are denoted b;. (a) Compute the Jacobians for the following functions. i. g(x) = Ax. ii. () f() where f: R" R is differentiable. iii. g(x) = (Ax + b) where f: R R is differentiable. (b) Compute the gradients and Hessians for the following functions. i. 91 (7) = Ax. ii. 92(7) = |||| iii. 93()=92(4x-6)= ||A||2. (Use the chain rule and the Jacobians computed in part (a).) iv. 94() = log(-es). v. 95(x) = 94 (Ax - b) = log(-1 e-bi). (Use the chain rule and the Jacobians computed in part (a); you can use the gradient V94 and Hessian V294 in your answer without having to rewrite it.) vi. 96()=e=e92(). (Use the chain rule and the Jacobians computed in part (a).) vii. 97(x)=ell 4-5=96 (A-6). (Use the chain rule and the Jacobians computed in part (a); you can use the gradient Vg6 and Hessian V296 in your answer without having to rewrite it.) Consider the case now where all vectors and matrices above are scalar; do your answers above make sense? (No need to answer this in your submission.)