The last three picture
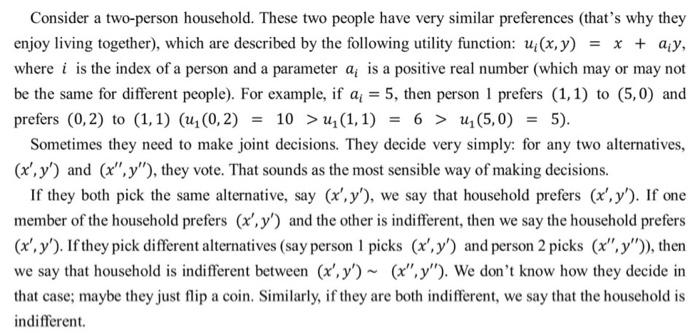
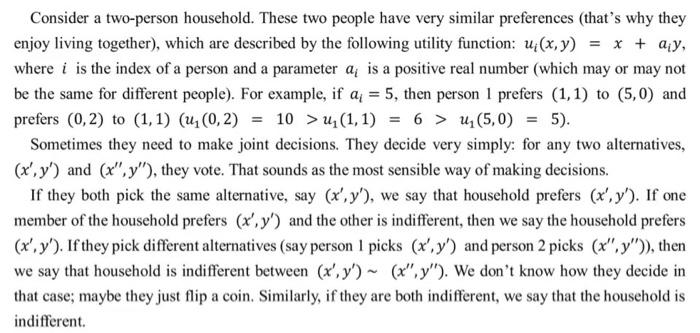
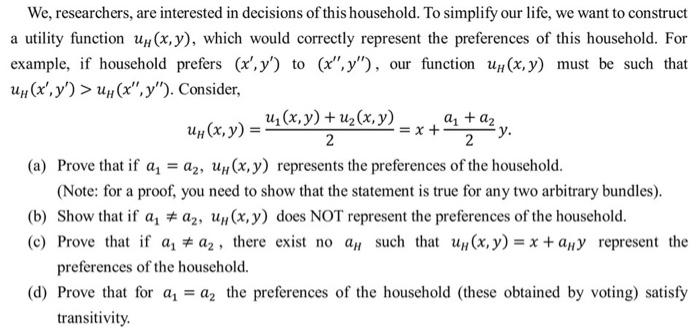
Consider a two-person household. These two people have very similar preferences (that's why they enjoy living together), which are described by the following utility function(*.y) - + 4y. wherei is the index of a person and a parameter is a positive sal number (which may or may not be the same for different people). For example, if 4 - 5. then persent prefers (1.1) to (5,0) and prefers (0.2) to (1.1) (,(0.2) - 10 >:(1.1) - 6 > (5,0) = 5). Sometimes they need to make joint decisions. They decide very simply for any two alternatives, (X.) and (x".y), they vote. That sounds as the most sensible way of making decisions If they both pick the same alterative, say (2.), we say that houschold prefers (X.) If one member of the household prefers (8.2) and the other is indifferent, then we say the household prefers ("./). If they pick different sinematives (say person I picks (2.) and person 2 picks (*".')), then we say that houschold is indifferent between (1.)-("). We don't know how they decide in that case, maybe they just flip a coin. Similarly if they are both indifferent, we say that the household is indifferent We researchers are interested in decisions of this household. To simplify our life, we want to construct a utility function (x,y), which would correctly represent the preferences of this household. For example. If houschold prefers (1* (**). cer function e(*.y) must be such that (X.)>(x",y). Consider u2(x.y) + z(x,y) Wy) = (a) Prove that if a = 0, wy) represents the preferences of the household. (Note: for a proof, you need to show that the statement is true for any two arbitrary bundles). (b) Show that if 4, #. G.y) does NOT represent the preferences of the houshold () Prove that if any, there is no such that y)=x+4y represent the preferences of the household (d) Prove that for a = the preferences of the household (these obtained by voting) satisfy transitivity We switch now to a three person household (with preferences ,y)= x + 47.62(x,y) = x + ayu,(x,y) = x + 0,7). They abo decide by voting if bundle (x,y) collects more votes than the bundle (3".), then (y) is selected. Apexe who is indifferent does not casta That is, if I and 2 peefer ("7). It will be selected regardless of what 3 prefers. If1 prefers (x,y). 2 is indifferent, and 3 prefiers (x"./) then the household is indifferent. If 1 and 2 ure indifferent then the household would select what 3 prekes les 3 is also indifferent, in which case the houschold would also be indifferent (c) Prove that preferences are transitive or give a counter-example ( Show that there is a lust one combination of values and such that these preferences cannot be represented by Oxy) = x + y for any Hints (b): you need to choose two bundles such that 2(5.9) and the woning procedure i described disagree on what the houschold prefers. Choose your bundles carefully: how difficult your algebra is would depend on your choice (ex your construction in (b) may guide your proof in (c. One way to start the proof is by saying suppose such or exists. Then you can derive some claim about ww&x.y) which contradicts that wn(x,y) represent the household preferences 5). Consider a two-person household. These two people have very similar preferences (that's why they enjoy living together), which are described by the following utility function: u(x,y) = x + ay, where i is the index of a person and a parameter ai is a positive real number (which may or may not be the same for different people). For example, if a = 5, then person I prefers (1,1) to (5,0) and prefers (0,2) to (1,1) (u; (0,2) = 10 > u1(1.1) = 6 > 4(5,0) Sometimes they need to make joint decisions. They decide very simply: for any two alternatives, (x',y') and (x",y'), they vote. That sounds as the most sensible way of making decisions. If they both pick the same alternative, say (x",y'), we say that household prefers (x",y'). If one member of the household prefers (x',y') and the other is indifferent, then we say the household prefers (x",y'). If they pick different alternatives (say person I picks (x,y) and person 2 picks (x",y")), then we say that household is indifferent between (x",y') - (x",y''). We don't know how they decide in that case; maybe they just flip a coin. Similarly, if they are both indifferent, we say that the household is indifferent. We, researchers, are interested in decisions of this household. To simplify our life, we want to construct a utility function up(x,y), which would correctly represent the preferences of this household. For example, if household prefers (x,y) to (x",y'), our function Up(x,y) must be such that uy(x'.y') > (x",y''). Consider, u(x,y) + uz(x,y) un(x, y) = at az X + 2 2 y. (a) Prove that if a; = az, uy(x,y) represents the preferences of the household. (Note: for a proof, you need to show that the statement is true for any two arbitrary bundles). (b) Show that if a, #az, uy(x,y) does NOT represent the preferences of the household. (c) Prove that if a # ay, there exist no ah such that un(x, y) = x + any represent the preferences of the household. (d) Prove that for a = a, the preferences of the household (these obtained by voting) satisfy transitivity, We switch now to a three-person household (with preferences uz(x, y) = x + azy, u2(x,y) = * + azy, uz(x,y) = x + azy). They also decide by voting: if bundle (x",y') collects more votes than the bundle (x",y"), then (x',y') is selected. A person who is indifferent does not cast a vote. That is, if 1 and 2 prefer (x',y'), it will be selected regardless of what 3 prefers. If I prefers (x',y'), 2 is indifferent, and 3 prefers (x",y"), then the household is indifferent. If 1 and 2 are indifferent, then the household would select what 3 prefers (unless 3 is also indifferent, in which case the household would also be indifferent). (e) Prove that preferences are transitive or give a counter-example. (1) Show that there is at least one combination of values aj, az and az such that these preferences cannot be represented by un(x,y) = x + ayy for any ap. Hints (b): you need to choose two bundles such that up (x,y) and the voting procedure I described disagree on what the household prefers. Choose your bundles carefully: how difficult your algebra is would depend on your choice. (c): your construction in (b) may guide your proof in (c). One way to start the proof is by saying: suppose such an exists. Then you can derive some claim about up(x,y) which contradicts that un(x,y) represent the household preferences. 5). Consider a two-person household. These two people have very similar preferences (that's why they enjoy living together), which are described by the following utility function: u(x,y) = x + ay, where i is the index of a person and a parameter a; is a positive real number (which may or may not be the same for different people). For example, if a; = 5, then person I prefers (1,1) to (5,0) and prefers (0,2) to (1,1) (u(0,2) = 10 > u,(1,1) = 6 > 4 (5,0) Sometimes they need to make joint decisions. They decide very simply for any two alternatives, (x',y) and (x",y"), they vote. That sounds as the most sensible way of making decisions. If they both pick the same alternative, say (x",y'), we say that household prefers (x",y'). If one member of the household prefers (x'.y') and the other is indifferent, then we say the household prefers (x",y'). If they pick different alternatives (say person I picks (x",y') and person 2 picks (x",y")), then we say that household is indifferent between (x',y') - (x",y"). We don't know how they decide in that case; maybe they just flip a coin. Similarly, if they are both indifferent, we say that the household is indifferent. We, researchers, are interested in decisions of this household. To simplify our life, we want to construct a utility function un(x,y), which would correctly represent the preferences of this household. For example, if household prefers (x',y') to (x",y'), our function un(x,y) must be such that Up(x,y') > Up(x",y''). Consider, u(x,y) + u2(x, y) Un(x,y) = a + a x + 2 2 y. (a) Prove that if a = az, up (x,y) represents the preferences of the household. (Note: for a proof, you need to show that the statement is true for any two arbitrary bundles). (b) Show that if a, #az, uy(x,y) does NOT represent the preferences of the household. (e) Prove that if aj #az, there exist no am such that un(x,y) = x + any represent the preferences of the household. (d) Prove that for a = az the preferences of the household (these obtained by voting) satisfy transitivity, We switch now to a three-person household (with preferences uz(x, y) = x + azy, u2(x,y) = * + azy, uz(x,y) = x + azy). They also decide by voting: if bundle (x",y') collects more votes than the bundle (x",y"), then (x',y') is selected. A person who is indifferent does not cast a vote. That is, if 1 and 2 prefer (x',y'), it will be selected regardless of what 3 prefers. If I prefers (x',y'), 2 is indifferent, and 3 prefers (x",y"), then the household is indifferent. If 1 and 2 are indifferent, then the household would select what 3 prefers (unless 3 is also indifferent, in which case the household would also be indifferent). (e) Prove that preferences are transitive or give a counter-example. (1) Show that there is at least one combination of values aj, az and az such that these preferences cannot be represented by un(x,y) = x + ayy for any ap. Hints (b): you need to choose two bundles such that up (x,y) and the voting procedure I described disagree on what the household prefers. Choose your bundles carefully: how difficult your algebra is would depend on your choice. (c): your construction in (b) may guide your proof in (c). One way to start the proof is by saying: suppose such an exists. Then you can derive some claim about up(x,y) which contradicts that un(x,y) represent the household preferences. Consider a two-person household. These two people have very similar preferences (that's why they enjoy living together), which are described by the following utility function(*.y) - + 4y. wherei is the index of a person and a parameter is a positive sal number (which may or may not be the same for different people). For example, if 4 - 5. then persent prefers (1.1) to (5,0) and prefers (0.2) to (1.1) (,(0.2) - 10 >:(1.1) - 6 > (5,0) = 5). Sometimes they need to make joint decisions. They decide very simply for any two alternatives, (X.) and (x".y), they vote. That sounds as the most sensible way of making decisions If they both pick the same alterative, say (2.), we say that houschold prefers (X.) If one member of the household prefers (8.2) and the other is indifferent, then we say the household prefers ("./). If they pick different sinematives (say person I picks (2.) and person 2 picks (*".')), then we say that houschold is indifferent between (1.)-("). We don't know how they decide in that case, maybe they just flip a coin. Similarly if they are both indifferent, we say that the household is indifferent We researchers are interested in decisions of this household. To simplify our life, we want to construct a utility function (x,y), which would correctly represent the preferences of this household. For example. If houschold prefers (1* (**). cer function e(*.y) must be such that (X.)>(x",y). Consider u2(x.y) + z(x,y) Wy) = (a) Prove that if a = 0, wy) represents the preferences of the household. (Note: for a proof, you need to show that the statement is true for any two arbitrary bundles). (b) Show that if 4, #. G.y) does NOT represent the preferences of the houshold () Prove that if any, there is no such that y)=x+4y represent the preferences of the household (d) Prove that for a = the preferences of the household (these obtained by voting) satisfy transitivity We switch now to a three person household (with preferences ,y)= x + 47.62(x,y) = x + ayu,(x,y) = x + 0,7). They abo decide by voting if bundle (x,y) collects more votes than the bundle (3".), then (y) is selected. Apexe who is indifferent does not casta That is, if I and 2 peefer ("7). It will be selected regardless of what 3 prefers. If1 prefers (x,y). 2 is indifferent, and 3 prefiers (x"./) then the household is indifferent. If 1 and 2 ure indifferent then the household would select what 3 prekes les 3 is also indifferent, in which case the houschold would also be indifferent (c) Prove that preferences are transitive or give a counter-example ( Show that there is a lust one combination of values and such that these preferences cannot be represented by Oxy) = x + y for any Hints (b): you need to choose two bundles such that 2(5.9) and the woning procedure i described disagree on what the houschold prefers. Choose your bundles carefully: how difficult your algebra is would depend on your choice (ex your construction in (b) may guide your proof in (c. One way to start the proof is by saying suppose such or exists. Then you can derive some claim about ww&x.y) which contradicts that wn(x,y) represent the household preferences 5). Consider a two-person household. These two people have very similar preferences (that's why they enjoy living together), which are described by the following utility function: u(x,y) = x + ay, where i is the index of a person and a parameter ai is a positive real number (which may or may not be the same for different people). For example, if a = 5, then person I prefers (1,1) to (5,0) and prefers (0,2) to (1,1) (u; (0,2) = 10 > u1(1.1) = 6 > 4(5,0) Sometimes they need to make joint decisions. They decide very simply: for any two alternatives, (x',y') and (x",y'), they vote. That sounds as the most sensible way of making decisions. If they both pick the same alternative, say (x",y'), we say that household prefers (x",y'). If one member of the household prefers (x',y') and the other is indifferent, then we say the household prefers (x",y'). If they pick different alternatives (say person I picks (x,y) and person 2 picks (x",y")), then we say that household is indifferent between (x",y') - (x",y''). We don't know how they decide in that case; maybe they just flip a coin. Similarly, if they are both indifferent, we say that the household is indifferent. We, researchers, are interested in decisions of this household. To simplify our life, we want to construct a utility function up(x,y), which would correctly represent the preferences of this household. For example, if household prefers (x,y) to (x",y'), our function Up(x,y) must be such that uy(x'.y') > (x",y''). Consider, u(x,y) + uz(x,y) un(x, y) = at az X + 2 2 y. (a) Prove that if a; = az, uy(x,y) represents the preferences of the household. (Note: for a proof, you need to show that the statement is true for any two arbitrary bundles). (b) Show that if a, #az, uy(x,y) does NOT represent the preferences of the household. (c) Prove that if a # ay, there exist no ah such that un(x, y) = x + any represent the preferences of the household. (d) Prove that for a = a, the preferences of the household (these obtained by voting) satisfy transitivity, We switch now to a three-person household (with preferences uz(x, y) = x + azy, u2(x,y) = * + azy, uz(x,y) = x + azy). They also decide by voting: if bundle (x",y') collects more votes than the bundle (x",y"), then (x',y') is selected. A person who is indifferent does not cast a vote. That is, if 1 and 2 prefer (x',y'), it will be selected regardless of what 3 prefers. If I prefers (x',y'), 2 is indifferent, and 3 prefers (x",y"), then the household is indifferent. If 1 and 2 are indifferent, then the household would select what 3 prefers (unless 3 is also indifferent, in which case the household would also be indifferent). (e) Prove that preferences are transitive or give a counter-example. (1) Show that there is at least one combination of values aj, az and az such that these preferences cannot be represented by un(x,y) = x + ayy for any ap. Hints (b): you need to choose two bundles such that up (x,y) and the voting procedure I described disagree on what the household prefers. Choose your bundles carefully: how difficult your algebra is would depend on your choice. (c): your construction in (b) may guide your proof in (c). One way to start the proof is by saying: suppose such an exists. Then you can derive some claim about up(x,y) which contradicts that un(x,y) represent the household preferences. 5). Consider a two-person household. These two people have very similar preferences (that's why they enjoy living together), which are described by the following utility function: u(x,y) = x + ay, where i is the index of a person and a parameter a; is a positive real number (which may or may not be the same for different people). For example, if a; = 5, then person I prefers (1,1) to (5,0) and prefers (0,2) to (1,1) (u(0,2) = 10 > u,(1,1) = 6 > 4 (5,0) Sometimes they need to make joint decisions. They decide very simply for any two alternatives, (x',y) and (x",y"), they vote. That sounds as the most sensible way of making decisions. If they both pick the same alternative, say (x",y'), we say that household prefers (x",y'). If one member of the household prefers (x'.y') and the other is indifferent, then we say the household prefers (x",y'). If they pick different alternatives (say person I picks (x",y') and person 2 picks (x",y")), then we say that household is indifferent between (x',y') - (x",y"). We don't know how they decide in that case; maybe they just flip a coin. Similarly, if they are both indifferent, we say that the household is indifferent. We, researchers, are interested in decisions of this household. To simplify our life, we want to construct a utility function un(x,y), which would correctly represent the preferences of this household. For example, if household prefers (x',y') to (x",y'), our function un(x,y) must be such that Up(x,y') > Up(x",y''). Consider, u(x,y) + u2(x, y) Un(x,y) = a + a x + 2 2 y. (a) Prove that if a = az, up (x,y) represents the preferences of the household. (Note: for a proof, you need to show that the statement is true for any two arbitrary bundles). (b) Show that if a, #az, uy(x,y) does NOT represent the preferences of the household. (e) Prove that if aj #az, there exist no am such that un(x,y) = x + any represent the preferences of the household. (d) Prove that for a = az the preferences of the household (these obtained by voting) satisfy transitivity, We switch now to a three-person household (with preferences uz(x, y) = x + azy, u2(x,y) = * + azy, uz(x,y) = x + azy). They also decide by voting: if bundle (x",y') collects more votes than the bundle (x",y"), then (x',y') is selected. A person who is indifferent does not cast a vote. That is, if 1 and 2 prefer (x',y'), it will be selected regardless of what 3 prefers. If I prefers (x',y'), 2 is indifferent, and 3 prefers (x",y"), then the household is indifferent. If 1 and 2 are indifferent, then the household would select what 3 prefers (unless 3 is also indifferent, in which case the household would also be indifferent). (e) Prove that preferences are transitive or give a counter-example. (1) Show that there is at least one combination of values aj, az and az such that these preferences cannot be represented by un(x,y) = x + ayy for any ap. Hints (b): you need to choose two bundles such that up (x,y) and the voting procedure I described disagree on what the household prefers. Choose your bundles carefully: how difficult your algebra is would depend on your choice. (c): your construction in (b) may guide your proof in (c). One way to start the proof is by saying: suppose such an exists. Then you can derive some claim about up(x,y) which contradicts that un(x,y) represent the household preferences