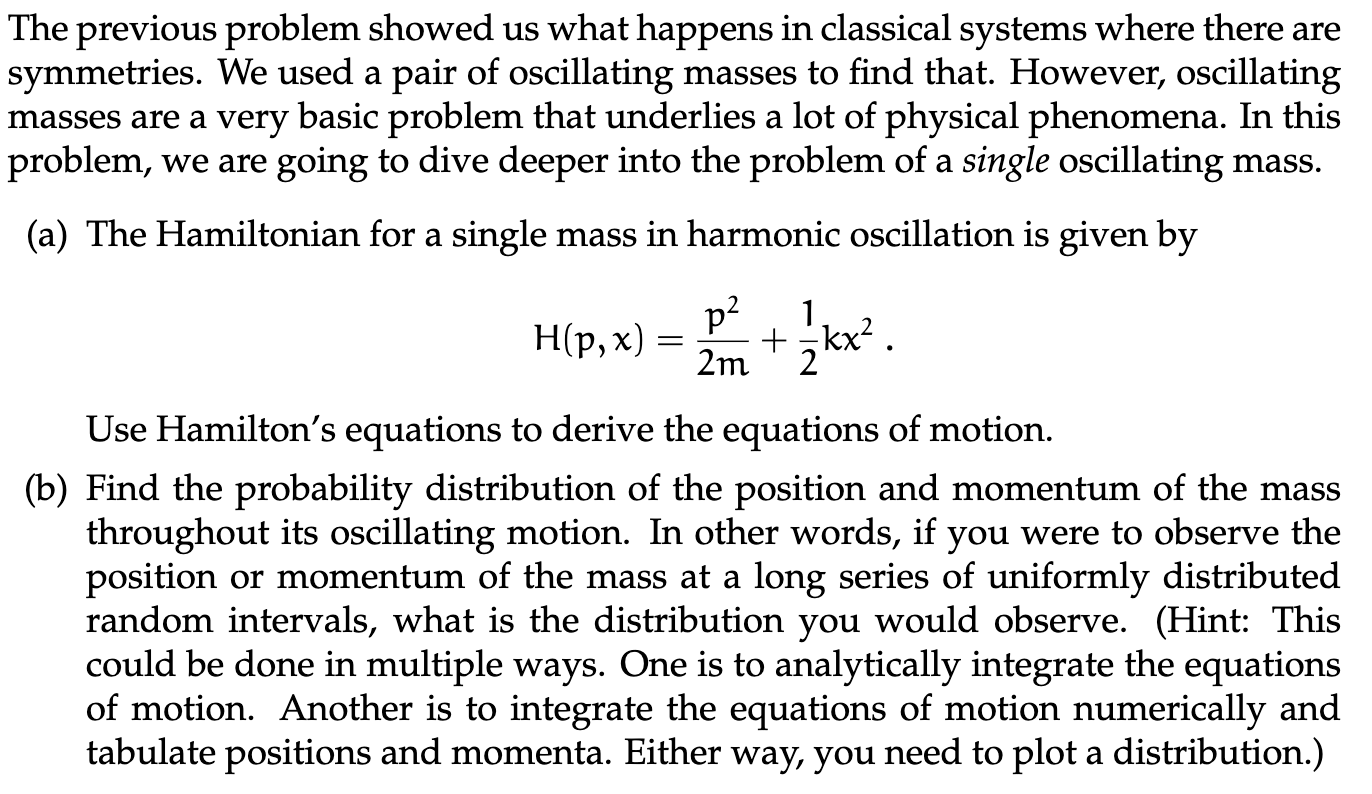
The previous problem showed us what happens in classical systems where there are symmetries. We used a pair of oscillating masses to find that. However, oscillating masses are a very basic problem that underlies a lot of physical phenomena. In this problem, we are going to dive deeper into the problem of a single oscillating mass. (a) The Hamiltonian for a single mass in harmonic oscillation is given by p 1 H(p, x) = + = kx. 2m 2 Use Hamilton's equations to derive the equations of motion. (b) Find the probability distribution of the position and momentum of the mass throughout its oscillating motion. In other words, if you were to observe the position or momentum of the mass at a long series of uniformly distributed random intervals, what is the distribution you would observe. (Hint: This could be done in multiple ways. One is to analytically integrate the equations of motion. Another is to integrate the equations of motion numerically and tabulate positions and momenta. Either way, you need to plot a distribution.)